Gyroscopic Force/Torque of a Constrained Wheel

Question

Description

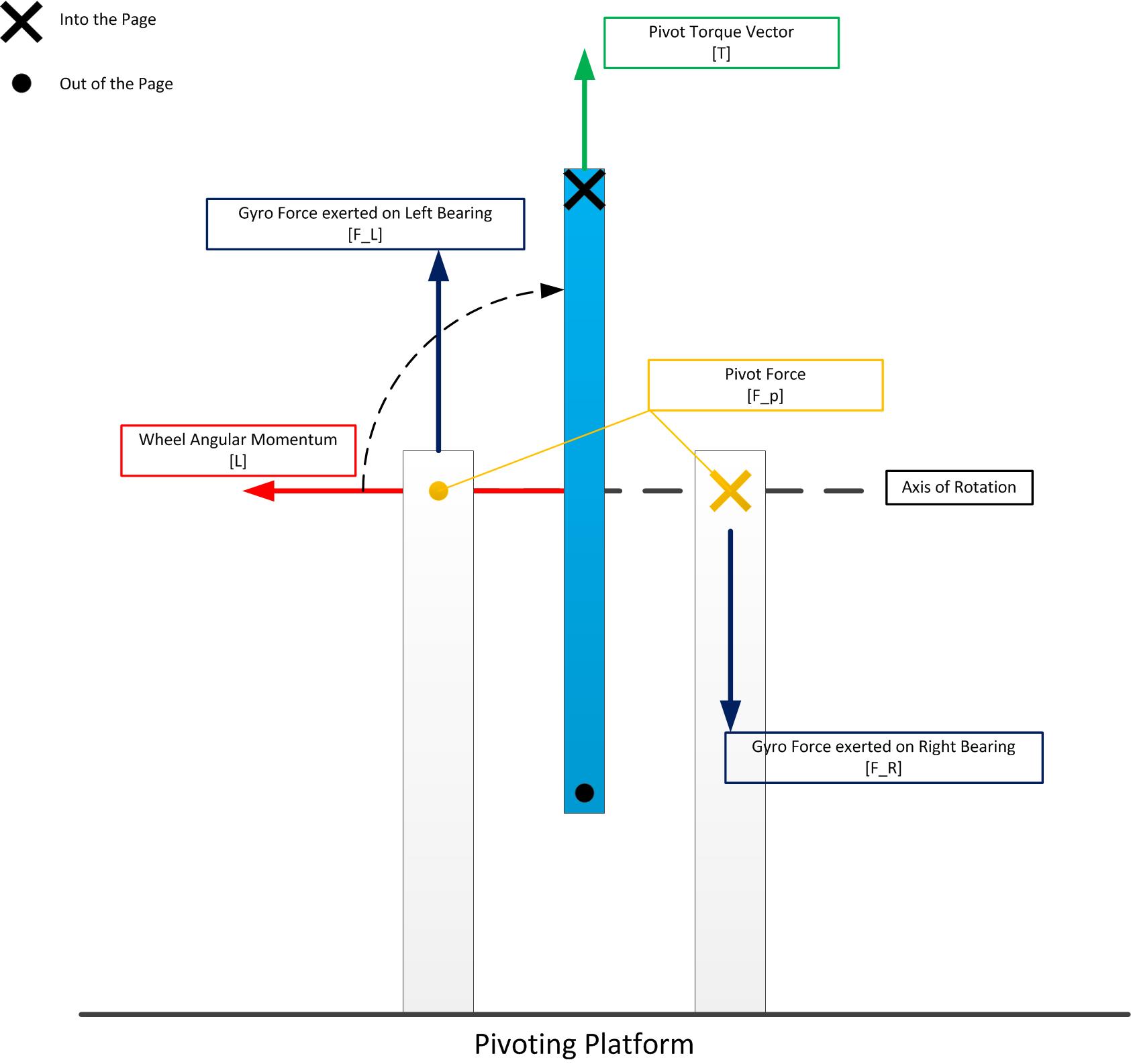
I have a wheel spinning away from me in the vertical plane. The angular momentum is pointing to the left. The axis of rotation is horizontal, and it is supported on either side by a bearing. Think of a free spinning bike wheel. If I then rotate the whole assembly, bearings and wheel, about the vertical axis, i.e. perpendicular to the axis of rotation, it will induce a torque in the vertical direction. Given what I understand about angular momentum and gyroscopes the wheel would want to resist this and instead rotate in the direction that would align it's horizontal angular momentum vector with the new vertical torque vector. Below is an image of what I am talking about.

The dotted arc shows the direction that the wheel would attempt to go. The Gyro Force exerted on the two bearings is what I am looking for. Also the arrows are not to scale and are unrelated to magnitude, they are only there to show direction. If anything is unclear please let me know and I will gladly attempt to clarify.

Question

How do I calculate the force that would be applied to both bearings by the wheel wanting to turn in the vertical plan perpendicular to it's spinning plane?

If it makes the problem easier we can also assume that I know omega_p which is the pivot speed of the whole setup.

I've searched all over the web for the answer to this question and can't seem to find the answer. I keep coming up with the speed that it would precess or direction of precession. I'm looking for the magnitude of the torque/force that the wheel applies to the bearings.

Reference Material

Here is a copy of a PDF that has gotten the closest to explaining what I am looking for but not quite it since the gyro I am talking about is fully constrained. I may have missed something though: http://veemgyro.com/wp-content/uploads/2015/11/White_Paper_1403-How_Gyros_Create_Stabilizing-Torque.pdf

Answer 1

What you have is an articulated system with two bodies. The math is quite complex to solve the general problem. I have done so for you.

Consider the two bearings A and B with gravity acting on the negative z direction and the disk spinning about the x axis. The bearing reactions are

$$ \begin{align} A_y & = \frac{I_{flip}}{\ell} \dot{\Omega} \\ B_y & = -\frac{I_{flip}}{\ell} \dot{\Omega} \\ A_z & = \frac{m_{disk}}{2}g - \frac{I_{spin}}{\ell} \Omega \omega \\ B_z & = \frac{m_{disk}}{2}g + \frac{I_{spin}}{\ell} \Omega \omega \\ \end{align} $$

where the spin speed is $\omega$, the pivot speed $\Omega$ (and their derivatives $\dot{\boxed{}}$), $\ell$ is the separation between the two bearings and $m_{disk}$, $I_{spin}$ and $I_{flip}$ are the inertial properties of the spinning disk.

• Reference: Derivation of Newton-Euler equations of motion

Answer 2

Looking closer at the document that I posted and then checking with my friend who did a littler derivation, the answer was in there all along and it's much easier then I expected.

$$ \tau_{precess} = I * \omega_{wheel} \times \omega_{pivot} $$

Then to get the force on each of the bearings, assuming they are equidistant, you divide the torque by 2 and divide by the radius.

$$ F_{bearing} = \dfrac{\tau}{2*dist_{bearing}}$$

For reference the explanation and then equations can be found on actual page 4 and 5 of the document I linked in the question. It should be steps 1-3a.

If you are curious the rest of that document does a great job at explaining how gyros stabilize vessels.