4
$\begingroup$

Two bodies in space always orbit their center of mass. So the relative motion of the Sun and the Earth happen in the same line, save for the rotation of the Sun. So, how do we measure

  • The time taken for the Earth to orbit the barycenter

  • The time taken for the Earth to orbit the Sun (The solar rotation period is 28 days while the Earth's period of revolution is 365 days, so does that mean the earth can never revolve around the Sun, considering the Earth is revolving around the barycenter, and not around a stationary Sun? Or do we calculate the time of revolution in the opposite direction? If I place a camera on the surface of the Sun, will I see the earth completing a revolution only in the opposite direction? If that is not the definition of a orbital revolution, what else is?)

I'm aware of the sidereal and tropical years for measuring the calendar year, I'm just concerned about the concept of orbital revolution.

Is the concept of revolution even properly defined compared to the conventional definition if we consider that we orbit the barycenter and not the center of the Sun?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
4
$\begingroup$

In a simplified two-body orbit, both objects orbit their common barycentre with the same orbital period. The orbital period can be estimated as (i) the time between two closest approaches (since in general there is some eccentricity in elliptical orbits), or (ii) the time between when a projected line between the two bodies is at a defined position on the celestial sphere (with a coordinate system defined by very distant astronomical objects, e.g. quasars).

The Earth does not "orbit the Sun", except in an approximate sense, but there would be nothing to stop you considering motion in the heliocentric frame and defining the orbital period of the Earth in the same two ways as above.

Improve this answer
$\endgroup$
6
  • $\begingroup$ The second point makes sense. Regarding the first point, isn't the eccentricity arising from the fact that the center of mass is not at the center of the line joining the two bodies? i.e. When considering the orbit of Earth around the center of the Sun, one of the two foci of the elliptical orbit is at their barycenter and the other is a moving foci through space. If we considered the orbits of each body about their barycenter itself, wouldn't it just form two concentric circles, with the Sun having the smaller circle? $\endgroup$
    – Jyothish Kumar
    Commented Sep 18, 2023 at 13:38
  • $\begingroup$ My point was based on the animations shown here. But then I saw that the binary star system also has a more eccentric orbit than the Pluto-Charon system in the animations. It also seems that the solution to the two-body problem is an elliptic (or other conic sections) with one focus at the barycenter. i.e. not just the relative orbits, but also the orbits about the barycenter are elliptic. Why are the orbits of each body around the barycenters not perfectly circular? (Although this may be better suited for a separate question) $\endgroup$
    – Jyothish Kumar
    Commented Sep 18, 2023 at 13:39
  • $\begingroup$ For example, if I rotate a pencil about its center (assuming the ends being two bodies of equal mass), both bodies orbit the center in circular orbits. If I instead rotate it about a point not at the center, both ends still rotate in circular orbits about the center of rotation, but the relative motion between the two bodies would be an elliptical orbit with one focus at the point of rotation and another a moving foci through space, near the lighter end. I'm not even sure if this can be called an elliptical orbit since the heavier body is also rotating. $\endgroup$
    – Jyothish Kumar
    Commented Sep 18, 2023 at 13:44
  • $\begingroup$ In the pencil example, since both bodies are orbiting their barycenter (center of rotation), it doesn't seem like the lighter body rotates around the larger body in any sense, aside from if we consider the rotation of the heavier body. i.e. As I said in the question, if I place a camera on the heavy end of the pencil, it won't see the lighter end orbiting around it, unless it was rotating. $\endgroup$
    – Jyothish Kumar
    Commented Sep 18, 2023 at 13:49
  • 1
    $\begingroup$ "Regarding the first point, isn't the eccentricity arising from the fact that the center of mass is not at the center of the line joining the two bodies?": No. Elliptical orbits can have any eccentricity, regardless of the mass ratio of the two components. $\endgroup$
    – ProfRob
    Commented Sep 18, 2023 at 15:09
2
$\begingroup$

The solar rotation is (mostly) irrelevant to the Earth's orbital period. (Incidentally, the Sun doesn't rotate like a solid body. Different latitudes rotate at different periods. See Solar rotation).

Yes, the Earth and Sun orbit about their common barycentre. However, the Sun / Earth mass ratio is huge (~329,000), so the distance of the centre of the Sun from the Sun-Earth barycentre is relatively small, ~455 km.

A more important factor is that the Earth and Moon orbit their common barycentre. That is, the Earth and Moon orbit the Earth-Moon barycentre (EMB), and the EMB orbits the Sun-EMB barycentre.

The Earth/Moon mass ratio is ~81.3. The mean Earth-Moon distance is ~385,000 km, and the mean distance of the centre of the Earth to the EMB is ~4,678 km, more than ten times greater than the Sun-EMB barycentre distance.

The actual Earth-EMB distance varies over the course of a month, and over the course of a year, mostly due to the eccentricity of the Earth-Moon orbit and of the EMB-Sun orbit. Here's a plot of the Earth-EMB distance, using data from NASA JPL Horizons:

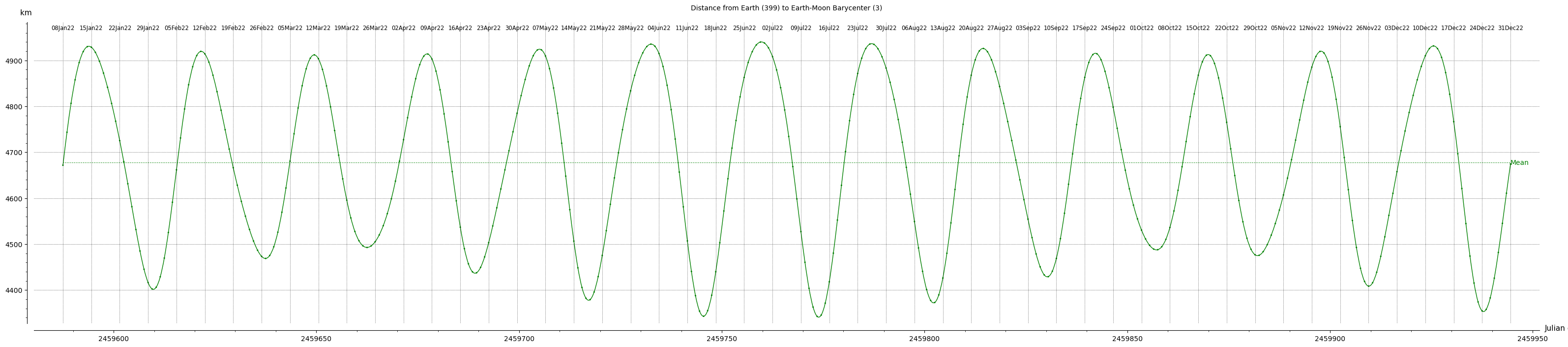
Earth-EMB distance

However, the effects of these barycentres on the Earth's orbital speed is relatively small. The Earth's mean orbital speed is ~29,780 m/s. The speed of the Earth's orbit around the EMB is only 12.4 m/s. And the speed of the Sun around the Sun-EMB barycentre is only 0.09 m/s.

The mean orbital radius of the EMB is ~149.58 million km, so the motion of the Earth relative to the EMB is rather small, but it's important when doing precision analysis of the Earth's orbital motion.

Here's a Horizons plot of the speed of the EMB relative to the Sun, using a 1 day timestep.

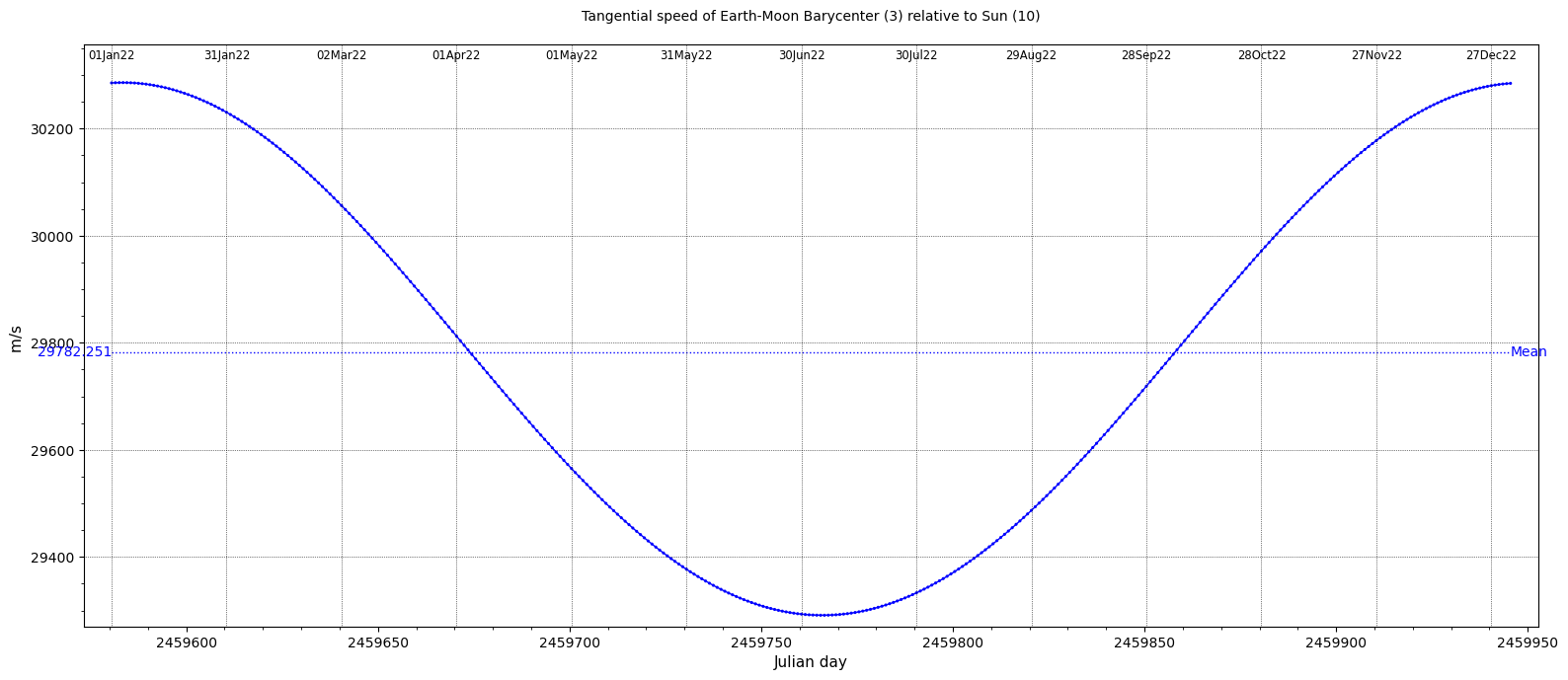
EMB-Sun speed graph

Here's the corresponding plot of the Earth's speed relative to the Sun. We can clearly see the effect of the Moon's perturbation.

Earth-Sun speed graph

In the question, you mention the sidereal and tropical years. Another important year is the anomalistic year, which is usually defined as the time between perihelion passages. The mean anomalistic year of the EMB is ~365.2596358 days. See Wikipedia's Sidereal, tropical, and anomalistic years for further details.

Our calendar uses the tropical year because we want the calendar year to stay synchronised with the seasons. But if you want a year that tracks when the EMB is closest to the Sun, then you need the anomalistic year.

The motion of the EMB is slightly perturbed by the other planets, primarily Venus (because it's close) and Jupiter (because it's massive). The plane of the orbit (the ecliptic) is quite stable, but the line from the perihelion to the aphelion slowly precesses.

Traditionally, motions in the Solar System used a frame defined by the ecliptic and the Earth's equatorial plane (i.e., the plane of the Earth's rotation on its axis). The intersection of these planes determines the equinox direction. However, the Earth's axis also precesses slowly. Modern celestial mechanics uses the International Celestial Reference Frame, which is based on a set of distant extragalactic objects, although it's aligned to the equatorial frame of the year 2000.

I have further information on the Earth / EMB motion here: https://astronomy.stackexchange.com/a/49546/16685 and on the topic of perihelion and the equinoxes & solstices here: https://astronomy.stackexchange.com/a/49605/16685

You may also be interested in the motion of the Sun relative to the Solar System barycentre (SSB): https://astronomy.stackexchange.com/a/28036/16685

Horizons data comes from the Jet Propulsion Laboratory Development Ephemeris. For further details, please see The JPL Planetary and Lunar Ephemerides DE440 and DE441, Park et al (2021).

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.