21
$\begingroup$

Long story short, my brother made a joke about how stupid it is to celebrate the Earth making one "trip" around the Sun: New Year's Eve.

So I got curious and was wondering: how could the first physicists guess that a revolution of the Earth around the Sun takes 365.25xxx days?

I've tried my luck on the internet but didn't find anything simple enough to understand. I've read about Kepler's law, and there are nice formulas, but how does he work this out? And how could the first people who knew that Earth was revolving around the Sun guess how much time an entire revolution took?

I asked my brother and he told me if he'd have to do it, he would simply watch the stars and map them, do that every night, and count the days until he came back to the exact same position of the first night. Is that realistic?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ There are quite a few different ways to define a year, see en.wikipedia.org/wiki/Year#Astronomical_years $\endgroup$
    – PM 2Ring
    Commented Nov 29, 2019 at 18:48
  • $\begingroup$ I've removed some comments that should have been posted as answers, and debates about their correctness. Please use comments to suggest improvements to the question, and use answers to answer the question. $\endgroup$
    – rob ♦
    Commented Nov 29, 2019 at 19:26

7 Answers 7

Reset to default
30
$\begingroup$

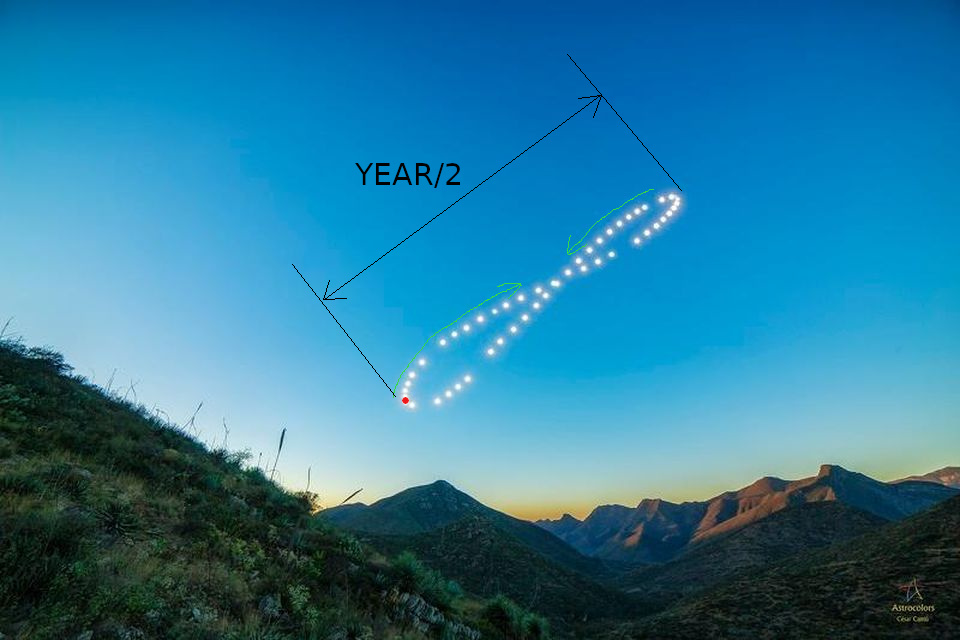
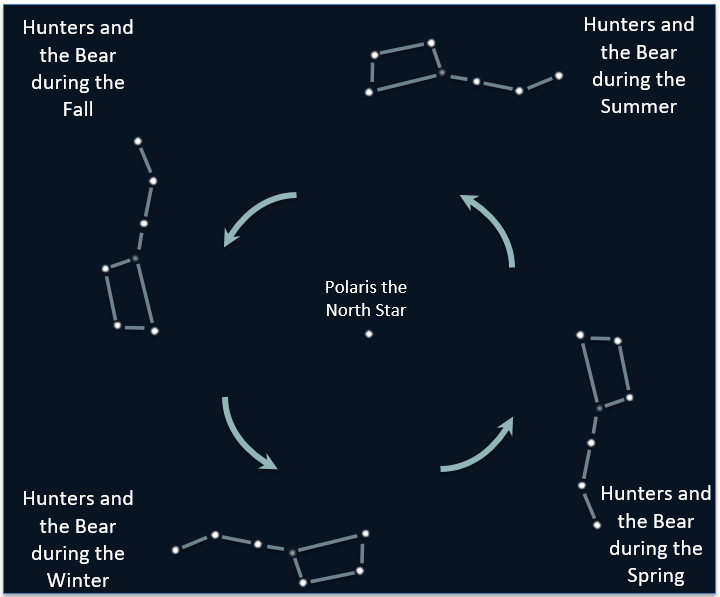
In ancient civilizations, astronomy was a serious business (among other reasons, to accurately predict the seasons), so there were a lot of scientists making very careful measurements. Even with the naked eye, you can make quite accurate observations, and the ancients used these observations well.

The first really accurate determination of the length of the year was made by Hipparchus, a Greek astronomer who lived circa 190-120 BC. He calculated a year to be 365 + 1/4 - 1/300 (365.24667) days long, which is 6 minutes/year longer than the current estimate. Before that time, most people used 365.25, which is also not too far off (11 minutes), but is likely also due to the lucky coincidence the actual value is so near the neat round 1/4 day.

Hipparchus mostly used exact measuring of the equinoxes (the moment day and night are just as long, and when the Sun rises exactly in the east and sets exactly in the west). It's possible to measure this quite exactly, up to an hour. But Hipparchus also used the data gathered by earlier astronomers, back until 432 BC (~300 years before him). When you have this large time-span, and combine multiple measurements to cancel out any errors, it's thus possible to get very accurate results. Oh, and he used solar eclipses to refine his measurements as well, though the equinoxes were more important (there are more of them).

Incidentally, he was also the first one to discredit your question ;-)

That is, what most people think of as "a year", is actually not the time it takes the Earth to complete one orbit around the Sun. It's very close, but the Earth also precesses: it's top (the poles) very slightly turn around. It also means that Polaris (our current "northern pole star") will not remain the northern pole star forever (but it will for several thousand years). This also means that the time from equinox to equinox is not exactly the same as the time it takes the Earth to complete an orbit, it is shorter by 20 minutes and 24.5 seconds.

The time it takes the Earth to complete an orbit is called a sidereal year, but the time it takes for the equinoxes to repeat (and thus the seasons), is called a tropical year. Strictly speaking, "a year" could refer to both of them, but most of the time a tropical year is meant. And a tropical year is also what our calendar is based on.

At least, the western calendar uses a value of 365.2425 days, while the modern established value for a tropical year is 365.24219 days (27 seconds shorter), and a sidereal year is 365.256 36 days (1197.5 seconds longer) These values are all assuming a day is exactly 86400 SI seconds, without any leap seconds.

Improve this answer
$\endgroup$
5
  • 4
    $\begingroup$ Can you elaborate on how precession changes how long it takes the Earth to complete an orbit around the sun? I am having a hard time seeing how a wobbly axis could either change the length of a day or the speed at which the Earth orbits the sun - and 20+ minutes/year seems like a big difference. $\endgroup$
    – Michael
    Commented Nov 29, 2019 at 21:04
  • $\begingroup$ Great explanation I loved it ! out of curiosity, did Hipparchus know that Earth was actually going around the sun, and not the opposite ? $\endgroup$
    – Loïc
    Commented Nov 30, 2019 at 1:00
  • 1
    $\begingroup$ @Michael Precession affects equinoxes (tropical year) as the exact point that the Earth's axis is perpendicular with the line to the sun slowly changes over time. As you say, the sidereal year is unaffected. $\endgroup$
    – jaxad0127
    Commented Nov 30, 2019 at 8:56
  • 1
    $\begingroup$ @Loïc Geocentric vs Heliocentric doesn't matter here. Equinox to equinox is the same either way. $\endgroup$
    – jaxad0127
    Commented Nov 30, 2019 at 8:57
  • 1
    $\begingroup$ @Loic I don't know if he was a proponant of heliocentrism or geocentrism (and as jaxadl0127 pointed out, it makes no difference here). The only thing I can find is that the idea of heliocentrism was introduced, but not widely accepted at his time. $\endgroup$
    – Emil Bode
    Commented Nov 30, 2019 at 11:31
29
$\begingroup$

Observe where the Sun rises and sets, and how high it gets in the sky at midday. These things repeat after 365 days.

Improve this answer
$\endgroup$
14
  • 8
    $\begingroup$ Yes, but isn’t it easier to notice the big bright thing? $\endgroup$
    – G. Smith
    Commented Nov 28, 2019 at 18:19
  • 12
    $\begingroup$ Early civilizations figured this out long before there was anything called physics. They needed to understand things like this to know when to plant crops. $\endgroup$
    – G. Smith
    Commented Nov 28, 2019 at 18:23
  • 2
    $\begingroup$ @G.Smith the early civilizations didn't know that the Earth goes around the Sun, though. They simply knew that 365 days is the duration of the cycle of seasons, which could be checked by observations of the Sun. $\endgroup$
    – IMil
    Commented Nov 29, 2019 at 4:23
  • 2
    $\begingroup$ This doesn't work in the equator, does it? $\endgroup$
    – justhalf
    Commented Nov 29, 2019 at 5:17
  • 2
    $\begingroup$ 365 is fine, but I'm really curious regarding the precision of experiments (conducted by ancient men, this way) to infer about the remaining 6 hours and a few minutes, especially if it takes ancient people a few minutes itself in recording/jotting their "observations". e.g. using this method, how precisely can you say that 1 orbit is 365 days, 365 days & 5 hours, versus 365 days & 6 hours, 365 days, 6 hours & 5 minutes ... Also, the Sun is extended, so would there be a discernable amount of difference between the last two, which ancient men could be sure of? (Notice that OP says 365.25xx d). $\endgroup$
    – 299792458
    Commented Nov 29, 2019 at 8:02
16
$\begingroup$

If you would capture sun position in the sky at the same time each day of year - you would get a periodic figure, called Analemma which shows sun's path in a sky through a whole year :

enter image description here

Improve this answer
$\endgroup$
10
  • 1
    $\begingroup$ @usernumber I believe he means the position along the horizon where the sun rises and sets. As viewed from a consistent location on earth's surface, changes over the course of the year, but once you go a full year, it'll be back where you started and the oscillation will begin again. $\endgroup$
    – Ruadhan2300
    Commented Nov 29, 2019 at 10:47
  • 2
    $\begingroup$ This raises the additional question, how could the first physicists measure "the same time each day". $\endgroup$
    – Federico Poloni
    Commented Nov 29, 2019 at 21:21
  • 1
    $\begingroup$ @FedericoPoloni Yes, you need decent mechanical clocks whose accuracy is better than the planet's rotation from day to day, and a well-measured mean solar day to know when to record the position of the sun to make an analemma. $\endgroup$
    – notovny
    Commented Nov 30, 2019 at 11:15
  • 3
    $\begingroup$ @FedericoPoloni The simplest way to measure the same time each day is to track noon, which you can do just by looking at the sun. In essence, you can just stick a pole in the ground, and every day mark the tip of the shadow when the sun is at its highest, and doing it for 365 days will get you a drawing of the analemma. $\endgroup$
    – Peteris
    Commented Nov 30, 2019 at 18:55
  • 2
    $\begingroup$ @Peteris no, you won't. Solar highest altitude doesn't always happen at noon but it always happen when the sun crosses the meridian. You wouldn't get an "8" shaped analemma, you'd just get an "I" shape. See "equation of time". $\endgroup$
    – Eric Duminil
    Commented Dec 2, 2019 at 3:37
9
$\begingroup$

I asked my friend, and he told me, if he'd have to do it, he would simply watch at the stars, and map them, and do that until he came back to the exact same position of the first night. Is that realistic ? I don't think the position would differ so much that the eye could see it. Am I right ?

No light pollution

Please remember there was no light pollution at that time. It was possible to see more stars than today, they appeared brighter and more colorful. The Milky Way, Andromeda Galaxy and Orion Nebula were perfectly visible to the naked eye.

Seasonal constellations

There are dozens of easy indicators in the night sky if you want to know the season:

With this information alone, it is possible to reliably tell the current month by simply looking at the night sky after sunset, without any instrument.

Accuracy could be improved by using a very bright star (e.g. Sirius) and clear reference points (e.g. a far-away mountain).

Guardians of the pole star

You could use Ursa Minor and Ursa Major as an annual clock, with the two guardians rotating around Polaris. They do a full turn in a year, so an accuracy of 1° will give you an accuracy of ~1 day. It shouldn't be hard to measure with an astrolabe.

enter image description here

Improving accuracy

You cannot expect high accuracy if you measure the thickness of a single sheet of paper with a ruler. You can improve it greatly if you stack 1000 sheets, measure the total height and divide it by 1000.

Patterns in star position will repeat after approximately 365 days, but they will repeat almost exactly after 4 revolutions in 1461 days, which gives $\frac{1461}{4} = 365.25$. With enough time and dedication, it's possible to improve the accuracy even further.

Improve this answer
$\endgroup$
1
  • 3
    $\begingroup$ The answer for "How did early physicists...?" is very often "by looking at stars". $\endgroup$
    – JiK
    Commented Nov 29, 2019 at 15:44
2
$\begingroup$

In order to find out how the topic evolved in the past 1000's of year, the proper search on the Internet is about the different calendar systems that people employed in the past.

Generally, calendars evolved between better and better precision and different attempts to fit the seasons and the moon phases around some easy calculatable numbers (12, 30, 360, etc...).

It was late in the process when people found out about the movement of the celestial bodies.

Improve this answer
$\endgroup$
2
$\begingroup$

It is not that simple. It takes tools such as Stonehenge to accurately time the passage of stars. You may then notice that the Sun's passage across the sky varies with a period of 365.25 days.

Improve this answer
$\endgroup$
3
  • 4
    $\begingroup$ I'm pretty sure to measure the length of the year, only two fixed points are necessary. So take a tree, stand where the sunrise and the tree are aligned, place a stone where you stand. Check again next year. $\endgroup$
    – Christian
    Commented Nov 29, 2019 at 14:54
  • 3
    $\begingroup$ @Christian Place a big, stable stone circle, such as Stonehenge, and keep people out. Put some scientist or priest in charge, preferable with guards. A tree is not a very good plan. It grows, changes shape, dies, is cut down, burns. Other trees block the view. Etc. $\endgroup$
    – my2cts
    Commented Nov 29, 2019 at 17:13
  • 2
    $\begingroup$ but building stonehege is hard. finding trees is easy. Depends on the precision you need. $\endgroup$
    – Christian
    Commented Nov 29, 2019 at 17:49
-2
$\begingroup$

It is a lot simpler. In Europe we have four seasons. The same season returns about 365 days later. Over time the measurement got more and more exact.

The reason we have seasons is that the earth axis is slightly inclined compared to the movement around the sun.

Improve this answer
$\endgroup$
6
  • 5
    $\begingroup$ I'm not so sure about that. There are so many fluctuations from one year to the other that I don't think it would be possible to time seasons more accurately than 1 or 2 weeks using weather alone. $\endgroup$
    – Eric Duminil
    Commented Nov 29, 2019 at 10:18
  • 2
    $\begingroup$ i would call the inclination a bit more than "slight", it's over 20 degrees! $\endgroup$
    – Michael
    Commented Nov 29, 2019 at 21:12
  • 1
    $\begingroup$ @EricDuminil If you know that, say, July 1st, 1919 was a summer day, and you know the count of days to July 1st, 2019, which falls into the 100th summer after that, you have already fixed the length of the year with a 0.25% accuracy, which is less than a day. You can get to your 2 weeks precision with an observation period of only 13 years using weather alone. $\endgroup$
    – cmaster - reinstate monica
    Commented Nov 29, 2019 at 23:48
  • $\begingroup$ @notovny see cmaster comment above. $\endgroup$
    – Eric Duminil
    Commented Dec 1, 2019 at 14:39
  • 1
    $\begingroup$ @notovny My point was: Take any day that feels like summer in 1919 and 2019, and you'll get your 0.25% accuracy for the length of the year. The first of July was just an example. You can greatly improve this method by taking an event that typically occurs within a shorter time frame than three month. The first frost could be a good one for my location: It's usually within the same 2 weeks time frame. By observing stars/sun, you basically do the same thing, using basic events with less variance. But you can get arbitrary precise with horribly variant events by using a long enough time span. $\endgroup$
    – cmaster - reinstate monica
    Commented Dec 1, 2019 at 22:25

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.