Are linear and angular kinetic energies separate from each other?

Question

Suppose an object was rolling (moving both rotationally and translationally):

• Would the object's total kinetic energy be the sum of both linear and angular kinetic energies? i.e. $K_{net}=\frac{1}{2}mv^2+\frac{1}{2}I\omega^2$?
• OR should linear and angular kinetic energies be treated as separate entities, similar to how linear and angular momentum are completely separate?

Thank you so much!

Answer 1

Short answer. These contributions can be identified in the kinetic energy of a rigid system, whose material points move under the rigid-body constraint

$\mathbf{v}_P - \mathbf{v}_Q = \omega \times (\mathbf{r}_P - \mathbf{r}_Q)$,

for every material points $P$, $Q$ of the system.

Kinetic energy as an additive physical quantity. For every physical system, the kinetic energy is an additive quantity, i.e. the kinetic eneergy of the system is equal to the sum of the kinetic energy of its parts: you take the parts of the system, you evaluate the kinetic energy of each part and sum these terms and you get the kinetic energy of the overall system.

For a system with a discrete point mass distribution, we can write it as

$K = \sum_i K_i = \sum_i \dfrac{1}{2} m_i |\mathbf{v}_i|^2 = \sum_i \dfrac{1}{2} m_i \mathbf{v}_i \cdot \mathbf{v}_i $,

or for a system with continuous mass distribution, with density $\rho(\mathbf{x})$, we can write it as

$K = \dfrac{1}{2} \displaystyle \int_{\Omega} \rho(x) |\mathbf{v}(\mathbf{x})|^2$

Kinetic energy for a rigid system. (here, only for discrete systems; as an exercise try to retrieve the same expressions for continuous systems). Using the rigid-body constraint, it's possible to write the velocity $\mathbf{v}_i$ of each point mass of the system w.r.t the center of mass of the system, $\mathbf{r}_G$,

$\mathbf{v}_i = \mathbf{v}_G + \omega \times (\mathbf{r}_i - \mathbf{r}_G)$,

where the position and the velocity of the center of mass are $\mathbf{r}_G = \dfrac{\sum_i m_i \mathbf{r}_i}{\sum_i m_i}$, $\mathbf{v}_G = \dfrac{\sum_i m_i \mathbf{v}_i}{\sum_i m_i}$.

Introducing the expression for $\mathbf{v}_i$ in the expression for the kinetic energy, we get

$K = \sum_i \dfrac{1}{2} m_i \mathbf{v}_i \cdot \mathbf{v}_i = \sum_i \dfrac{1}{2} m_i (\mathbf{v}_G + \omega \times (\mathbf{r}_i - \mathbf{r}_G)) \cdot (\mathbf{v}_G + \omega \times (\mathbf{r}_i - \mathbf{r}_G)) $,

and rearranging the terms

$K = \dfrac{1}{2} \sum_i m_i |\mathbf{v}_G|^2 + \mathbf{v}_G \cdot \omega \times \underbrace{\sum_i m_i (\mathbf{r}_i - \mathbf{r}_G)}_{=\mathbf{0} \text{ (def of G)}} + \dfrac{1}{2} \sum_i \underbrace{ m_i(\omega \times (\mathbf{r}_i - \mathbf{r}_G)) \cdot (\omega \times (\mathbf{r}_i - \mathbf{r}_G))}_{= - m_i \omega \cdot (\mathbf{r}_i - \mathbf{r}_G) \times ((\mathbf{r}_i - \mathbf{r}_G) \times \omega)} $.

Now, we can sum over $i$, to recognize:

• the total mass of the system $m = \sum_i m_i$
• the inertia tensor of the system w.r.t. its center of mass $G$, $\mathbb{I}_G = - \sum_i m_i (\mathbf{r}_i - \mathbf{r}_G) \times (\mathbf{r}_i - \mathbf{r}_G) \times$

and eventually write the kinetic energy for a rigid system as the sum of the contribution of the translation of its center of mass and the rotation around it,

$K = \dfrac{1}{2} m |\mathbf{v}_G|^2 + \dfrac{1}{2} \omega \cdot \mathbb{I}_G \cdot \omega$.

Answer 2

Let me first discuss the relation between linear momentum and angular momentum.

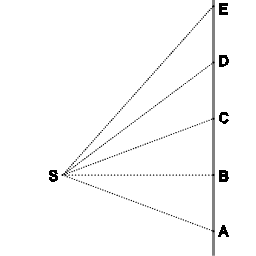
Diagram 1. Area property of uniform linear motion

'S' represents a stationary point. Put differently: let point S be in inertial motion, and let the inertial coordinate that is used be the one that is co-moving with point S.

Let an object be in inertial motion. The rectilinear motion brings it along the points A, B, C, D, E, ...

Inertial motion has the following property: in equal intervals of time equal distances of space are traversed.

So: the lengths of the intervals AB, BC, etc. are all the same.

It follows geometrically that the areas of the triangles SAB, SBC, etc. are all the same.

In Isaac Newoton's work the Principia the first theorem presented is a geometric demonstration that Kepler's law of areas follows from first principles.

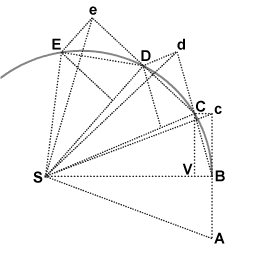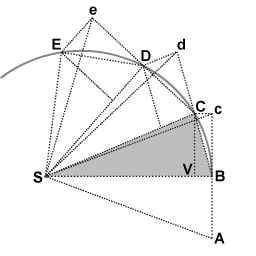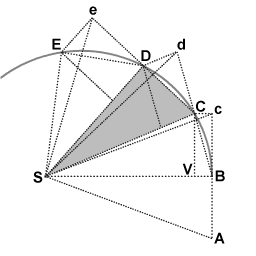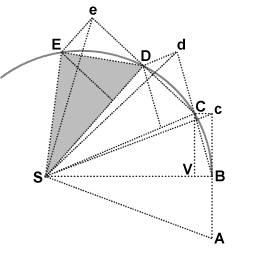
Diagram 2. Newton's demonstration of Kepler's area law

(For explanation of the diagram: stackexchange answer about angular momentum, )

As we know: kepler's law of areas was a precursor to the concept of conservation of angular momentum.

For the case of circular motion: Let the circumnavigating velocity be $v_c$ and let the radius of circular motion be $r$. With $\omega$ for angular velocity:

We have the general relation between velocity along circular motion, and angular velocity:

$$ v_c = \omega \cdot r \tag{1} $$

The area of a triangle is proportional to the product of the base and the height. In diagram 2 the length of the base is the radial distance $r$ and the height of the triangle is proportional to the velocity component perpendicular to the radial line.

That is, in the context of newtonian mechanics, the connectin between linear momentum and angular momentum.

Energy

The great advantage of the concept of kinetic energy is that it is proportional to the square of the velocity.

In many cases it is necessary to decompose velocity in perpendicular components, which is decomposition according to Pythagoras' theorem. Kinetic energy slots in with that, as kinetic energy is proportional to the square of velocity.

For the case of circular motion the kinetic energy of the circumnavigating object is interconverted between linear form and angular vorm according to (1)

Linear kinetic energy: $\tfrac{1}{2}m {v_c}^2$

Angular kinetic energy: $\tfrac{1}{2}m r^2 \omega^2$

When the circumnavigating motion is not circular motion you have to decompose the velocity in radial component and perpendicular-to-radial component. For the angular kinetic energy only the perpendicular-to-radial component of the velocity is used.

Answer 3

Regarding the second bullet, it is correct that linear (translational) and angular (rotational) kinetic energies are separate and distinct components of kinetic energy, like linear and angular momentum are separate and distinct components of momentum. But there is an important difference.

Linear and angular momentum are conserved quantities. Kinetic energy is not a conserved quantity. It is the sum of the mechanical kinetic and potential energies that is conserved for an isolated system with no dissipative internal forces (e.g., friction) involved, or

$(KE)_{tot}+(PE)_{tot}=constant$

And

$\Delta (KE)_{tot}+\Delta (PE)_{tot}=0$

For example, if your ball was undergoing pure rolling down an incline plane of height $h$ starting from rest, then at the bottom of the incline the total change in KE plus the total change in gravitational PE (neglecting any air resistance) will be zero, or

$$\frac{1}{2}mv^2+\frac{1}{2}I\omega^2-mgh=0$$

To sum it up, while the first bullet is correct, the analogy of the second bullet is only partially correct,

Hope this helps.