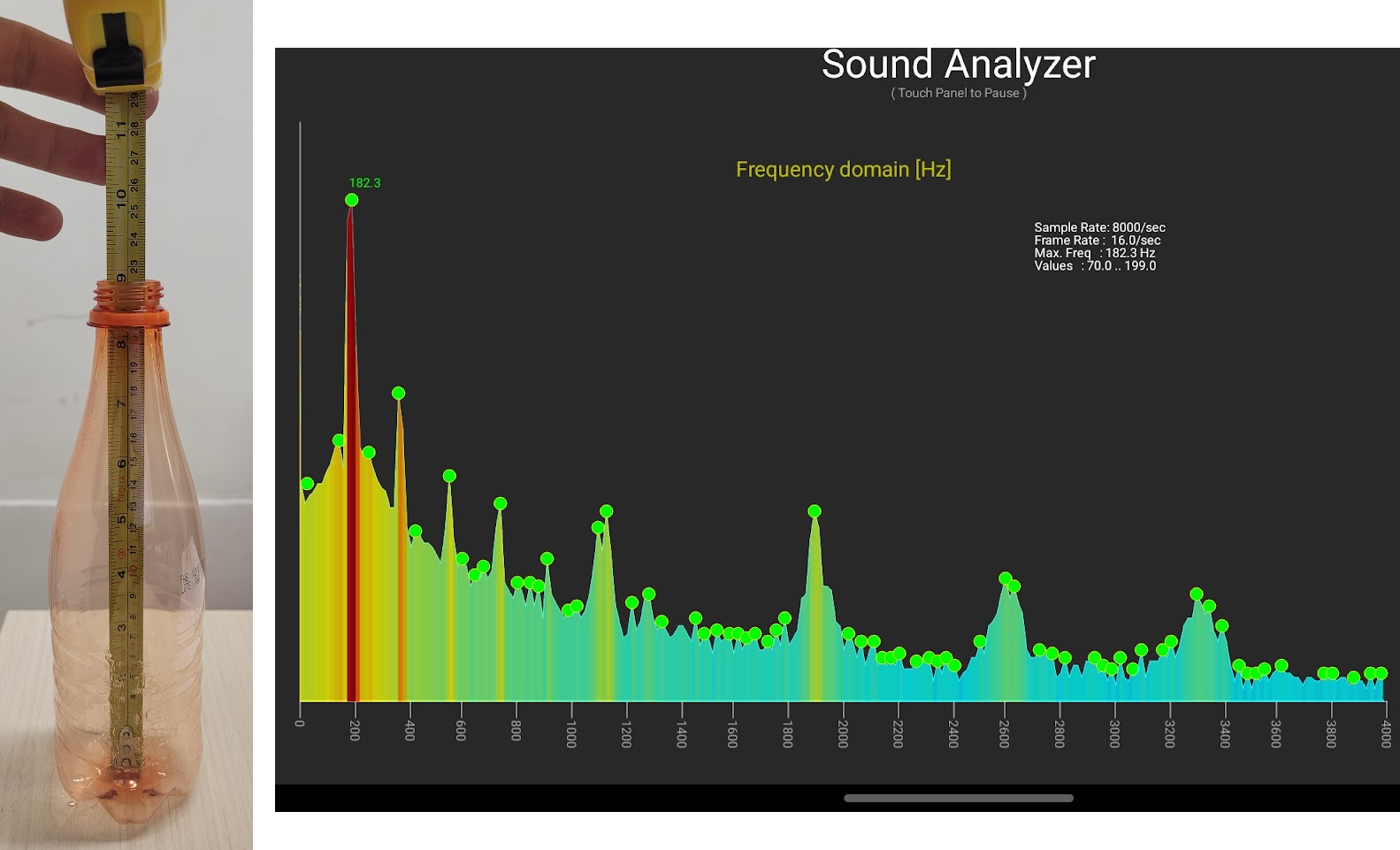
Preparing one lecture about resonance in cylindrical tubes with one closed end, I decided to make some experiments to compare calculated frequencies and measured ones.
We can find anwhere (click here, for example) that the frequencies in such cylindrical tubes match the following formula: $$ f_n = n \cdot \frac{c}{4L}, $$ where $n = 1, 3, 5, 7, ...$, $c \approx 330~\mathrm{m/s}$ is the speed of sound in air, and $L$ is the length of the tube. For one experiment, I used a bottle with $L = 22.5~\mathrm{cm}$ (see the figure below), so that the first resonant frequency is expected to be $f_1 = 367~\mathrm{Hz}$. However, I used a mobile app called Sound Analyzer (click here to install) and measured half of that value. I repeated this test for different bottles, always finding the same discrepancy of a factor of half.
Searching in the internet, I found some pages and articles about Helmholtz resonance, as can be found here: Frequency of the sound when blowing in a bottle.
Every page or paper about Helmholtz resonance present the formula for the frequency: $$ f = \frac{c}{2\pi} \sqrt{\frac{A}{LV}}, $$ where $L$ is the length of the neck of the tube, $A$ is the area of the aperture, and $V$ is the volume of the resonant cavity.
Using Helmholtz formula, therefore, I measured the neck to be $L = 9~\mathrm{cm}$, $A = 3,80~\mathrm{cm^2}$, and $V = 450~\mathrm{mL}$, so that $f \approx 161~\mathrm{Hz}$, much closer than the first calculation.
But I belive that the bottle is quite close to a cylinder, so that manipulating the Helmholtz formula, approaching the generalized, I made $V = AL$, so that the final formula turns to $$ f = \frac{c}{2\pi L}, $$ which is somwhat different from the first equation at the beginning of this question.
My question is, why I always measure half of the calculated frequency for the first resonance mode of one closed end cylinder, no matter the bottle I try (as long as it is a bottle with behaved shape similar to the one in the picture)?