1
$\begingroup$

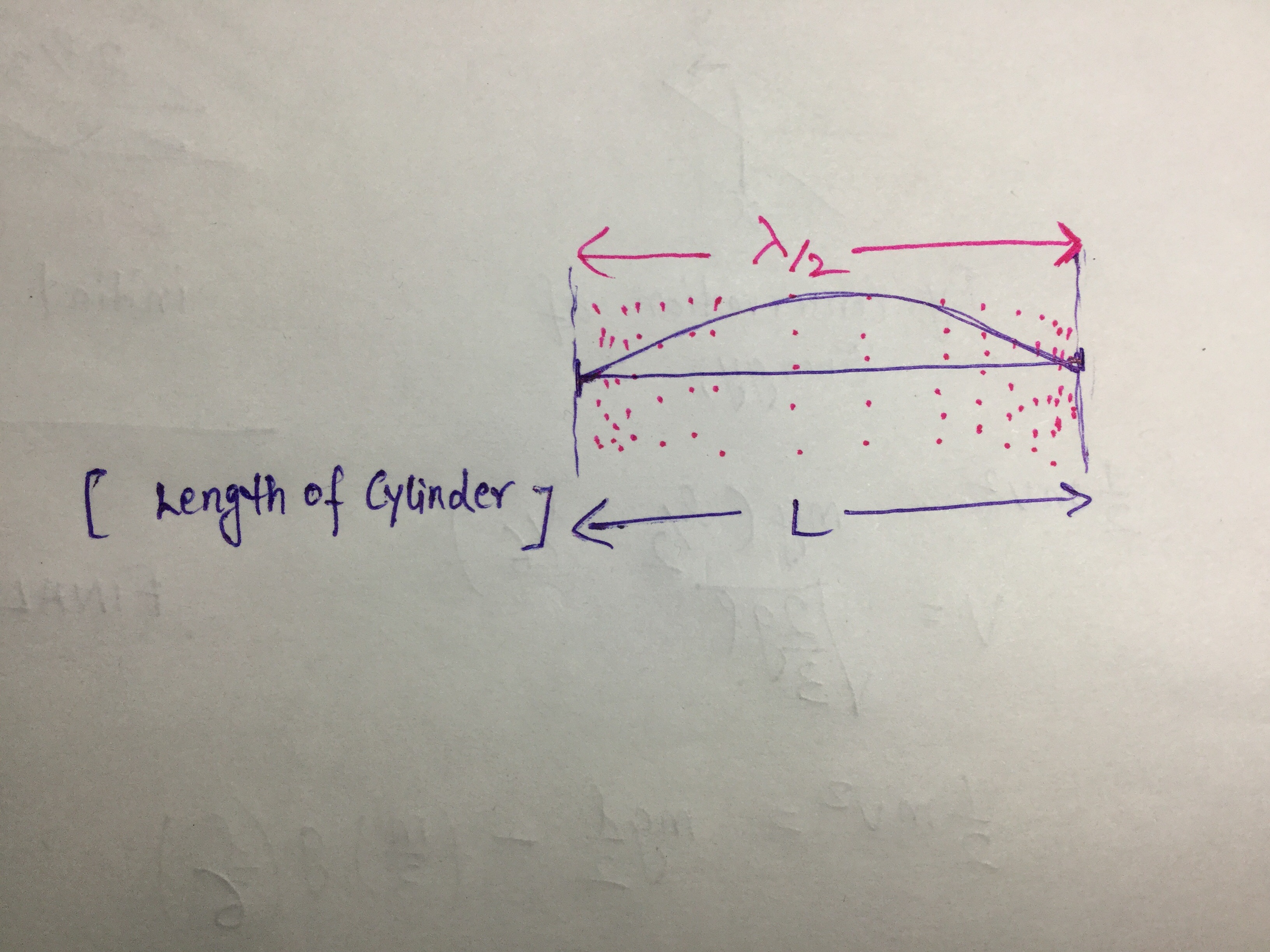
I want to calculate the resonant frequencies of a closed cylinder covered with rigid walls. The cylinder is of dimensions: diameter = $1m$, length = $1m$. Would this fundamental mode formula be correct to use in this case:

$$f = \frac{v_{sound}}{2L}$$

I think it is only valid for one-dimensional ducts such that diameter $\ll$ length but I am not sure about this. Could you please help me out with an explanation and how the above formula was derived and in which conditions is it valid to be used?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ This formula is for a cylinder open at one end. Is this your type of cylinder? $\endgroup$
    – nasu
    Commented Mar 21, 2019 at 19:51
  • $\begingroup$ Yes that's right. Thank you. I have just corrected the formula. $\endgroup$
    – Becay
    Commented Mar 22, 2019 at 4:52

1 Answer 1

Reset to default
1
$\begingroup$

This can be derived by simply using the a very fundamental equation that λ𝑓=𝑣.
For fundamental or the lowest frequency the system can achieve, the wavelength should be maximum, for a given wave speed.The dots in the background show pressure compressions and rarefactions

Putting the maximum wavelength of the wave using the diagram, you would get the desired relation.
This relation is derived by using the basic definitions of wave and hence is valid in general for waves where the two end points to be considered are nodes.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Meaning that the formula can also apply even in three dimensional spaces? $\endgroup$
    – Becay
    Commented Mar 28, 2019 at 9:38
  • $\begingroup$ Yes, i believe so, also actually this disturbance happens in 3 dimensional space, where the air moves in packets of compression and rarefaction. $\endgroup$
    – Sidharth Giri
    Commented Mar 28, 2019 at 19:20
  • 2
    $\begingroup$ For the OP's situation where the length = diameter, this will give only a small number of the possible resonances. For example, a rectangular box (e.g. a room) with dimensions $x \times y \times z$ has resonances $\dfrac v 2 \sqrt{\left(\dfrac i x\right)^2 + \left(\dfrac j y\right)^2 + \left(\dfrac k z\right)^2}$ for all integers $i. j, k >= 0$, where $v$ is the speed of sound. Similar formulas for a "wide" cylinder are more complicated. $\endgroup$
    – alephzero
    Commented Jan 31, 2021 at 19:16

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.