Understanding the bandgap in $E-k$ diagram in the nearly free electron model

Question

The nearly free electron model, solved using perturbation theory, reveals that a band gap opens up at each Brillouin zone boundary. Why is a point just below $k=\pi/a$ lowered in energy and a point just above $k=\pi/a$ raised in energy? Why is it not the other way around? I have followed the mathematics (of perturbation theory) from Steve Simon's book and also from David Tong's lecture notes. But this point is not addressed there. Any clue?

Answer 1

As a quick review, the Bloch Hamiltonian for the free electron model with (arbitrary) lattice spacing $a$ is $$H_k:= \frac{1}{2}(-i\nabla+k)^2$$ which has eigenvectors and eigenvalues $$u_{nk}(x) = \frac{1}{\sqrt{a}}e^{2\pi i nx/a}\qquad E_{nk}= \frac{(k+2\pi n/a)^2}{2}$$ For most values of $k$, these eigenvalues are non-degenerate. However, there are two points in the Brillouin zone for which this isn't true; the first is at $k=0$, where $E_{n0} = E_{(-n)0}$, and the second is at $k=\pm \pi/a$ (which we identify as the same point), where $E_{n(\pm \pi/a)}= E_{m(\pm \pi/a)} $ whenever $n+m=\mp 1$.

---

When we add in a generic perturbation, it is the lifting of these degeneracies which corresponds to the opening of a gap. For example, we might add a cosinusoidal perturbation $$V(x) = -\lambda\cos(2\pi x/a) = -\frac{\lambda}{2} (e^{2\pi i x/a}+e^{-2\pi i x/a})$$

The naive first order corrections to the energy eigenvalues are easily seen to vanish: $$\langle u^{(0)}_{nk},Vu^{(0)}_{nk}\rangle = \frac{1}{a}\int_0^a V(x)\mathrm dx = 0$$ When computing the first order corrections to the energy eigenvectors, we immediately run into a problem:

$$u_{nk}^{(1)} = -\sum_{m\neq n}\frac{\langle u_{mk},V u_{nk}\rangle}{E_m^{(0)}-E_n^{(0)}} u_{mk}$$

The aforementioned degeneracies cause the naive correction to blow up, rendering our perturbative expansion invalid. When we're near one of the $k$'s where these degeneracies arise, we should use degenerate perturbation theory instead.

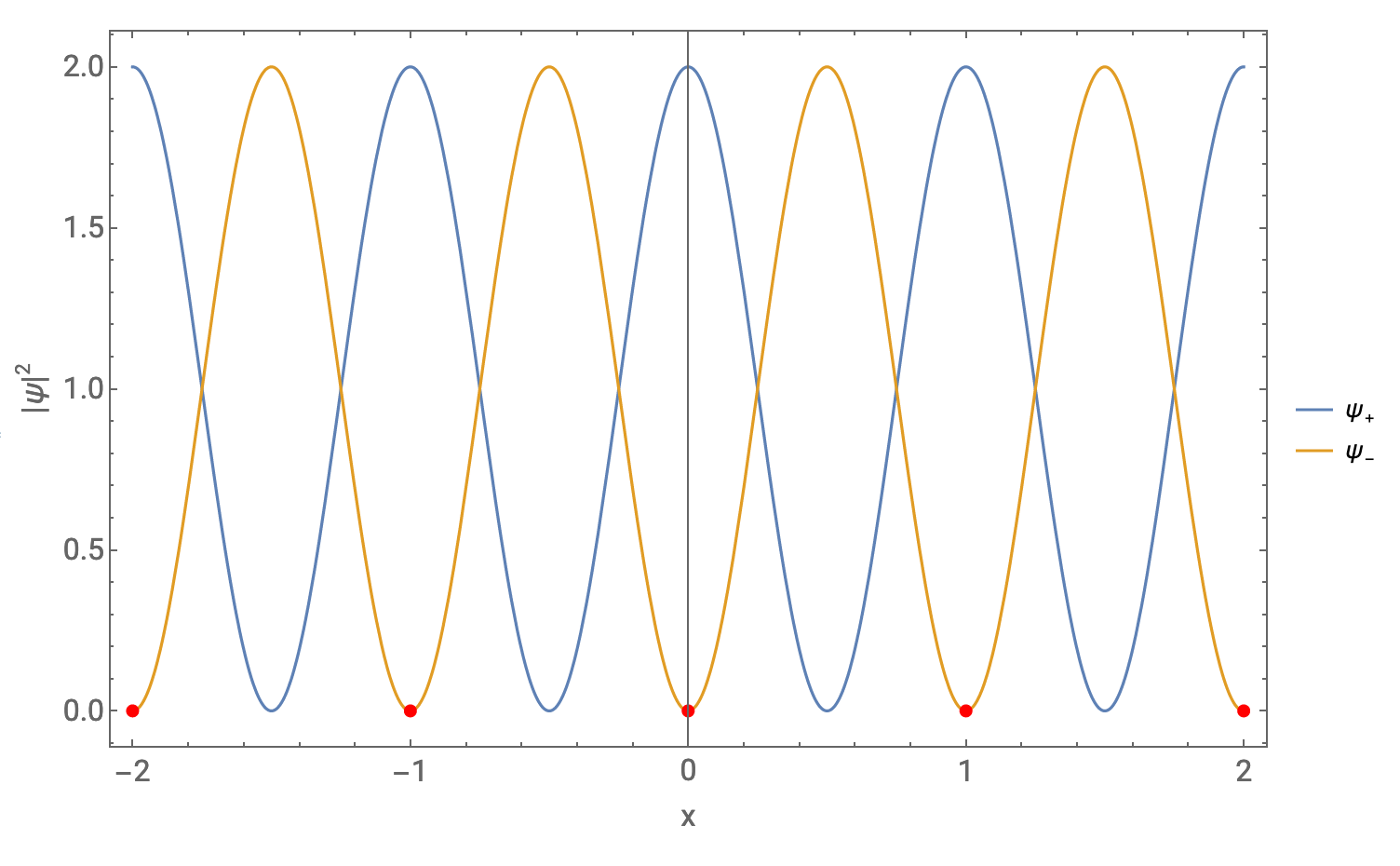
To be specific, we might consider the degeneracy between $u_{0,\pi/a}$ and $u_{1,\pi/a}$. The correct approach is to choose eigenfunctions $\psi_\pm$ which are linear combinations of the degenerate $u$'s but which also diagonalize the perturbation. The $2\times 2$ block of $V$ corresponding to our degenerate subspace is $$\tilde V = \pmatrix{0 & -\lambda/2\\-\lambda/2 & 0}\qquad \tilde V_{nm} = \langle u_{n(\pi/a)},V u_{m(\pi/a)}\rangle$$ Clearly this is diagonalized by $$\psi_{\pm} = \frac{1}{\sqrt{2}}(u_{0,\pi/a} \pm u_{1,\pi/a})$$ and so the first order corrections to our energies are $$E^{(1)} = \mp\frac{\lambda}{2}$$ and the appropriate eigenvectors are to zeroth order $$\psi_+ = \frac{1 + e^{2\pi ix/a}}{\sqrt{2a}} \qquad \psi_- = \frac{1-e^{2\pi ix/a}}{\sqrt{2a}}$$

---

Why is a point just below $k=\pi/a$ lowered in energy and a point just above $k=\pi/a$ raised in energy?

I don't think that's the right way to look at it. It's not that $u_{0,\pi/a}^{(0)}$ is pushed down in energy while $u_{1,\pi/a}^{(0)}$ is pushed up. Instead, the symmetric linear combination $\psi_+$ is pushed down while the antisymmetric linear combination $\psi_-$ is pushed up.

The reason for this is not hard to see:

The red dots are the ions. Note that in $\psi_+$, the probability density for the electron is maximized right on top of the ions. Because $V(0)<0$, the energy of this state is lowered by the perturbation. On the other hand, in $\psi_-$ the probability density for the electrons is maximized at $\pm a/2$, and because $V(\pm a/2)>0$, the energy of this state is raised by the perturbation.

This is an illustration of a very general rule from perturbation theory - when you have a degenerate subspace $\mathscr D$, then there's no unique choice of basis for $\mathscr D$. When we turn on a perturbation, we can reasonably expect the perturbed eigenfunctions to be small corrections to some basis of $\mathscr D$, but certainly not every basis of $\mathscr D$. In our case, at $k=\pi/a$ we see that the perturbed eigenstates are not given by small corrections to $u_0$ and $u_1$, but rather to $\psi_\pm$. The states which are pushed up or down in energy are not $u_0$ or $u_1$, but $\psi_+$ and $\psi_-$.