Nearly Free Electron Model (Perturbation Theory)

Question

I am having difficulty understanding the degenerate perturbation theory treatment of the nearly free electron model. So for a free electron, the energy dispersion is relation is $E^{0}=\frac{h^{2}k^{2}}{2m}$. Assuming the 1d lattice constant is $a$ and so the two states at $|k=\frac{\pi}{a}\rangle$ and $|k'=\frac{-\pi}{a}\rangle$ will satisfy Bragg condition and will be degenerate since they have the same energy (i.e $E_{k}^{0}=E_{k'}^{0}=\frac{h^{2}{\pi}^{2}}{2ma^{2}}$). So assuming the periodic potential is $V$ then the first order energy correction for the energies of $|k=\frac{\pi}{a}\rangle$ and $|k'=\frac{-\pi}{a}\rangle$ are the eigenvalues of the following matrix:

$$\begin{pmatrix} \langle k|V|k\rangle & \langle k|V|k'\rangle \\ \langle k'|V|k\rangle \ & \langle k'|V|k'\rangle \\ \end{pmatrix}=\begin{pmatrix} 0 & V_{G} \\ V^{*}_{G} \ & 0 \end{pmatrix}$$ which are $\lambda = \pm\left| V_G\right|$. So am I right to assume that the state $|k'=\frac{-\pi}{a}\rangle$ is pushed down by - $\left| V_G\right|$ and state $|k'=\frac{\pi}{a}\rangle$ is pushed up by $\left| V_G\right|$. I know this is incorrect since the gap opens up in both directions (up and down) at $k=\frac{\pi}{a}$ and $k=\frac{-\pi}{a}$so I want to know what I am missing.I attached a picture to show the results of perturbation theory. However, this is not correct and we actually get two more states after perturbation and a bandgap of $2\left| V_G\right|$. So how can we start with two states before perturbation and then end up with four states after perturbation (see picture).Could anyone explain why this is the case?

Answer 1

There is a misconception in the question. The states don't somehow multiply when we introduce the periodic potential and shift energies at the Brillouin zone boundary up and down. Instead what we are doing is changing the basis from the free-particle plane-wave basis to one that (approximately) diagonalizes the full Hamiltonian (which includes the kinetic energy and periodic potential terms). Once the periodic potential is introduced, the plane-wave states are no long states of well-defined energy (they are not energy eigenstates), and so they cannot be labeled with energies. Instead, it is particular linear combinations of the plane-wave states that are eigenstates of the Hamiltonian, and it is these states that can be labeled with energies.

(More detail below the break.)

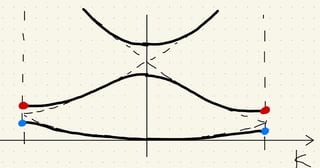
There is another possible cause of the misconception. We don't shift both states ($k=\pm \pi/a$) both up and down, making four states. Instead, these two sates combine to form two new states, one which is shifted down and one which is shifted up. When we draw the dispersion relation is reduced zone scheme, the states at the Brillouin zone boundary with the same energy are the same state (i.e., you kind of have to think of the Brillouin zone as having "periodic boundary conditions", in a sense. Consider the picture below: the dots with the same color are actually the same state. It looks as if, in this picture, we have created four states out of two, but that's just a problem with our picture.

---

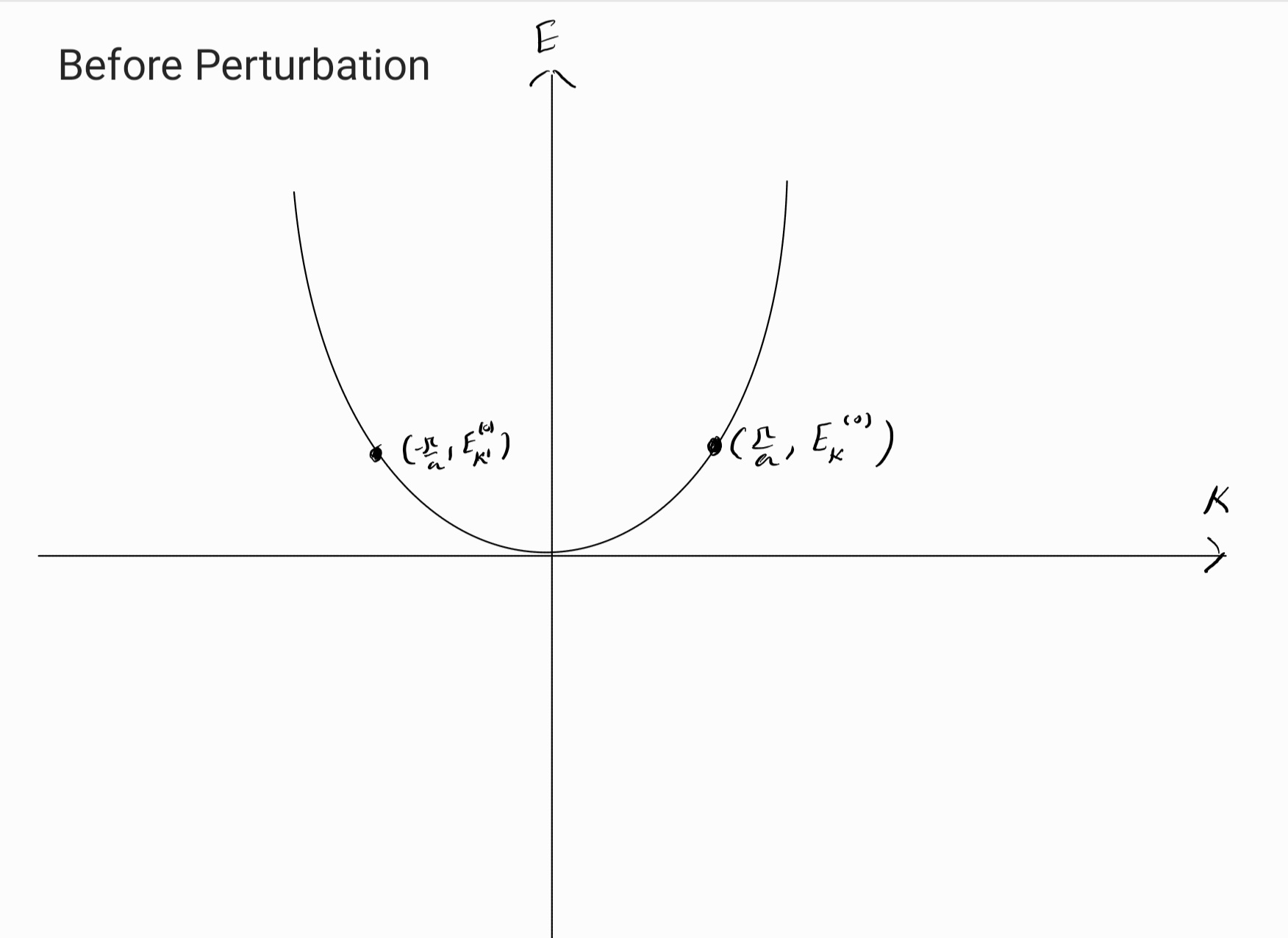
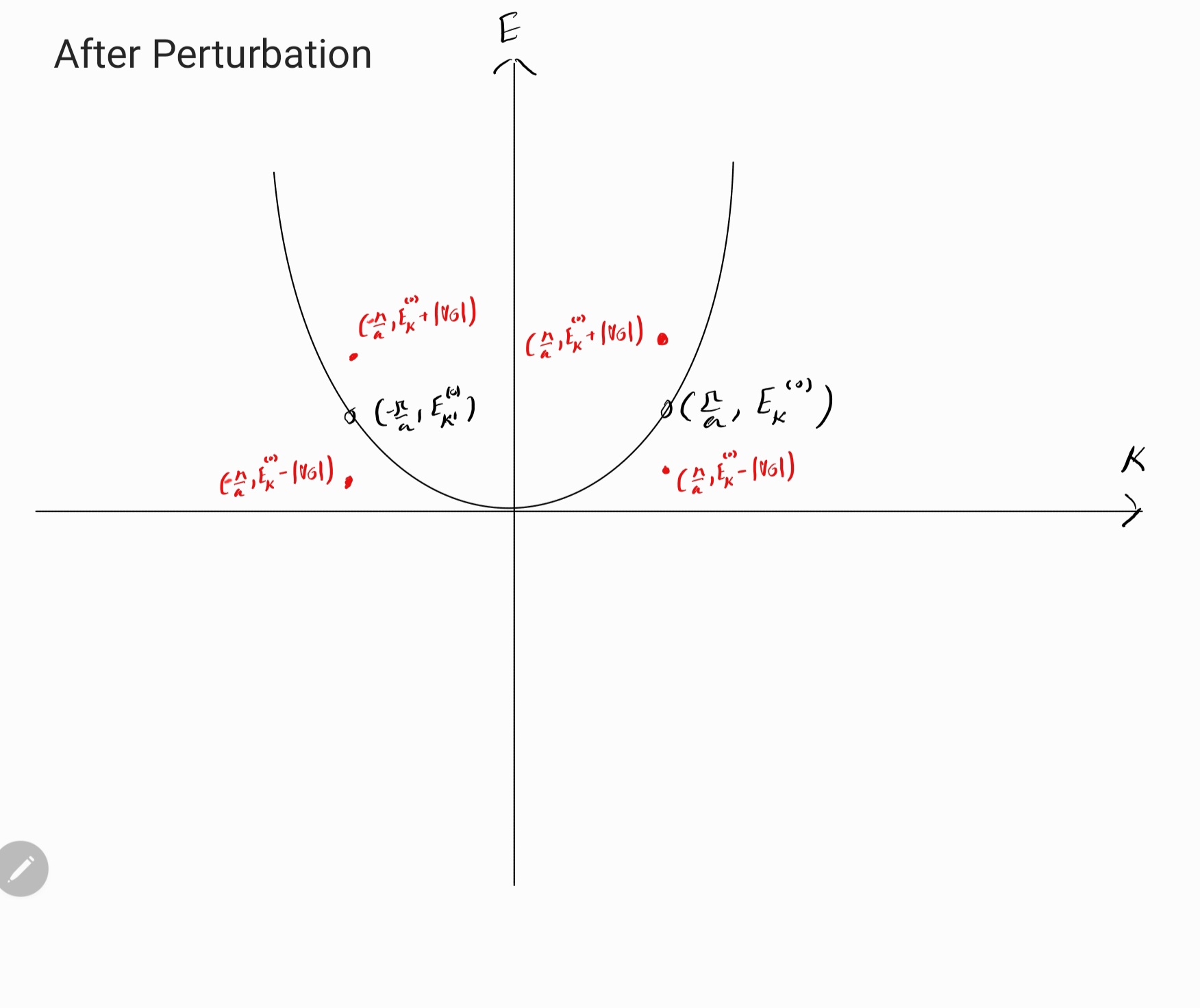
We want to diagonalize the full Hamiltonian, including the free-particle term (kinetic energy $\hat{T}$) and the periodic potential $\hat{V}$. We do this approximately by using perturbation theory. Due to the periodic perturbing Hamiltonian, the plane wave state corresponding to the free-particle dispersion relation that you are showing are no longer energy eigenstates. Instead, you must form linear combinations of the plane wave state to construct the states of well-defined energy (i.e., energy eigenstates).

Thus, you are changing the basis from the set of plane-wave states to the set of energy eigenstates of the full Hamiltonian. This is difficult to do in general, but for the states at the Brillouin zone boundary (at $k=\pm \pi/a$), we can make a good approximation that the eigenstates are the symmetric and anti-symmetric combinations of the corresponding plane-wave states. This is in fact what results from diagonalizing the two-by-two matrix shown in the OP: the eigenvectors of that matrix are $(1,1)$ and $(1,-1)$, and so the resulting (approximate) eigenstates are just $$ \psi_{\pm}(x) = \frac{e^{\pi i x/a}\pm e^{-\pi i x/a}}{\sqrt{2}}\,. $$ These are clearly no longer plane-wave states. The plane-wave states are not part of the new basis. They still exist, but they're not energy eigenstates, so you cannot label them with energies, as OP is doing in the picture.

Part of the problem might be that the symbol $k$ is often used in this context to denote two different (but related) things. For plane-waves, it's just the wave-vector (related to the momentum). But once you've introduced the periodic potential and "folded" the dispersion relation over into the first BZ, $k$ now corresponds to quasi-momentum (aka crystal momentum), the momentum-like quantity conserved in systems with discrete translational invariance. Momentum $k$ labels the plane-wave basis, and quasi-momentum $k$ labels the Bloch basis, constructed using particular linear combinations of the plane-wave states.