1
$\begingroup$

I am told that the image created by a parabolic mirror is the same size as the object when it is on the center of curvature, which is twice away from the focus of the parabola. I tried to prove this in the following way:

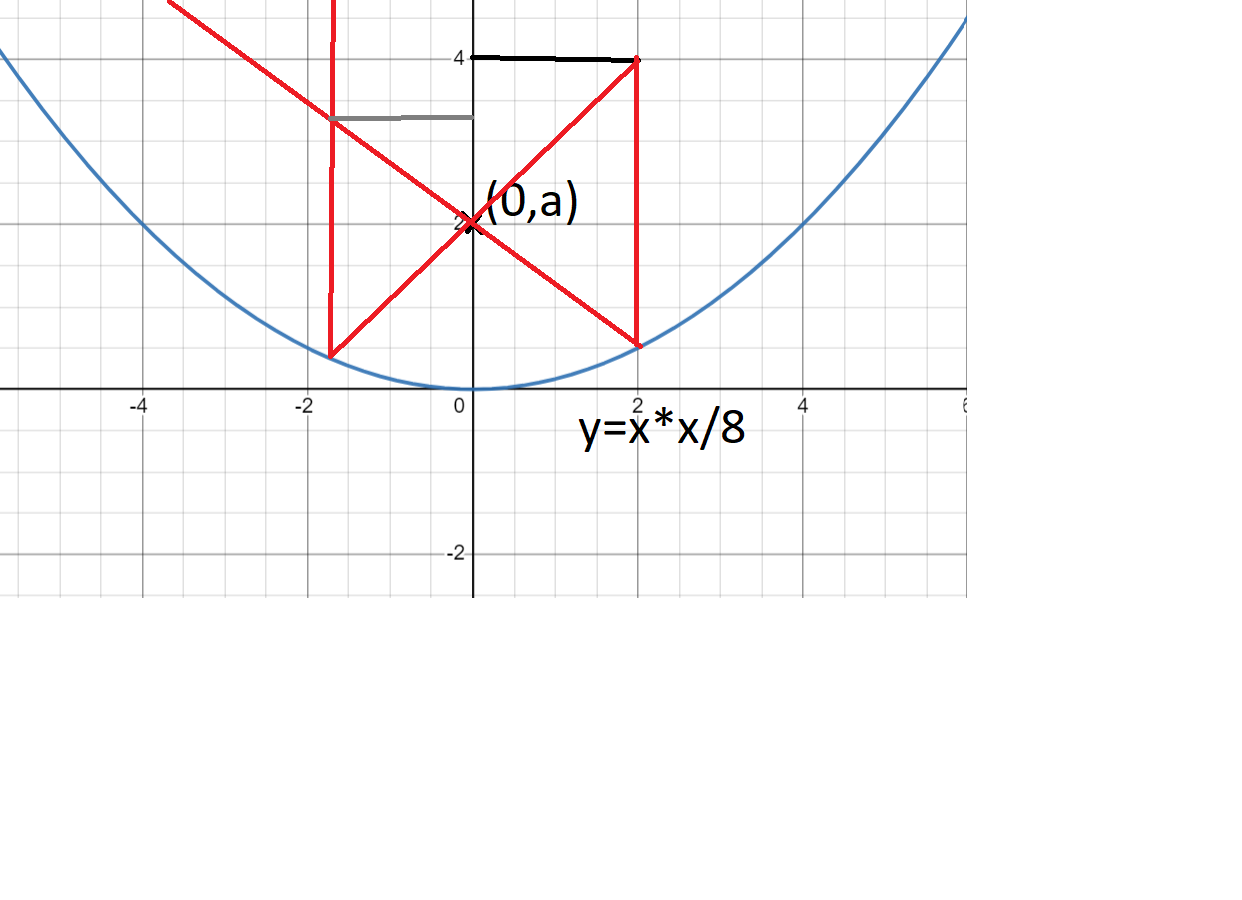
Given a parabola with focus $(0,a)$, we obtain the equation $y=x^2/4a$. Now, the center of curvature is at $(0,2a)$, and hence an object of length $l$ must have its highest point at $(\pm l,2a)$. Let's choose $(l,2a)$ as the highest point, in which case the image should have a lowest point $(-l,2a)$.

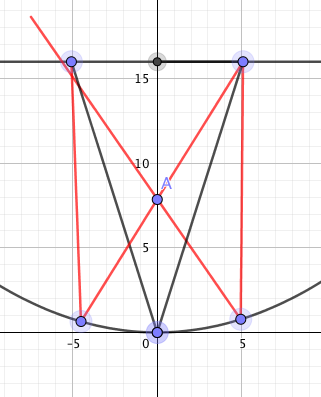
Now, the standard procedure to find the image is to draw 2 lines from $(l,2a)$; one that intersects the focus (0,a) and another that is parallel to the principal axis of the parabola, as shown by the figure below.

Parabola

The former creates a line that goes through the points $(l,2a)$ and $(0,a)$ and has the form

$$y=\frac{a}{l}x+a$$

But we see that this doesn't intersect the parabola $y=x^2/4a$ at some point with $x$ coordinate $-l$, leading me to believe that the image isn't actually the same size.

The figure corroborates this geometrically as well.

Is the image of an object at the center of curvature of a parabolic mirror actually the same size as the object itself?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
0
$\begingroup$

I think that it is an image in the Gaussian approximation: rays not very far away and not very inclined with respect to the axis of symmetry (approximate stigmatism). In this case, we can use the usual conjugation relation for spherical mirrors. It indicates that for an object which is twice away from the focus, the image is at the same place as the object, the same size but reversed from the object.

Hope my poor english is OK !

Improve this answer
$\endgroup$
4
  • $\begingroup$ Are you sure? I have seen multiple sources that state the image to be exactly the same size. For instance, Khan Academy: khanacademy.org/science/physics/geometric-optics/mirrors/v/…, $\endgroup$
    – pjq42
    Commented Jul 21, 2022 at 17:01
  • $\begingroup$ At 7mn22 of this video, I see an image which is at the same location than the object, the same size but reversed as predicted by the conjugation relation ? $\endgroup$
    – Vincent Fraticelli
    Commented Jul 21, 2022 at 17:11
  • $\begingroup$ Yes, and it is explicitly said that this should always be the case when the object is on the circle of curvature. However, my question contradicts that. $\endgroup$
    – pjq42
    Commented Jul 21, 2022 at 17:15
  • $\begingroup$ I have not repeated your calculations but I think the problem is that you are reasoning using a geometrical construction that is only valid in the Gaussian approximation. In the general case, the emerging rays do not intersect at a single point: there is no image in the strict sense. We must assume that the rays are not very inclined and not very far from the axis. $\endgroup$
    – Vincent Fraticelli
    Commented Jul 21, 2022 at 17:29
0
$\begingroup$

You are right! if you really construct a picture for any parabolic mirror and use more than just two rays , the meet at different points so there will mot be a sharp picture, in your image you could use one more ray, so one going to 0 and be reflected in the same angle. In reality it is a good approximation, but only with very flat parabola and objects not to large, Thats why to construct pictures one uses completely flat object instead of a real parabola I made picture with a much flatter parabola and it is almost true. the mirrors pos used are about ^10 cm in diameter and a focus length oenter image description heref 10 to 20cm

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.