1
$\begingroup$

A mobile phone lies along the principal axis of a concave mirror with its distorted image, as shown in Fig.

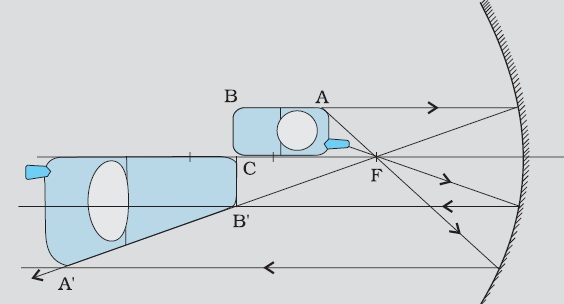
enter image description here

How do we actually figure out the formation of such images? The answer says: "The image of the part which is on the plane perpendicular to principal axis will be on the same plane. It will be of the same size." I require further explanation.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Supposing the antenna was pushed over to the principal axis, if one imagined the rest of the phone vanishing, the part about having the same size would clearly not apply. Also, there is no "the plane perpendicular to", there are infinitely many such planes. So it leads me to believe that the normal plane through C has been singled out for special treatment. The equal lengths of CB and CB' can be explained, then. $\endgroup$
    – rschwieb
    Commented Nov 29, 2014 at 11:26
  • $\begingroup$ I've never taken a course on optics, but I know a lot about geometry. I don't get the choice of drawing the bases of the images lining up this way. For a regular mirror, images appear to be the same distance away as their reflections, but that clearly isn't obeyed here. It could be a principle of optics I don't know. But still, the thought experiment i suggested seems to collide with that idea. $\endgroup$
    – rschwieb
    Commented Nov 29, 2014 at 11:31

1 Answer 1

Reset to default
2
$\begingroup$

For a parabolic mirror, lines that start parallel to the main axis are reflected through the focal point. Lines that start out through the focal point are reflected parallel to the axis.

This simple rule allows you to draw two lines from any point in the object that will intersect in the corresponding point in the image.

Just try this in the image you drew and you'll see that it works.

Note that the same thing is almost true for a spherical mirror - for small angles the difference between sphere and parabola is very small but as you get further off-axis the difference does start to matter. But for the purpose of your original question I don't think you need to worry about it.

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.