1
$\begingroup$

This question appeared on this site Q17: here

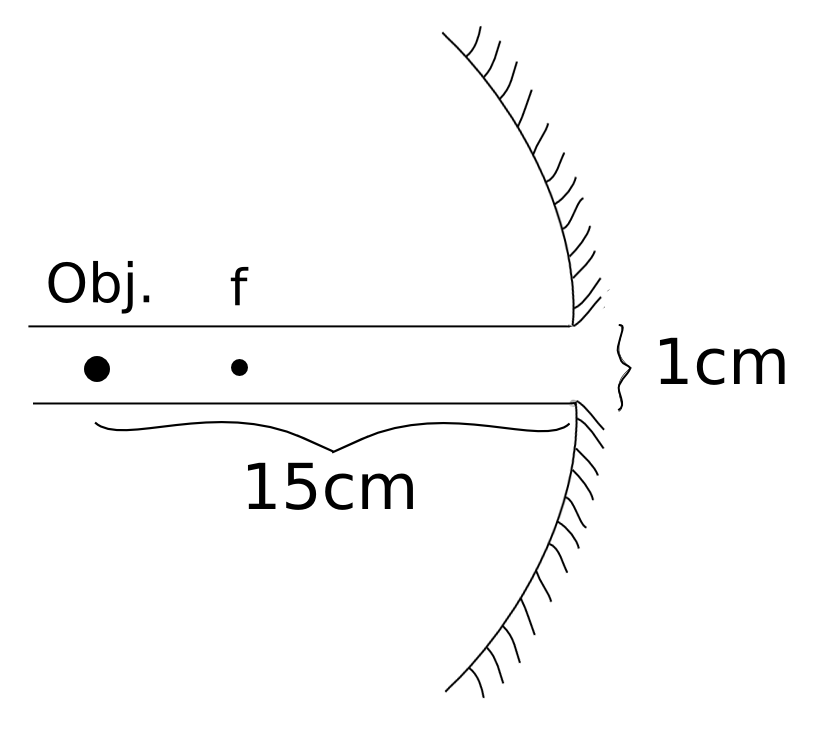
A concave mirror is broken into two parts and these parts are separated by a distance if 1 cm. The focal length of the mirror is 10 cm. Find the location of images(in cm), formed by the 2 parts of the mirror. The object is midway between the two principal axes at a distance of 15 cm from P.

I don't know whether the principal axis of the mirror is parallel to the principal axis of the mirror below. How do we define the principal axis here? what will the location of the image be? thank you.

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ Can we assume, unlike your drawing, that the two mirror segments were separated purely in translation? (that is, the "inner" edges at the 1-cm gap have slope infinity). I'm guessing the problem wants you to see that each segment forms an image as would have been formed if the entire mirror had been moved $0.5 cm$ up or down. $\endgroup$
    – Carl Witthoft
    Commented Nov 22, 2014 at 17:47

2 Answers 2

Reset to default
4
$\begingroup$

The 2 "half mirrors" separated by 1 cm is equivalent to 2 full mirrors whose principal axis' are at a distance of 1 cm from each other , with the object in between as shown in the figure. F1,F2 are the 2 focal lengths

From the figure, we can say that the object distance are the same for the 2 mirrors. The only thing different will be the image heights, whose direction will be opposite of each other and the magnitudes will be the same.
Using the mirror formula, we get the images will be formed at a distance 30 cm in front of the mirror and at a height of 2 cm on either sides of the original principle axis.

Improve this answer
$\endgroup$
3
$\begingroup$

If you have a normal concave mirror, the image is formed from all parts of the lens, and hence covering any part of the mirror just reduces the intensity of the image and does not affect its position.

Now, in your case, we are also translating the mirror perpendicular to the principal axis. This changes the "height" of the object but not the "distance" from the mirror.

Assume origin at the center of the mirrors, we can write $$u = -15 \; \text{cm}, \, f = -10 \; \text{cm}$$ which implies $$v = \frac{uf}{u-f} = -30 \; \text{cm}$$

The "height" of the object is $0.5 \; \text{cm}$, and magnification is $$-\frac{v}{u} = -2$$ Thus the images are formed at $$y = \pm 2 \; \text{cm} ,\, x = -30 \; \text{cm}$$

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.