I had uploaded the same problem in maths stack exchange but since it got no answer and because I think that it is a problem that can be seen both as a physical one I considered uploading it both on PSE. (Original post: A circle and the probability as a function of time). So here is the problem: Supposing that we have a circle of radius $R$ which at $t=0$ does not contain any sphere as we a wall surrounds it allowing no sphere to enter in it. At $t=0$ we remove the circular "wall". In the space outside the circle, we suppose that there exist spheres of negligible dimensions all moving at a steady speed of magnitude $u$ in completely random directions. We suppose that the whole system is located inside a rectangular box such that the dimensions of the system(Circle-spheres) are considered negligible. If there exist inside the rectangular box $N$ spheres and the box has a total surface of $S$ what is the probability as a function of time that after we remove the "wall" the circle remains empty? (consider everything is in 2d and also consider that the spheres do not interact with each other when they come in contact) Is this problem solvable? (This problem is not any homework and I haven't seen any similar to this. The only case I have seen probability being a function of time is in the quantum mechanics wave function. I would appreciate it if someone could inform me if there are any maths for problems including probability as a function of time?) (In order for this question to not be considered as a solution-asking one in homework exercises, somebody could just tell me if the problem is solvable and if the probability-time relation is a regular thing in physics\maths). Thanks in advance for any help. Edit: By the "the spheres do not interact with each other" I mean that the spheres pass through each other so we don't have to take their collisions into consideration. Also, it is true as noted in the comments that "spheres of negligible dimensions" implies that they are seen as points moving on the plane. I apologize if any confusion occurred. 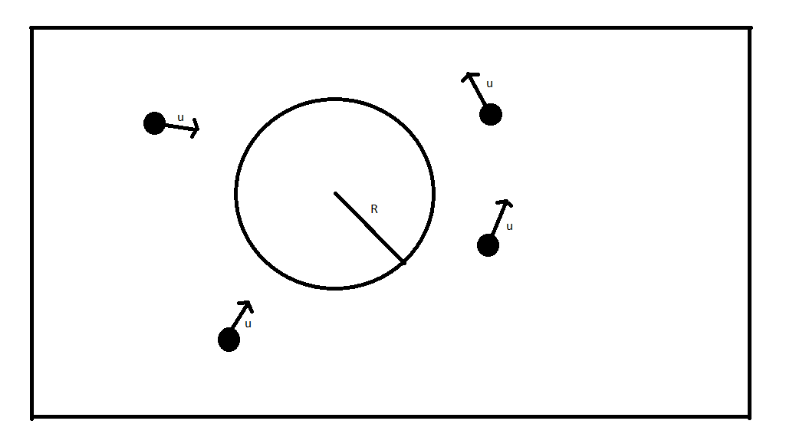
-
1$\begingroup$ "consider everything is in 2d" The spheres are therefore circles? What would be their area? But then "spheres of negligible dimension"? Then they're points? Exactly how does a sphere occupy the region over a flat surface? When you say the spheres do not interact, does this also mean they do not collide? You really have to include a lot more information. Have you tried to do this by starting with the simplest case, which would be the same problem but with one "sphere", then seeing what you'd get in the case of an arbitrary number N? $\endgroup$– joseph hCommented Jun 25, 2022 at 23:03
-
2$\begingroup$ I do not know the probabilities, but think about it as a gas. Once you remove the partition the molecules will immediately start filling the previous gap (even if they can only collide with the walls. The larger the N the faster. I am sure someone with good knowledge of statistical mechanics could answer this more precisely. I am commenting just in case you don't get any answers. $\endgroup$– user338734Commented Jun 25, 2022 at 23:04
-
$\begingroup$ @joseph h it is true that the information I give is inadequate so I made some edits. The "negligible dimension spheres" means that they are seen as points while the fact that the system is considered 2d makes the "spheres" statement incorrect. Furthermore the fact that "spheres do not interact" means that they pass through each other when they are supposed to collide (It would be afterall impossible to consider the collisions of simple points ). Regarding your view that analyzing it for one sphere at first and then generalizing it would answer I think it's easier to do it for large N at first. $\endgroup$– Kani PenCommented Jun 26, 2022 at 13:13
1 Answer
I assume classical mechanics applies to this problem.
If by the spheres "do not interact with each other" you mean no collisions, then only those spheres initially headed in a direction that hit the circle are counted.
I assume by the spheres "do not interact with each other" you mean they can only elastically collide. You would have to do a simulation stepping thorough time to calculate collisions and the final velocity (vector) of each sphere considering oblique elastic collisions. As an approximation you can treat the particles as points of equal mass that only change direction (not speed) after an elastic collision. Particles can both enter and exit the circle. If you remove the wall, particles can escape the box, but if the wall remains particles scatter off the wall. Over a long time (steady state), if the wall is removed and there are no collisions outside the wall (no reflection of particles back into the box) all particles eventually escape the box. Over a long time (steady state), if the wall is not removed, the fraction of particles inside the box is equal to the ratio of the circle area to the box area.
There may be a good answer from statistical mechanics, but I am not an expert in that area. Perhaps others can address this as @Carlos Gauss suggests in an earlier comment.
-
$\begingroup$ By the expression "do not interact with each other" I mean that when they are supposed to collide they instead pass through each other. $\endgroup$– Kani PenCommented Jun 26, 2022 at 8:07
