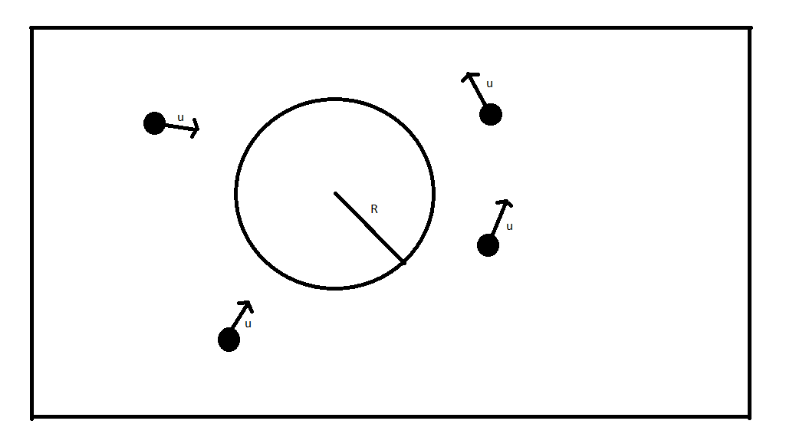
I had uploaded the same problem in maths stack exchange but since it got no answer and because I think that it is a problem that can be seen both as a physical one I considered uploading it both on PSE. (Original post: A circle and the probability as a function of time). So here is the problem: Supposing that we have a circle of radius $R$ which at $t=0$ does not contain any sphere as we a wall surrounds it allowing no sphere to enter in it. At $t=0$ we remove the circular "wall". In the space outside the circle, we suppose that there exist spheres of negligible dimensions all moving at a steady speed of magnitude $u$ in completely random directions. We suppose that the whole system is located inside a rectangular box such that the dimensions of the system(Circle-spheres) are considered negligible. If there exist inside the rectangular box $N$ spheres and the box has a total surface of $S$ what is the probability as a function of time that after we remove the "wall" the circle remains empty? (consider everything is in 2d and also consider that the spheres do not interact with each other when they come in contact) Is this problem solvable? (This problem is not any homework and I haven't seen any similar to this. The only case I have seen probability being a function of time is in the quantum mechanics wave function. I would appreciate it if someone could inform me if there are any maths for problems including probability as a function of time?) (In order for this question to not be considered as a solution-asking one in homework exercises, somebody could just tell me if the problem is solvable and if the probability-time relation is a regular thing in physics\maths). Thanks in advance for any help. Edit: By the "the spheres do not interact with each other" I mean that the spheres pass through each other so we don't have to take their collisions into consideration. Also, it is true as noted in the comments that "spheres of negligible dimensions" implies that they are seen as points moving on the plane. I apologize if any confusion occurred.