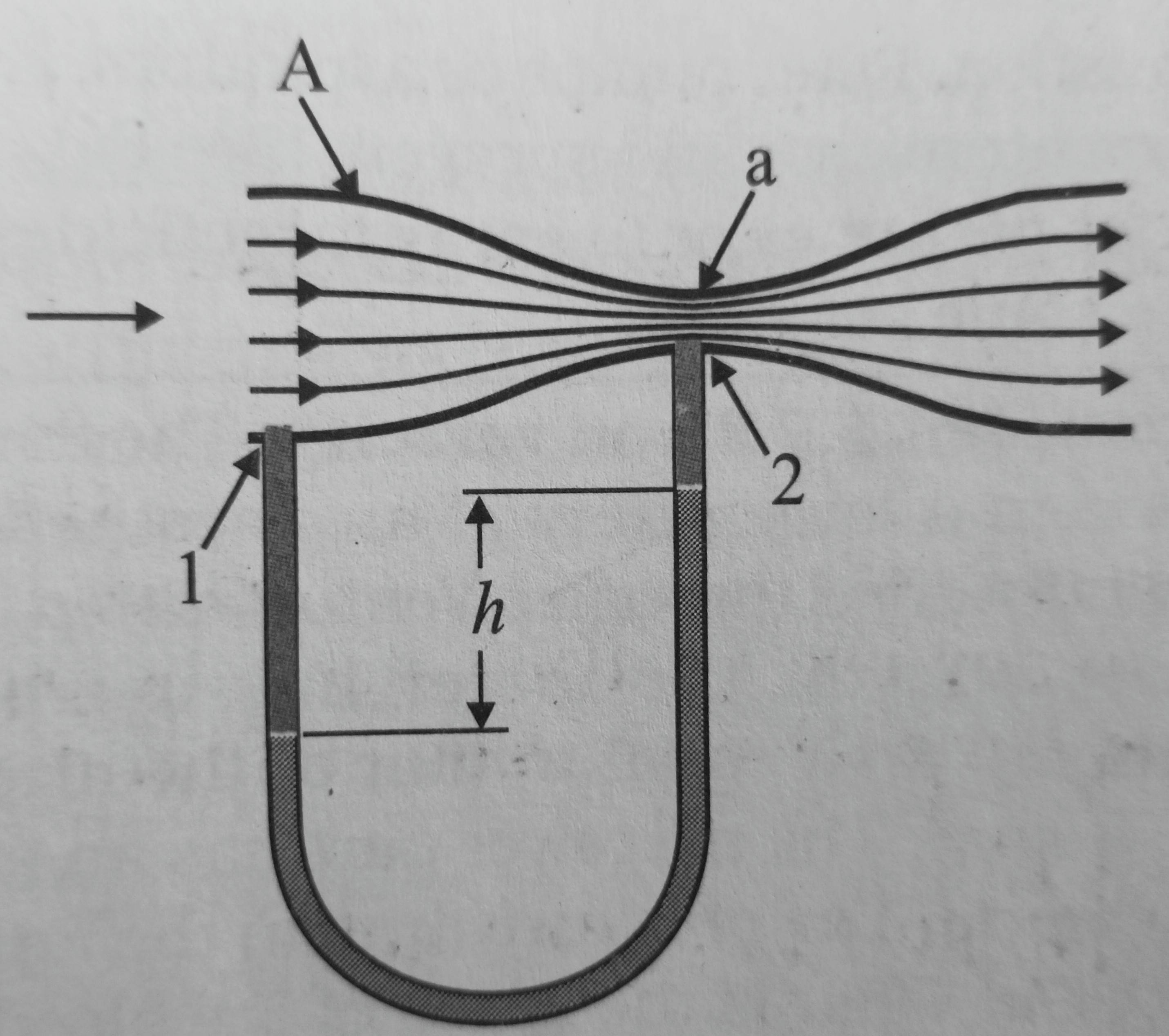
The following is the picture of a venturi-meter attached with a u-tube manometer. We have a fluid flowing in the direction as shown through cross sectional areas $A$ and $a$. We find an expression for $v_1$ which is velocity at $A$ through Bernoulli principle, continuity equation.
My question:-
Why is the pressure on fluid at $1$ and at cross sectional area $A$ the same?
The flow is constrained by the walls to only flow in one direction at point 1.
Under the assumptions of Bernoulli's law, if the flow velocity has only a component in one direction then it is not possible to have pressure gradient in the other direction. Otherwise it would flow in the other direction, but it can't because it is constrained.
If you look at the stationary flow equations in the radial direction: $$0 = -\partial_y p + \mu\partial_x^2 u_y$$ when $\vec{u}=(u_x, u_y) = (U, 0)$ then clearly the $y$-gradient of the pressure must vanish, i.e. there is no pressure differences along the cross-section.
