I'm currently studying a introductory section on fluids and was given this principle of continuity: $$A_1 v_1 = A_2 v_2$$ I understand the logic behind it (viz. the conservation of mass and whatnot). But I do wonder whether that implies constant fluid velocity given equal cross-sectional area. If $A_1 = A_2$, then $v_1 = v_2$. So it doesn't matter what happens in the middle (if the pipe goes up and down, if there's a pump in the middle, and whatnot), so long as the fluid is flowing somehow, the velocity of the fluid is constant. Is that understanding correct? If that's true, why does textbook also say that: "Left to itself, the fluid will slow down on the way to the top, because it's got to work against gravity?" (Referencing a figure that involves a pipe which rises in elevation)
-
$\begingroup$ You are right! The fluid's pressure will drop as you ascend. $\endgroup$– DanDan面Commented Jun 15 at 19:00
-
$\begingroup$ The pressure will drop from Bernoulli's Equation. Will the fluid velocity constant? By continuity, it seems like it will. It seems like the pressure drop (increase in v) is counteracted by gravity (decrease in v) to maintain constant velocity. But, the book is saying that fluid will slow down as it goes up (which obeys common sense). Which is right? $\endgroup$– procommaniaCommented Jun 15 at 19:08
-
$\begingroup$ Also, if you take a level pipe of equal cross-sectional area (no change in elevation) and consider Bernoulli's Equation, $P_1 - P_2 = \frac{1}{2} \rho v_2^2 - \frac{1}{2} \rho v_1^2$. Thus a change in pressure (pump?) would effect a change in velocity, but that can't happen by continuity because $A_1 = A_2$. Does that mean that pressure is constant given $A_1 = A_2$? $\endgroup$– procommaniaCommented Jun 15 at 19:13
-
$\begingroup$ The equation that you quote, $A_1 v_1 = A_2 v_2$, is a version of the law of conservation of mass whereas once there is a change of height, pressure, etc, you need another equation which is based on the law of conservation of energy. $\endgroup$– FarcherCommented Jun 15 at 21:37
-
$\begingroup$ "Left to itself, the fluid will slow down on the way to the top, because it's got to work against gravity?" Did the book elaborate on what it meant bye "Left to itself"? $\endgroup$– Bob DCommented Jun 15 at 22:02
1 Answer
Assuming Ideal situation (where there is no friction in pipe, laminar flow, incompressible fluid etc.), you are right that if area of pipe is uniform then the velocity will not change. But this only happens when water is continuously coming in from one end of the pipe. Because the derivation of equation of Continuity for flow of fluid assumes steady flow of fluid along with the condition that the cross section of pipe must be fully occupied by fluid during flow. That is pipe is not partially filled.
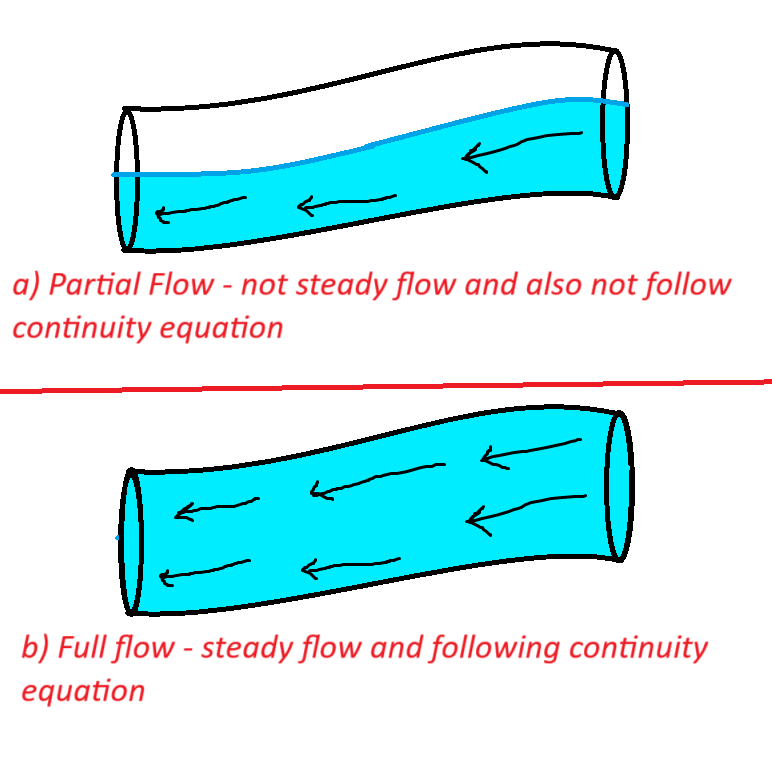
If this condition is satisfied then yes, the velocity will remain same whatever the pipe orientation is, whether it goes up or down. (The pump, in general, will change the velocity hence it should not be included here).
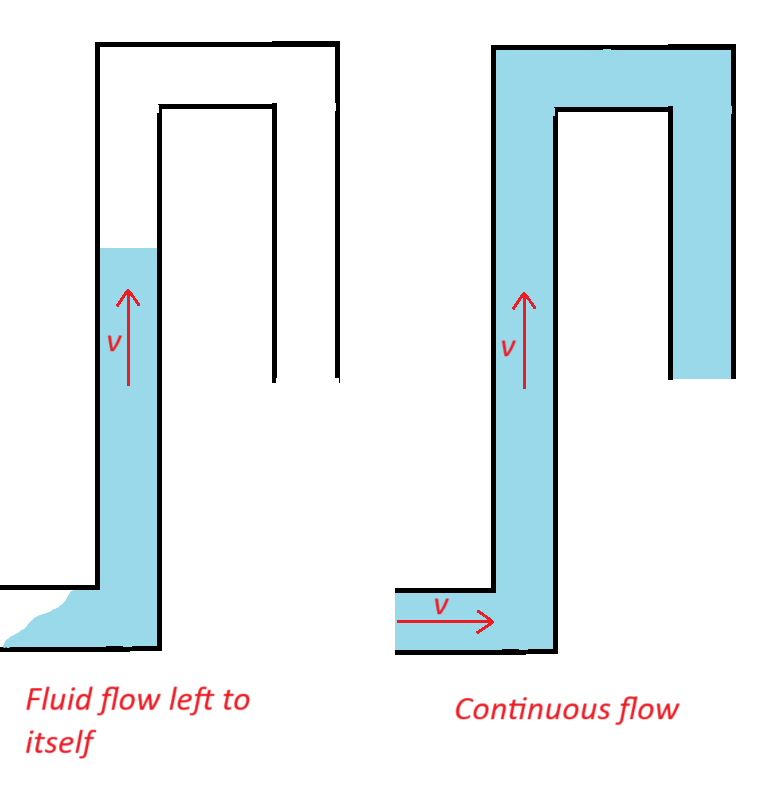
From what I can understand, the book is saying that when a chunk of fluid is set to flow in a vertical pipe, without any external force acting on it, the fluid flow slows down because of conservation of energy (Increase in potential energy due to work done by gravity decreases the Kinetic energy, so that total energy is constant).
But when the fluid is continuously being fed in from one end of pipe then the push force that exerts on the fluid, from behind, counters the work done by gravity and hence the velocity of fluid will remain constant in all parts of pipe, whether it goes up or down, following the equation of continuity $Av_1=Av_2$.
Also the constantly feeding of fluid in pipe requires an external force, like pump, and indirectly this force counters the work done by gravity.
-
$\begingroup$ Thank you for the explanation. The illustrations are very helpful. One other question. In full flow, if we have a pump (and one end of the pipe is connected to an infinite reservoir), would the continuity equation still hold? In other words, would the flow rate increase? $\endgroup$ Commented Jun 16 at 22:25
-
$\begingroup$ @procommania Yes the continuity equation will still hold. Because when you start the pump it will increase flow rate of both incoming and outgoing water from pump. Hence if you are thinking that incoming water's velocity is less and than the outgoing water has more speed that its wrong. This is because if pump increase the outgoing water's velocity, then same amount of water is needs to enter in same amount of time to continue the flow. Hence considering uniform pipe, $Av_1=Av_2$ gives $v_1=v_2$. Reference image $\endgroup$ Commented Jun 19 at 7:29
