Mirror Equation for convex mirror

Question

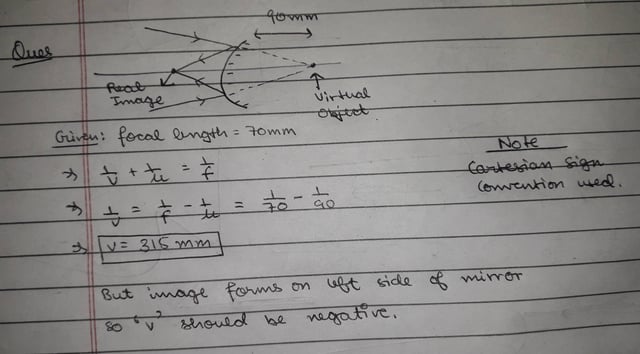
Question:
I have a convex lens with focal length $\mathrm{70mm}$ and it's forming a real image. We have a virtual object at $\mathrm{90mm}$ to the right of the mirror. Using the mirror equation gives the wrong solution.

Image for clarification:

My attempt:

1. $v$ (object distance)=$\mathrm{+90mm}$
2. $u$ (image distance)= unknown
3. $f$ (focal length)=$\mathrm{+70mm}$

Using mirror formula, $\frac{1}{f}=\frac{1}{u}+\frac{1}{v}$ I get $v$=$\mathrm{+135mm}$

Problem:
I was expecting $v$ to come with a negative sign since image formed on left side of mirror.

PS:

1. I am using Cartesian convention (direction of incident light taken as positive and other as negative).
2. The question is about why using the virtual object messes up answer from mirror equation.
3. I am aware that other convention also exists (in which $f$ for convex lens is negative, however this convention is the one i need to know where it goes wrong).
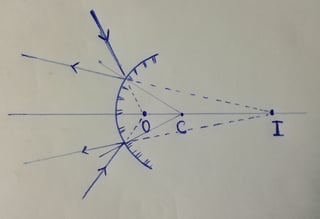
4. [Edit After @Infinite suggested that the diagram May be wrong] The diagram actually comes from the secondary mirror in a cassegrain telescope (like here).Though I think that if both the ray diagram and the calculation is correct and my use of mirror equation is correct then the only thing left is the data itself (70mm,etc),which is incorrect. But to conclude that I need to know that I correctly used the mirror equation here.

Answer 1

What you have done is correct. We have to judge the diagram by obtaining the results from the formula but not just simply imagining a diagram. The results suggest that your diagram was wrong and the correct diagram could be the below one:

---

Extending the comments:
To get a real image the virtual object should lie in between pole and the focus. We can prove this result from mirror formula. From the sign conventions, we have $f>0$ ,$u>0$ and $v$ should be less than zero (for forming real image).
So from mirror formula, $ \frac{1}{v}=\frac{1}{f}-\frac{1}{u} <0$ $\implies u<f$