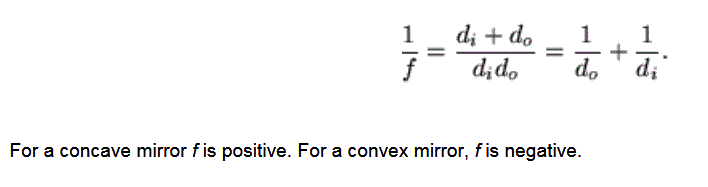Can a convex mirror form a real image?

Question

Is it possible to arrange a setup in which a convex mirror forms a real image(i.e an image that can be obtained on a a screen.)

Imagine a setup in which light from infinity falls on a concave mirror and the mirror converges it on the focus.(f would be negative)

However, if we put a convex mirror before the focus of the concave mirror,then the light rays will not actually meet, and the focus of the concave mirror(where the light would have normally converged if we had not placed a convex mirror) will act as a virtual object for the convex mirror.

In this situation, u (object distance) is positive and and so is the focal length of the convex mirror.

From the mirror equation, v=f*u/u-f For this value to be negative,f>u(where f if focal length of convex mirror) so that a real image is formed. But can this be practically done in the lab?How can we choose different sizes of mirrors and screen so that they do not block the light rays? I tried doing it but it did not work out. Can someone help and maybe suggest another setup.

Answer 1

Any discussion of concave/convex mirrors needs to begin with a statement of the particular version of the mirror equation to be used, along with the convention for setting and interpreting the signs of focal lengths, and object/image positions.

For example, from http://scienceworld.wolfram.com/physics/MirrorFormula.html:

Unmentioned in this is the convention that virtual images and objects are found behind the mirror and have negative values of $d$.

In the particular example you present, the image formed by the primary mirror is a real image. If you put infinity for the object distance and a positive focal length, you find a positive image distance.

But when you insert a convex mirror, with a negative focal length, into the optical path, you must also consider the position of the real image (now an object) relative to the convex mirror. The object is behind the convex mirror; it is a virtual object, and its distance from the convex mirror is negative.

With appropriate positioning of the convex mirror, the formula will produce a positive value for the image distance. There will be real image formed in front of the convex mirror.

You've just designed a Cassegrain telescope...