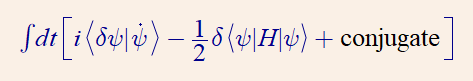I'm reading a text which discusses time dependent variation principle (Geometry of the Time-Dependent Variational Principle in Quantum Mechanics by Kramer and Saraceno), and there is some part of a derivation which I cannot understand. I'm trying to do a similar derivation in a slightly different context, so if anyone here could shed some light on what I don't understand, that would be really helpful.
This is the relevant part:
Up to the integration by parts I understand everything, so everything that happens up until then you can take as given. They then write "therefore, up to some total derivatives, we obtain...".
But I do not understand why this is what they obtain. When I plug in the integration by parts (and ignore the total derivatives, since they won't affect the solution), I get something slightly different:
Note that I know the origin for one difference - for my case I am taking $\langle\psi | \psi\rangle=1$, and so the term which depends on $\langle\delta\psi | \psi\rangle$ vanishes (and that makes sense, I'm fine with it). But the Hamiltonian term seems to be different than what they get and I am not sure why.
Moreover, I am not sure how they made the step that leads to equation $(2.3)$ (with this treatment of the bra and ket as independent variables). Does anyone understand how they did it?
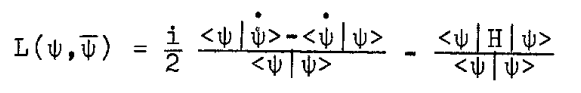
By the way, just for completeness, this is the relevant Lagrangian:
If anything here needs further clarification, let me know.