Does this system violate the second law of thermodynamics?

Question

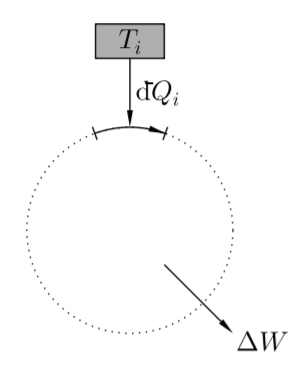
Consider a system (Blundell and Blundell 2nd edition, page 135) where a cycle consists of connecting each point to a reservoir at temperature $T_i$ and a heat amount $\delta Q_i$ enters that point. A whole cycle results in a work output $\Delta W$, which, since over a cycle $\Delta U = 0$, by the First Law, is given by: $$\Delta W = \sum_{cycle} \delta Q_i$$ However, Kelvin's statement of the Second law states that no process can have the full conversion of heat into work as a unique result. How is this not happening here? There is no colder reservoir so it looks to me like all the individual heat ammounts are fully converted into work.

Answer 1

I quote from the text on p. 134:

[W]e would like to generalize our treatment so that it can be applied to a general cycle operating between a whole series of reservoirs...Our general cycle is illustrated in Fig. 13.10(a). [emph. added]

The index $i$ indicates that multiple reservoirs at temperature $T_i$ are being used, each delivering infinitesimal heat $\text{đ}Q_i$. We conclude that some values of $\text{đ}Q_i$ must be negative to avoid requiring entropy to disappear, which—as you note—is forbidden by the Second Law.