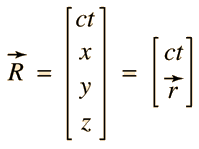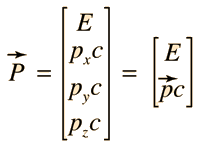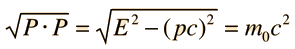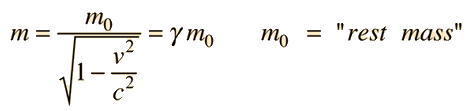Can an object theoretically reach, or exceed the speed of light? [duplicate]

Question

I'm sorry if this is a naïve question, but it's been bugging me for a bit. If a spacecraft were traveling in a perfect space with unlimited fuel, could it not, assuming it is not influenced by other objects in space, continuously accelerate until it reaches or exceeds the speed of light?

Answer 1

No it couldn't. One way to see this is to use the equation for kinetic energy, $E_k$, of a body of (invariant) mass $m$, as derived from the Special Theory of Relativity... $$E_k=(\gamma - 1)mc^2\ \ \ \ \ \ \ \text{in which}\ \ \ \ \ \ \ \gamma=\frac 1{\sqrt{1-u^2/c^2}}$$ It's easy to see that $E_k$ approaches infinity as the speed, $u$, approaches the speed of light, $c$, so however much fuel you burn to accelerate the rocket, you can never give it enough kinetic energy to reach the speed of light.

[Note that the above equation for $E_k$ boils down to the familiar newtonian equation, $E_k =\tfrac 12 m u^2$, when $u \ll c$.]

Answer 2

Let me concentrate on the part of the question about the accelerating spacecraft with the idealized engine (not running out of fuel). So imagine that you are sitting on this spaceship. The engine is running constantly at the same rate, nothing changes at all.

So imagine that the spacecraft is standing still. Then the engine is started and you have an acceleration, i.e. \begin{equation} v|_{t=0}=t_0,\quad \frac{dv}{dt}|_{t=t_0}=a \end{equation}

Let spacecraft move in the same direction it is accelerating. You can move into the inertial reference frame moving with the same velocity. Then again, in that reference frame the spacecraft at this specific moment will have zero velocity but nonzero acceleration, \begin{equation} \tilde{v}|_{\tilde{t}=\tilde{t}_0}=0,\quad \frac{d\tilde{v}}{d\tilde{t}}|_{\tilde{t}=\tilde{t}_0}=a \end{equation} We will call the acceleration as measured in this reference frame the proper acceleration. The situation with our spaceship as described above when nothing changes from the point of view of the passenger on the spaceship corresonds to the constant proper accelaration.

What will be such motion with the constant proper acceleration look like from the initial stationary reference frame? You will find that, \begin{equation} \frac{d}{dt}\Big(\frac{v}{\sqrt{1-\frac{v^2}{c^2}}}\Big)=a \end{equation} Which can be solved yielding, \begin{equation} v=\frac{at}{\sqrt{1+\frac{a^2t^2}{c^2}}} \end{equation}

For small $t$ you get the usual non-relativistic acceleration, \begin{equation} t\ll \frac{c}{a},\quad v\simeq at \end{equation} but when the velocity approaches $c$ it stops growing. For very large times you get, \begin{equation} t\gg \frac{c}{a},\quad v\simeq c\Big(1-\frac{c^2}{2a^2t^2}\Big) \end{equation} The velocity approaches $c$ asymptotically but never reaches it.

If you consider changing proper acceleration you fill find that you still can't reach $c$ let alone exceed it.

I would like to stress that from the point of view on the spaceship nothing special happens. In fact when the spaceship will reach $0.99c$ and you jump outside and thus will be moving afterwards inertially, from you point of view the spaceship at first (until its relative velocity will not grow enough) will move according to the non-relativistic law $v\simeq a t$.

Answer 3

Moving close to the speed of light needs the algebra of special relativity, i.e objects are no longer described as three dimensional vectors with time as a parameter, but time becomes also part of the variables in the special relativity four vector space.

where p is the old three vector momentum and E the energy of the object.

Each object is described by such a four vector and the "length" of the four vector is the invariant mass of the object.

When a massive object is in motion, a spaceship for instance, in order to increase its velocity energy must be supplied, according to the Lorentz algebra.

here $m_0$ is the invariant mass of the object in its rest frame, and $m$ is the relativistic mass , the classical mechanics, inertial mass that appears in the formula $F=ma$

One sees from the formula that the relativistic mass becomes infinite if the object can gain the velocity c. Thus it is only zero mass particles that can have the limit of velocity c.

As the Lorentz transformations have been extensively tested with particle physics and with calculations for observations in astrophysics our present theories accept that only zero mass particles travel with velocity c. All massive objects have to stay below.