A photon travels in a vacuum from A to B to C. From the point of view of the photon, are A, B, and C at the same location in space and time? [duplicate]

Question

So, for a photon, everything just is ?

Answer 1

As other answers have pointed out, there is no point of view or frame of reference that keeps up with a photon. Never the less, the idea that such a frame of reference exists as the limit of infinite boosts is a very natural one that comes up over and over. Here is why there are problems with that idea.

Suppose you start at rest in a certain frame of reference, and accelerate at $1$ g for $1$ sec. This give you a new speed. Do this again and again.

As you travel faster and faster an observer in your starting frame sees you traveling closer and closer to the speed of light, your clock running slower and slower, and your ruler getting shorter and shorter. The limit of these measurements is you traveling at the speed of light, your clock stopped, and your ruler contracted to $0$ length.

It is natural, but wrong, to suppose that at this point your frame of reference is the same as a photon. Therefore photons experience no time, and see the entire universe as contracted to a plane.

---

First, the limit state doesn't match what we see when we observe photons.

Photons travel at a finite speed. As they advance, they change phase. So the idea that they are in a frame where no time passes and all points along their path have been compressed into the same point is wrong.

---

Second, you don't get closer to traveling as fast as a photon.

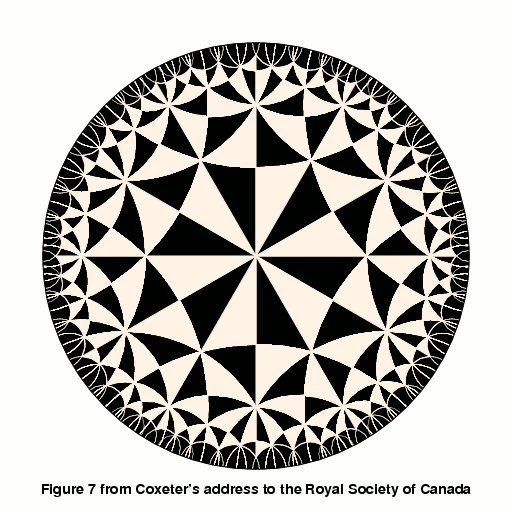
This can be illustrated by a hyperbolic tesselation of the plane. The tesselation below uses 30, 45, 90 degree triangles. It became famous when Escher used it as the basis of his Circle Limit woodcuts. In this post, it represents a $2D$ velocity space.

An observer is stationary in his reference frame. This velocity is the center point. The sides of the triangles represent boosts in various directions.

As you undergo boost after boost, the observer sees you travel faster and faster. Your velocity is a point farther and farther from the center of the circle. But each boost gives a smaller change to your velocity. You never reach the edge, which represents the speed of light.

After each boost, you can measure the speed of a photon. Each time, it is still passing you at the speed of light. You are no closer to its speed.

---

This video shows how velocity space appears to you as you try to accelerate to the speed of light. Or equivalently, try to reach the edge of velocity space. (It isn't quite the same tesselation, and the path isn't quite a straight line. But it gives the idea.)

All the triangles are the same, though the ones far away appear distorted. These triangles become normal as you approach them.

No matter how many boosts you undergo, you are still at rest in your own frame. From your point of view, you are in the center of the circle. You are a finite number of boosts from the observer and an infinite number from the circle. No part of the circle has become any closer or farther from you.

As you move from triangle to triangle, you stay at rest in your own frame, though you move far away from the observer. You see each boost as making the same change in velocity. Though the cummulative effect on the velocity of the observer gets smaller and smaller. The observer recedes at close to the speed of light, but never reaches it.

From your point of view, the observer is getting closer to a state where his ruler shrinks to $0$ and his clock stops. You might think the observer is closer to matching speed with a photon you send in his direction.

The observer thinks no such thing. He sees your photons arriving at the same speed as always, though they are increasingly red shifted.

---

Mathematically, you are advancing from 1 boost to 2 boosts to 3 boosts, etc. The limit of this sequence is an infinite number of boosts. This really means the sequence diverges and there is no limit. The definition of an infinite limit is that given any finite number, after enough steps you will pass that number. The limit is not a state where you are sitting on a point named infinity.

This means given any speed slower than light, after enough boosts you will be going faster than that speed. But there is never a state where you are going the speed of light.

If you try to construct a limit, it would go something like this: For any $\epsilon > 0$, there is a point $P$ in velocity space where you would measure the velocity as $v_p$ such that ($c - v_p) < \epsilon$. But an observer at $P$ would see a photon pass him at $c$. So the limit as "$P \rightarrow $ the edge" is a state where the observer at P sees a photon pass him at $c$. This really means at all large boosts, an observer at $P$ sees a photon pass him at $c$.

The separation between the interior of the circle and the edge is absolute. All points in the interior of the circle are an infinite number of boosts from the edge. No number of boosts brings an observer from the interior to the edge. Likewise, it never brings a photon from the edge into the interior.

---

Some references on the mathematics of the tesselation, and how Escher's woodcuts illustrate it:

• TheFamilyof“CircleLimitIII”EscherPatterns
• How Did Escher Do It?
• Escher and Coxeter – A Mathematical Conversation

---

Edit - I have updated this answer to make it clearer.

In the meantime, the question has been closed as a duplicate. Site policy is to put all answers under one question and not encourage duplicate questions. So I have copied this answer to How does a photon experience space and time?

Answer 2

The Minkowskian geometry of spacetime has a notion of observer-independent “distance” between points that is very different from the Euclidean notion of distance. In Euclidean geometry, two points with zero distance between them are the same point. In Minkowskian geometry, two points with zero spacetime distance between them can be different points in spacetime.

Mathematically, this is because the spacetime metric

$$ds^2=dx^2+dy^2+dz^2-c^2dt^2$$

is not a positive-definite metric like the Euclidean metric

$$ds^2=dx^2+dy^2+dz^2$$

is.

All of the spacetime points along a photon’s path (A, B, C, etc.) are different points. But these points have zero spacetime distance between them. Furthermore, this distance is zero in all Lorentz frames.

Answer 3

The photon doesn't have a point-of-view. There are no inertial frames that are light like, and there is really nothing to be gained by saying "what-if?".

If you put the origin of your coordinates at $B$, and line the $z$-axis with the photon's momentum, then the three events are (with c=1):

$$ [(-A,0,0,-A), (0,0,0,0), (C,0,0,C)]$$

where $A>0$ and $C>0$.

The best you can do is boost along the $z$-axis with speed $\beta$. In this frame, the three events are:

$$ [(-fA,0,0,-fA), (0,0,0,0), (fC,0,0,fC)]$$

where:

$$ f = \gamma(1-\beta) = \frac{1-\beta}{\sqrt{1-\beta^2}} = \sqrt{\frac{1-\beta}{1+\beta}}$$

turns out to be the relativistic Doppler shift factor.

This can be rewritten as:

$$ [(-A',0,0,-A'), (0,0,0,0), (C',0,0,C')]$$

Though you can make $f\rightarrow 0$ as $\beta \rightarrow 1$, nothing has really changed (since $A$ and $C$ are arbitrary).

Answer 4

As noted in other answers, the "point of view of the photon" is not meaningful because there is no Lorentz reference frame in which the photon is at rest. However, a different but related question can be answered: How are points A, B, and C on the photon's trajectory related in the geometry of spacetime? The intuition that they are in some sense "at the same location" may come from the fact that the Minkowski metric gives a "distance" of zero between them (lightlike separation, zero lapse of proper time). However, we should be clear that this does not imply "sameness", because:

• The Minkowski metric is indefinite, and so zero "distance" does not imply sameness as it does with a definite metric (e.g., in Euclidean space). The separateness of points A, B, and C in spacetime is inherent at the level of topology, which is logically prior to any metric. A small "neighborhood" of A, for example, includes points that are "close" to A in a purely topological sense, and can be chosen so that B and C lie outside this neighborhood. Such neighborhoods can be defined by "distance" using a definite metric, but not an indefinite metric; in the latter case, they are still well-defined (and "look like" arbitrarily squashed Euclidean balls), but are not metrical.

• Points A, B, and C have a quantifiable relation beyond being distinct: They can be indexed by the affine parameter along the photon's lightlike (null) geodesic. Thus, we can say (for example) that B is 1/3 of the way from A to C. This affine parameterization (like the notion of geodesic itself) relies only on defining parallel transport in spacetime, which is more structure than topology but still less than a metric. So again, it works for distinguishing A, B, and C because it doesn't try to use metrical "distance".

Answer 5

From the point of view of the photon, are A, B, and C at the same location in space and time?

...

So, for a photon, everything just is ?

The question is (in a sense) unanswerable since there is no such thing as "the point of view" of the photon.

But, perhaps in a "meta" sense it is answerable, since if it is unanswerable then what is the point of this answer I'm writing?

This answer is really just providing an example in an attempt to help ease the mental itch one feels when one does not want to believe that there is no such thing as "the point of view of the photon."

Anyways.

For concreteness consider just two specific events (Event A and Event B) as observed by a physical observer (say, a scientist on earth): Event A is a photon passes the location $x_A$ at time $t_A$ (I'll take the x axis as the direction of travel of the photon); Event B is the photon is as location $x_B$ at time $t_B$.

Since, in this specific example, it is a photon being observed we have the relationship: $$ c = \frac{x_B - x_A}{t_B - t_A} \equiv \frac{\Delta x}{\Delta t}\;. $$

Suppose now that different physical observer is observing these same events, but now from a frame that is moving with velocity $v$ in the x-axis direction. This different observer's measurements of the time and space intervals for Event A and Event B are related to the first observers measurements by a Lorenz transformation as: $$ \Delta \tilde t = \gamma \Delta t(1-\frac{v}{c}) = \Delta t \sqrt{\frac{1-\frac{v}{c}}{1+\frac{v}{c}}} $$ and $$ \Delta \tilde x = c\Delta t\sqrt{\frac{1-\frac{v}{c}}{1+\frac{v}{c}}}\;. $$

Where, again, the specific case we are considering is the observation (by two different observers in different inertial reference frames) of a photon traveling from $x_A$ to $x_B$.

Unfortunately, regardless of how hard a physical observer tries (how close $v$ is to $c$ from one frame to the other), they will never "catch up to" the photon. In other words, regardless of which observer's frame we are in, the speed of the photon is $c$: $$ \frac{\Delta \tilde x}{\Delta \tilde t}=\frac{c\Delta t\sqrt{\frac{1-\frac{v}{c}}{1+\frac{v}{c}}}}{\Delta t \sqrt{\frac{1-\frac{v}{c}}{1+\frac{v}{c}}}} = c = \frac{\Delta x}{\Delta t}\;. $$

Now, you might look at my equation for $\Delta \tilde x$ and for $\Delta \tilde t$ and say "Hmm, if I set $v$ equal to $c$ in those equations then there is no distance and no time between the two events." But, of course, the Lorenz transformation matrices are infinite at $v=c$ so this approach is frowned upon.

Better yet you might say "Hmm, as $v$ approaches $c$ from below both $\Delta \tilde x$ and $\Delta \tilde t$ approach zero. But, although both $\Delta \tilde x$ and $\Delta \tilde t$ approach zero their ratio is always fixed (and equal to $c$ since it is a photon being observed).

In other words, any physical observer will always be travelling less than $c$ and any physical observer will always see the photon's speed as $c$.

If we assume that only a physical observer can have a "point of view" then it is clear that a physical observer can't have the "point of view" of a photon. Of course, I could have just said this without writing any equations. The equations are just there to provide an example of how mixing around the various symbols in equations and then trying to interpret them out of context can lead to what is likely unknowable nonsense.

I think the most reasonable approach is to just accept that there is no "point of view" of a photon. But regardless of what you choose to say, there is nothing "deep" here. The "point of view of a photon" doesn't have any meaning other than what you might give it by going through some hand-waving rigmarole similar to the above. Furthermore, there will likely be no progress made by thinking about "the point of view of a photon"--it is likely a pretty useless concept and as such better to spend your time elsewhere.

One final point you may want to consider is that it is possible (and easy) to ask unanswerable nonsense questions. We can not stop you from stringing words together into sentences that look syntactically like well formed questions, but are semantic nonsense. For example: "What is the square root of a watermelon?" This is a syntactically proper sentence with a question mark at the end... but it is nonsense. These kind of questions tend to not lead to anything very useful.

Answer 6

This is one of those cases where it is important to remember that photons are a mathematical construct that is useful to explain/predict observed phenomena.