How does light behave within a black hole's event horizon?

Question

If the event horizon of a black hole is the distance from the center from within which light cannot escape, imagine a person with a flashlight falls into the black hole.

He points his flashlight in a precisely radial direction and turns it on. Now there is a light ray moving outward at the speed of light. If it now cannot move in an arc, but rather is constrained to radial motion, it must, at some point before the horizon, switch directions and fall back into the black hole.

If the speed of light is constant, how does it suddenly change directions, without either decelerating, or requiring an infinite amount of energy?

Answer 1

One of the problems with describing any situation in general relativity is choosing an appropriate set of coordinates. Far from the black hole we use the Schwarzschild coordinates $t$ and $r$ (we'll ignore the angular coordinates). These are just the radial distance as measured with your rulers and the time measured on your clock so they have a nice simple interpretation. The trouble is that as you approach the event horizon the time gets dilated by a factor:

$$ \frac{d\tau}{dt} = 1-\frac{2M}{r} $$

and at the event horizon, where $r = 2M$, this factor goes to zero. This means time slows to a stop at the event horizon, and it's the source of the common claim that nothing can ever cross the event horizon.

Obviously we're going to struggle to describe what happens to light from a torch inside the event horizon if it takes an infinite time for the torch to even reach the event horizon, let alone cross it. So we need to look for a better coordinate system. We could try using the coordinate system of the astronaut falling in. The trouble with this is that for any freely falling observer spacetime is locally flat, so (ignoring tidal forces) the astronaut thinks they are motionless in flat space. When they turn on the torch the light just speeds off at $c$ as usual.

Outside the event horizon we can use shell observers i.e. observers hovering at a fized distance from the horizon. The trouble is that inside the event horizon it's impossible to hover at fixed $r$, so we can't use shell coordinates either.

So what to do? Well in cases like this we have to choose a set of coordinates that don't correspond directly to anything seen by an observer. This allows us to describe what happens inside the horizon, but at the expense of simplicity. In particular it becomes hard to match the description with our intuitive feel for what happens. Sadly this is a price we have to pay.

The very best coordinates to use are the Kruskal-Szekeres coordinates $u$ and $v$ because they make the causal structure immediately obvious. However these are prohibitively complicated for the non-specialist. The coordinate $u$ is spacelike but isn't simply radial distance, while the coordinate $v$ is timelike but isn't simply time. So I'm not going to use the KS coordinates to answer this question. However, if you're feeling brave have a look at my answers to Would the inside of a black hole be like a giant mirror? and Taking selfies while falling, would you be able to notice a horizon before hitting a singularity? where I use the KS coordinates to answer related questions.

In this case I'm going to use the Gullstrand-Painlevé coordinates. sometimes known as rainfall coordinates or the river model. In these coordinates $r$ is the same radial distance as in the Schwarzschild coordinates, so that's easy to understand. However the time coordinate $t_r$ is the time recorded by a clock carried by a freely falling observer, and because of the time dilation mentioned above this is not the same as the time recorded by the Schwarzschild observer far from the event horizon. Bear this in mind as you consider what follows.

I've already used the GP coordinates to calculate the speed of light heading to or from the black hole in my answer to Why is a black hole black?. The result is:

$$ \frac{dr}{dt_r} = -\sqrt{\frac{2M}{r}} \pm 1 \tag{1} $$

where the $+$ gives the speed of an outgoing ray and the $-$ gives the speed of an ingoing ray. Note that this uses geometric units where $c = 1$. In these units the event horizon is at $r_s = 2GM$. If we use equation (1) to calculate the speed of an outgoing light ray at the event horizon $r = 2M$ we get:

$$ \frac{dr}{dt_r} = -\sqrt{\frac{2M}{2M}} + 1 = 0 $$

and we find that at the event horizon a light ray doesn't shine out, stop and fall back. Instead its velocity is zero so it is fixed motionless and doesn't go anywhere. Inside the event horizon, where $r < 2M $, the velocity of an outgoing ray is negative. So inside the horizon even a light ray directed outwards actually moves inwards not outwards. This is the key result we need to answer the question.

Admittedly we're using an odd time coordinate, but the $r$ coordinate is our good old Schwarzschild coordinate. So while we may may quibble about the exact value of the calculated velocity the sign is unambiguous. That means when our falling astronaut shines his torch outwards the light does not move out, come to a halt and fall back again. The light is moving inwards from the moment it leaves the torch. The reason the astronaut sees the light move away is because the astronaut is falling inwards even faster than the light.

A comment asks if this means the astronaut is moving faster than light, and yes it does. However this shouldn't surprise you as in GR it's only the local velocity of light that is constant at $c$. At distant locations light can move faster or slower than $c$ (though we'll never observe it moving faster as a horizon will get in the way). For example it's well known (or should be!) that sufficiently distant galaxies are moving faster than light.

There is one last loose end to tie up. I claimed above that the astronaut sees the light move away because the astronaut is falling inwards faster than the light is. Can we prove this? It's actually fairly easy to prove if we start from the well known result that the velocity of an observer falling freely from infinity is (in Schwarzschild coordinates):

$$ \frac{dr}{dt} = -\left( 1 - \frac{2M}{r} \right)\sqrt{\frac{2M}{r}} $$

To convert this to the Gullstrand-Painlevé coordinates we note that the rainfall time $t_r$ is just the proper time $\tau$ along the trajectory of the infalling astronaut, and the proper time is related to the coordinate time by the expression I gave above:

$$ \frac{d\tau}{dt} = 1-\frac{2M}{r} $$

The velocity of the astronaut in GP coordinates is then simply:

$$ \frac{dr}{dt_r} = \frac{dr}{dt_r}\frac{d\tau}{dt} = = -\frac{\left( 1 - \frac{2M}{r} \right)\sqrt{\frac{2M}{r}}}{1-\frac{2M}{r}} = -\sqrt{\frac{2M}{r}} $$

Compare this with equation (1) for the velocity of the light, and you'll see that the velocity of light differs from the velocity of the astronaut by $1$. So the light is always moving at a velocity of $c$ relative to the astronaut.

Answer 2

There are many extra subtle effects that you neglect – the red shift, perhaps the Hawking radiation that makes the black hole shrink, and so forth (one could recommend you to learn the Penrose causal diagrams) – but if one tries to be cooperative, he must say that it is indeed the case that some "velocity of the light from the flashlight" expressed in appropriate variables indeed switches the sign when the flashlight crosses the horizon.

For the neutral black holes, this is manifest in the Schwarzschild coordinates where the metric is $$ c^2 {d \tau}^{2} = \left(1 - \frac{r_s}{r} \right) c^2 dt^2 - \left(1-\frac{r_s}{r}\right)^{-1} dr^2 - r^2 \left(d\theta^2 + \sin^2\theta \, d\varphi^2\right)$$ Light has to propagate to the future (no one can travel in the past) and indeed, in these coordinates it means $dt\gt 0$. And it must propagate over null geodesics which means $$c\,dt (1-\frac{r_s}{r}) = dr $$ for $ds^2$ to vanish. The angular variables contribute nothing.

You see that $dr/dt$ which is a sort of "coordinate velocity", measuring how much the radial coordinate $r$ changes as a function of the Schwarzschild time $t$, is equal to $c(1-r_s/r)$ and indeed, as we switch from $r\gt r_s$ to $r\lt r_s$, this quantity $dr/dt$ switches the sign ($dr/dt$ is equal to zero when the light is just crossing the event horizon – well, such light is "confined" at a fixed value of $r$).

I must emphasize that this sign flip is an artifact of the chosen (Schwarzschild) coordinates. There exist other coordinates that, in the vicinity of the event horizon of a large black hole and in the reference frame of an infalling observer or the flashlight, resemble the Minkowski space. In these coordinates, the light always propagates along $x=ct$ trajectories with a fixed slope and sign. And in these coordinates, the event horizon is a plane that is moving by approximately the speed of light in the "outward" direction (the event horizon is not static in these coordinates!) which is the explanation in these coordinates why the flashlight's light can't catch up with the event horizon.

One may choose many different coordinates and they may have advantages. A black hole is a static object so one may choose coordinates in which the metric tensor is independent of time $t$; Schwarzschild coordinates are an example. And one may choose coordinates that describe the vicinity of the event horizon "smoothly", without singularities and confusing sign flips of the velocity. But there are no coordinates that would have both properties at the same moment.

Answer 3

Now there is a light ray moving outward at the speed of light.

I'm afraid that isn't the case; within the event horizon of a Schwarzschild black hole, the radial coordinate is timelike and so, moving 'outward' toward the horizon is as impossible as moving 'backward' in time.

This plain to see in the Kruskal–Szekeres coordinates:

Image credit

See that, considering the light cone shown inside the black hole, even light emitted in the direction of the horizon always gets closer to the singularity (the $r=0$ hyperbola at the top of the diagram), eventually reaching it, and never gets closer to the horizon (the $r=2M$ line through the origin).

Answer 4

First, the speed of light, as measured by a local observer, is always the same, that is $c$.

To correctly pose your problem, you have to use a light-cone version of Kruskal Szekeres coordinates

The metric is given by :

$ds^2 = F(r) dU dV + r^2 d\Omega^2$, where F(r) is some function of r

The black hole interior is given by $U > 0$ and $V > 0$

The outgoing null geodesics are given by $U = Cte$ (with $V$ increasing).

The ingoing null geodesics are given by $V= Cte$. (with $U$ increasing).

The future horizon is given by $U = 0$ and $V > 0$.

The future singularity is in $UV = 1$ with $U > 0$ and $V > 0$.

So, now, imagine, you are in the interior of the black hole, that is $U > 0$ and $V > 0$, you are sending a outgoing radial light signal, but this signal is at $U= Cte$, so the variable $U$ stays $>0$. But the future horizon is $U = 0$ and $V >0$. So your outgoing signal never reachs the (future) horizon, because the value $U=0$ is never reached.

It is better to draw a litte diagram with the coordinates U and V orthogonal, With upwardly directed axes, and with U and V making an angle or 45 degrees from the vertical.

To complete the schema, you have also :

The past singularity is in $UV = 1$ with $U < 0$ and $V < 0$.

The past horizon is given by $V = 0$ and $U < 0$.

Answer 5

I think a possible analogy would be to imagine that the singularity is a waterfall. By emitting light, you are trying to send a signal upstream using a tame fish. Outside the event horizon the fish is able to make headway against the current. But the river flows so fast within the event horizon as it approaches the waterfall, that your fish ends up going over the waterfall shortly after you do because the river is flowing faster than the fish can swim. Note that at no point does the fish change direction and it moves with respect to you at "the speed of fish".

This "river model" for a black hole is discussed in detail by Hamilton & Lisle (2006).

Answer 6

For better clarity, let's define the following:
Axial direction = the direction the person & light beam are drawn into the BH.
Radial direction = the direction perpendicular to the axial direction.

If we, looking in the same direction as the person & light are being drawn into the BH, watch the light beam as it is drawn into the BH, we will see the following:

1. Whatever reflected light the beam may produce (i.e. due to gas, debris, etc) that it collides with, as it approaches the event horizon. (This assumes we start at a point in which we can still see the reflected light from the light beam).

2. The force of the light beam will not travel beyond the outer edge of the BH (at some point) as it is drawn further into the BH.

3. The person & the light beam would disappear from our view as it passed the event horizon (much like a ship going beyond the horizon at sea).

4. If we change our pov (& acceleration) so that we could look into the light beam as it is drawn into the BH (like a camera that follows a sprinter on a race track), we would see the light beam widen in the axial direction (i.e. parallel to the direction of the moving person) as the forces of the BH overcome the forces of the light beam & distort/weaken it. I think the light would just blink out (from our perspective) when it got too far into the BH.

re "If it now cannot move in an arc, but rather is constrained to radial motion, it must, at some point before the horizon, switch directions and fall back into the black hole."
--Or, perhaps it is just weakened to the point that it cannot exit beyond the BH.
Also, forces near the center of the BH are greater than forces near the rim of the black hole (i.e. like a vortex). Greater forces will affect the light more & lesser forces will affect the light less. So, that probably means the light near the center of the BH is bent/attenuated faster than light near the edge/rim of the BH.

re: "If the speed of light is constant, how does it suddenly change directions, without either decelerating, or requiring an infinite amount of energy?"
--There are forces in the universe greater than light. They don't necessarily need to be infinite to bend/slow, distort, or destroy the force of light.

Answer 7

speed is $$\frac{\text{distance}}{\text{time interval}}$$

but at the event horizon of a black hole, time interval becomes $0$.

Imagine a flashlight flashes periodically 1 flash/s (in flashlight's reference frame). As flashlight getting close to the event horizon, someone far away from the event horizon will see flashlight flashing $0.1$ flashes/s, $0.001$ flashes/s $0.00001$ flashes/s, and decreasing as it approaches at the event horizon.

The number of flashes per second is a time interval observed by us (people far away from the black hole).

So, our one second is flashlight's 0.00001 seconds.

Flashlight at the event horizon sees the light traveling at a speed of light (in vacuum) for 1 seconds (can be 10 seconds or $x$ seconds), we'll see a light travelling at a speed of light for 0 seconds.

From the formula:

$$ \text{speed} \times \text{time interval} = \text{distance} $$

:)

Although light has constant speed of $299,792,458$ [m/s] in a local inertial frame neglecting regarding elements (i.e., in vacuum), distance that light travels at the event horizon becomes 0 (it is travelling in a constant (and finite) speed though).

Light never decelerates, or changes direction. It travels straight "out" with respect to the person falling in, but straight "in" with respect to us, the observers outside the black hole (insofar as much as the relevant coordinate systems say) radially with a constant speed, yet we the external observers never observe it penetrating the event horizon, as if it had stopped there.

Isn't it weird?

Answer 8

I was searching for a duplicate question when I came across this one, it's not quite the same as my question but it seems useful.

I was going to ask: "If you shot an arrow at a black hole how long would it take to hit the surface", I thought it would make for an interesting question.

The difference with this question seems to be that you're the arrow and pointing a flashlight back to the starting line.

Let me start my answer by saying that I'm a long distance and time from being qualified to answer this question.

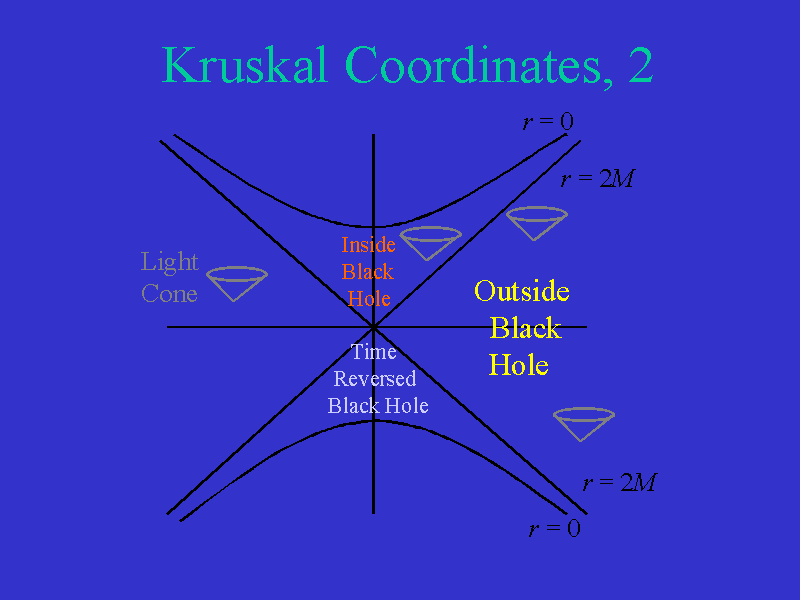
If the event horizon of a black hole is the distance from the center from within which light cannot escape, imagine a person with a flashlight falls into the black hole.

OK, so let's not concern ourselves with:

• The length of time you expect the batteries to last, so it's ∞
• Your starting speed relative to the black hole, so it's 0
• You say: "... cannot move in an arc ...", so it's like a Light Saber (not a watering hose)
• Your strength, so it's ∞ (you can't be flattened, or drop the heavy flashlight), unless you hit your head on a rock (the surface of the black hole); that causes you to lose all your strength (you'll see how that aids the answer later)
• Motion of the black hole through space, you could match it; nor should it spin as that would add angular motion (light would "arc", to use your terminology) and increase the gravity at the equator (and reduce it at the poles) due to the fusion mass's flattening

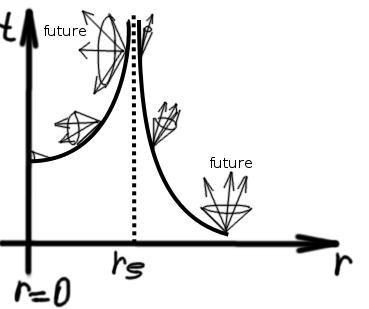
He points his flashlight in a precisely radial direction and turns it on. Now there is a light ray moving outward at the speed of light. If it now cannot move in an arc, but rather is constrained to radial motion, it must, at some point before the horizon, switch directions and fall back into the black hole.

Your assumption is that the light travels at the speed of light, that also assumes that inside the Event Horizon is a vacuum, it seems unlikely but let's go with that. I'll address slow light below.

Imagine the event horizon like it's an enormous plastic bubble, thinner than a soap bubble and immobile at absolute zero (very strong but you can penetrate it).

If the speed of light is constant, ...

The speed of light is constant, in a vacuum. Nothing says light can't travel slower.

See Lena Hau's video: "Prof. Lene Hau: Stopping light cold" where she creates slow light conditions, causing light to travel as slow as 17 meters per second and even stopping it completely.

There are theories about faster than light travel also. See source: notational speed greater than the speed of light, otherwise inside the event horizon would be brightly lit (maybe it is, don't ask me).

... how does it suddenly change directions, ...

Imagine on Earth throwing a rubber ball directly straight at a cement wall (slightly tilted away from you); it bounces back into your hand. [Apply appropriate conventional math.] Finished, next situation.

Now instead imagine you're traveling towards a black hole head first, pointing the flashlight between your feet. You're inches away from the event horizon and there's either no accretion disk or you're in a clear spot free of debris.

The moment before you touch the event horizon you and your flashlight are traveling at virtually the speed of light (minus a tiny bit)

[Remember: We are assuming that we are in a vacuum, which is unlikely.]

If you were not strong you would stretch and your feet would travel two little bits slower than the of light. Let's assume your head, flashlight and feet all travel at the same speed.

Now instead of you being like the rubber ball and bouncing off of the event horizon you penetrate it, like a bullet through a lightbulb.

If the event horizon were infinity flat (and so were you for an instant) you'd be traveling at the of light (if you're in a vacuum), and for an instant light wouldn't be emitted from the point source bulb of your flashlight (everything, including light, is constrained to one direction; it is: 'towards the black hole or bust').

While traveling through the event horizon, and a moment later, you'd be traveling at the of light.

The moment you cross the event horizon you are traveling the same direction (physically, from your point of view, from an observer's point of view it's like their eyeball is the black hole and the event horizon a mirror) you were a moment ago, away from the starting line.

You're back to normal length (because you're so strong). The only reason to flatten you for an instant is to define a specific location where head and feet both travel at the of light. [Bad math and gross oversimplification, OK. Since we're assuming that we're in a vacuum valid calculations were thrown out already.]

Think of it as a "mandatory direction", nothing can travel in the opposite direction you are going, your shoe can not possibly fall off, you can't drop your keys (or flashlight) and light (or anything) can only travel at an angle between: slightly greater than 90° (sideways), that would require tremendous energy, and straight towards the central point.

If you had a flashlight with a wide beam pointed sideways at 90° slightly more than half of the flashlight would be black (because light couldn't push it's way out) and the remaining slightly less than half of the emitting surface would have the light sucked downwards towards the central point, like the number 7 with a short too and rounded corner (like a question mark with a flat top like a number seven).

... without either decelerating, ...

Nothing, not even light, can travel with enough force to travel downward and importantly your direction (as seen / unseeable by an outside observer) doesn't change ...

You always travel towards the center of the black hole (we're assuming it's not spinning and that if it's moving you are matching it) at an increasing speed, passing the speed of light (slightly, due to the Gravitational Constant of the black hole) at the event horizon where it is space itself that is inverted.

You are like the rubber ball bouncing off a paper thin wall except the moment you touch the wall (to bounce) you are (assuming you're paper thin) on the opposite side of the wall and bouncing from it's opposite side (space directions are inverted top to bottom but your direction of travel is the same as a moment ago).

So there's no deceleration and no energy or effort on your part to pierce the event horizon.

... or requiring an infinite amount of energy?

It is direction itself which is opposite (East and West, or North and South, flip). It's like the Earth was far bigger, hollow, and gravity reversed; you fall towards the central point. It's as though the event horizon was pushing you and shrinking at your feet; but it's the black hole and it's gravitational constant pulling you in and slowly accelerating your speed.

So light from your flashlight no longer illuminates your feet they are black (we're stretching the truth, to assume you can see and think), light can either travel towards the 'battery end' of the flashlight or it is blocked; like a laser pointer placed tip down on a mirror. Nothing has enough energy to travel towards your feet.

It's like traveling through a "two-way mirror tinfoil ball", as you continue to penetrate the layers you can only see down if the photons from the object can catch up, and objects above your head can't emit light that can escape the gravity and hit your eyes.

If the light can't possibly be emitted (like if it were an LED flashlight) then it can go nowhere, possibly heating the chip; no matter. Flip the flashlight 180° (before you crash into the black hole's liquid fusing (fusioning) surface) and the light exits the flashlight at the speed of light plus the Gravitational Constant.

You next crash into the black hole's surface at the speed of light plus terminal velocity, no bounce or splash, and because you're so tiny in comparison and your space is compressed, so (virtually) no effect.

"Infinite" energy, speed, etc. is impossible; much like all the galaxies together don't have an infinite number of atoms.

There's no acceleration or deceleration exactly at the event horizon, the direction of space flips and your inertia travels the same direction as moments before.

[That's my oversimplied no-math explanation.].

Answer 9

As I know, Field Theory, that to what appeals the topic creator cannot explain the very powerful gravitation fields . So trying to understand what happens with a photon there are inside the Black Hole in meaning of Field Theory, or Special Relativity, isn't a good idea.

The Nature has no the alone space , and the alone time , you can abstractly image that, but there aren't created the pure space , or the pure time in the laboratory.

So why the speed of light is constant, how does it suddenly change directions, without either decelerating, or requiring an infinite amount of energy?

Photons don't change their directions, they moving straight on the geodesic lines of the space-time, and inside the Black Hole curvature of the spacetime is so huge that all of this lines, leads the way straight to the singularity.

Like ants on the apple (but imagine that the ants inside an apple and cannot break the border, moving fast as they can , but always traps in the center of the apple). They CANNOT get through the apple , and Photons cannot broke the spacetime and get trough the spacetime CONTINUUM.

You can image that, like hockey players cannot break the ice, and will moving straightforward on the surface of the ice, even if you will make the huge ice surface curvature! :) It will be not a good picture...
I think inside the Black Hole will be something like that r - radius from the Black Hole's center , t - local time , cone - light cone, $\Large{r_{s}}$ - Schwarzschild radius .

Photons have no mass (yeah , it's another hard thing to imagine but nevertheless), and you cannot do force to them in the classic meaning of that thing... :)

So light rays can't break the spacetime continium and they can't back in time! So only way for them trough the huge curvatury spacetime inside the Balck Hole leads to the future = to the singularity center.
You can read the good answer to your question in the page 43 of that book Black Holes - Lectures of Physics

Answer 10

How does light behave within a black hole's event horizon?

It doesn't behave at all.

If the event horizon of a black hole is the distance from the center from within which light cannot escape, imagine a person with a flashlight falls into the black hole.

I've explored this with a variety of relativists, and posed this question. The answer comes as a surprise to most people.

He points his flashlight in a precisely radial direction and turns it on. Now there is a light ray moving outward at the speed of light. If it now cannot move in an arc, but rather is constrained to radial motion, it must, at some point before the horizon, switch directions and fall back into the black hole.

This isn't true. At no point in the scenario does the light ever switch direction and fall back into the black hole.

If the speed of light is constant, how does it suddenly change directions, without either decelerating, or requiring an infinite amount of energy?

It isn't constant. See the Einstein digital papers. This dates from 1920:

Or see Irwin Shapiro talking about it in 1964:

The speed of light varies with gravitational potential. Light goes slower when it's lower. And what that means, is that the upward vertical light beam speeds up. I know that's not what you've been told, but I'm not making this up. See this PhysicsFAQ article for more. Now, take a look at Wikipedia. Note this: "The coordinate speed of light (both instantaneous and average) is slowed in the presence of gravitational fields". That ties in with what Einstein and Shapiro were saying, and Don Koks and others. Now pay attention to this: "at the event horizon of a black hole the coordinate speed of light is zero". The light doesn't get out because the coordinate speed of light, the speed of light as measured by distant observers, is zero. As your observer descends, the light coming from his torch emerges slower and slower and slower, until in the end, it doesn't emerge at all. That's why the black hole is black.

Notes:

Einstein dismissed Gullstrand-Painleve coordinates for good reason. A gravitational field is a place where a concentration of energy in the guise of a massive body has "conditioned" the surrounding space, altering its metrical properties, this being modelled as curved spacetime. But it's curved spacetime, not falling-down spacetime. The notion that we live in some Chicken-Little world where space is falling down is popscience pseudoscience.

IMHO Einstein would have similarly dimissed Kruskal-Szekeres coordinates. You'll be aware that at the event horizon gravitational time dilation goes infinite and a clock doesn't tick? What Kruskal-Szekeres coordinates do is effectively place a stopped observer in front of the stopped clock and claim that "in his frame" he sees the clock ticking normally. Even though he's at a place where the clock has stopped, and light has stopped. I'm afraid it's "nonsense in neverland". It's a dead parrot sketch.

As for redshift, an ascending photon doesn't lose energy. In similar vein a descending photon doesn't gain any. You know this because you know that conservation of energy applies: if you send a 511keV photon into a black hole, the black hole mass increases by 511lkeV/c². The redshifted photon is emitted at a lower frequency when the emitting body is at a lower elevation, it doesn't lose any energy as it ascends. As for Hawking radiation, after forty years we have no evidence. Which is hardly surprising since it ignores the infinite time dilation and relies upon virtual particles popping into existence even though they only exist in the mathematics of the model. And it relies on negative-energy particles. If you see any of those, make sure you put in a call to Stockholm. In addition and despite much mathematical handwaving, "a black hole is a static object", so the event horizon isn't moving at the speed of light.

Kevin Brown's mathspages article The Formation and Growth of Black Holes is worth a read. Pay attention to the frozen star interpretation. He doesn't rate it, but he wrote this article before the Einstein digital papers came online. IMHO this relatively unknown interpretation will eventually become the mainstream interpretation. It's just a matter of time.