Is a tangential acceleration, and thus an angular acceleration necessary for rotation to occur? If an object in a UCM rotates then would centripetal acceleration be sufficient to induce rotation? Then, why would the equation relating angular and linear acceleration not include a centripetal component? Is there no rotation if angular acceleration does not exist? I'm referring to an orbital rotation. I ask because I'm having a hard time understanding why only the tangential component of an applied torque causes rotation and that the radial component doesn't, when the radial component of an applied torque could induce an opposite reaction force with which your centripetal acceleration would be present, and wouldn't that centripetal acceleration then at least partly cause rotation if there is rotation in a UCM where only centripetal acceleration exists?
-
$\begingroup$ 'Centripetal acceleration' is not sufficient to induce angular motion. You need to have some initial tangential velocity as well. In fact, it is only after angular motion starts that we call it centripetal acceleration. Before this there's only linear acceleration as directed by the force field. $\endgroup$– Ambica GovindCommented Nov 4, 2021 at 5:29
2 Answers
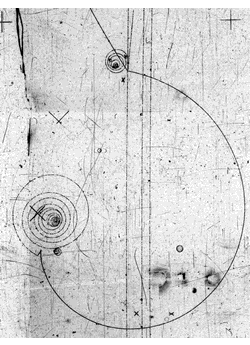
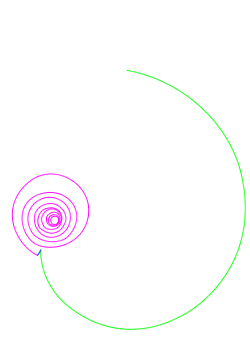
See this bubble chamber picture of charged particles moving in a perpendicular to the page magnetic field, to get an intuition.
All tracks entering the chamber from below , enter the magnetic field and are subject to the
constraint , centrifugal force equal to centripetal, so in the chamber they are parts of a circle.
The pi to mu to positron decay, seen on the right in the bubble chamber pictures, shows the effect of deceleration on the circular motion, which is due to the energy loss from ionizing the hydrogen in the bubble chamber. Instead of the end positron keeping a circular helix ( there is a component perpendicular to the field) the path diminishes.
An original ( $Δ(t)$)tangential acceleration is necessary for the start of circular motion, in the positron case , it is the decay of the muon that gives the initial momentum and the magnetic field the tangential impetus. Uniform circular motion should have occurred, if there were not the energy loss in the path.
Does an object performing a uniform circular motion rotate even when there is no angular acceleration?
An object can rotate uniformly (fixed radius) only if there is no acceleration or deceleration. Angular or linear acceleration/deceleration destroys the uniformity.
-
$\begingroup$ This is a nice answer but it seems a bit overkill wrt Is a tangential acceleration, and thus an angular acceleration necessary for rotation to occur? $\endgroup$– GertCommented Nov 4, 2021 at 7:14
-
$\begingroup$ I wanted to help the OP to get an intuition on how circular motion happens in experiment $\endgroup$– anna vCommented Nov 4, 2021 at 7:18
-
-
Is a tangential acceleration, and thus an angular acceleration necessary for rotation to occur?
Just look at Newton's First Law, here for rotation:
A body continues in its state of rest, or in uniform rotation, unless acted upon by a net torque.
A body acted upon by a net torque moves in such a manner that the time rate of change of angular momentum equals the net torque.
So let the torque about the axis of rotation be $\tau$ and $\omega$ the angular velocity and $\vec{L}$ the angular momentum, then:
If $\omega=0$ and $\tau=0$ then $\omega$ will remain zero.
If $\omega \neq 0$ and $ \tau=0$ then $\omega$ will not change in time.
But if $\omega=0$ OR $\omega \neq 0$ and $\tau \neq 0$ then:
$$\vec{\tau}=\frac{\mathrm{d}\vec{L}}{\mathrm{d}t}$$
If the inertial moment $I$ is time invariant we can simplify this to:
$$\vec{\tau}=\vec{\alpha} I$$
where:
$$\vec{\alpha}=\frac{\mathrm{d}\vec{\omega}}{\mathrm{d}t}$$