One-loop diagram calculation: Wick rotation and gamma matrices

Question

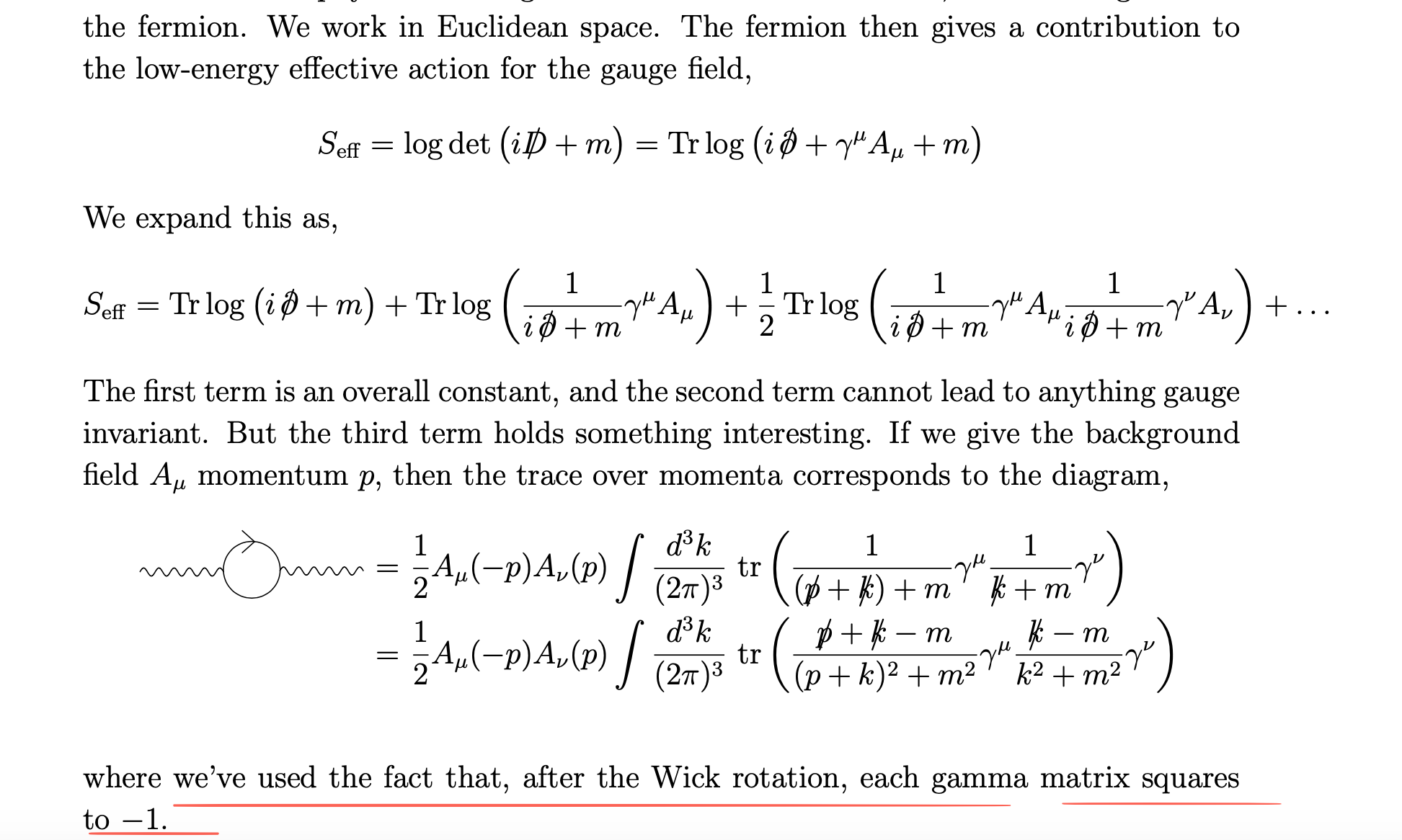
I have a question about a calculation which is performed in David Tong's lecture notes on Gauge Theory (page 400, chapter 8). At the bottom (see below for a screenshot), we want to calculate the one-loop diagram. He Wick rotated to Euclidean space. I don't understand how to obtain the second line from the first? When he rewrites the trace?

Also, given that $\left\{ \gamma^{\mu}, \gamma^{\nu} \right\} = 2 \eta^{\mu \nu}$ with $\eta^{\mu \nu} = (+1, -1, -1)$ (we are in $(2+1)$-dimensions), what does this become after Wick rotation? Does it become $\left\{ \gamma^{\mu}, \gamma^{\nu} \right\} = 2 \delta^{\mu \nu}$ with the standard Euclidean metric?

I was thinking of doing: $(\gamma^{\mu} k_{\mu} + m) (\gamma^{\nu} k_{\nu} - m) = k^2 - m^2$ (this is true in Lorentzian signature, not sure if after Wick rotation). From this it would follow that $$ \frac{1}{ \gamma^{\mu} k_{\mu} + m} = \frac{ \gamma^{\nu} k_{\nu} - m}{ k^2 - m^2}. $$ But I think there is a sign mistake, and not sure how to rewrite the term with $1/ ((\gamma^{\mu} p_{\mu} + \gamma^{\nu} k_{\nu}) + m)$.

Answer 1

The last equality on the page follows immediately from seeing that the inverse of $\gamma_{\mu} k^{\mu} + m$ is $-\frac{\gamma_{\mu} k^{\mu} - m}{k^2 + m^2}$. If we assume that all scalar products are euclidean scalar products and either the $k^{\mu}$ is numerator is considered Wick rotate, $k^{\mu} = (i k^0 ,\vec{k})$ (this would be somewhat incosistent) or the gamma matrices are considered Wick rotated $\gamma^{\mu} \rightarrow (i \gamma^0, \vec \gamma)$, which implies $\{ \gamma_{\mu}, \gamma_{\nu} \} = -2 \delta_{\mu \nu}$ (this might be what he means by "gamma matrices square to $-1$"). $$ \frac{\gamma_{\mu} k^{\mu} - m}{k^2 + m^2} (\gamma_{\nu} k^{\nu} + m) = \frac{\gamma_{\mu} \gamma_{\nu} k^{\mu} k^{\nu} - m^2}{k^2 + m^2} = \frac{-(k^0)^2 - \vec k^2 - m^2}{k^2 + m^2} = - 1. $$ I agree that the notation would be somehow weird and since I am not familiar with Tong's lecture notes this may also be an error.