Internal energy does not include kinetic energy of the system as a whole. But why do we consider internal energy as work done by enviornment (if we don't consider heat). E.g., when I fish a block with a force $F$ and perform work on it, the work goes in kinetic energy. However the formula for internal energy includes this work. Some type of contradiction in definition and formulation seems to be there.The formula is $\Delta U=\Delta W+\Delta Q$,where $\Delta W$ is the work done by enviornment on system and $\Delta Q$ is the heat supplied.
$\begingroup$
$\endgroup$
5
-
$\begingroup$ What you say is not correct: the energy that goes to increasing the kinetic energy of an object as a whole (i.e., the energy fo its center-of-mass) is not counted as a change of the internal energy. $\endgroup$– Roger V.Commented Jun 2, 2021 at 10:01
-
$\begingroup$ But the formula says something like tthat ,I understand what you are saying $\endgroup$– Varad TandaleCommented Jun 2, 2021 at 10:03
-
$\begingroup$ Perhaps, you could include this formula in the question. It could be that you apply it incorrectly. $\endgroup$– Roger V.Commented Jun 2, 2021 at 10:05
-
$\begingroup$ I have added the formula there $\endgroup$– Varad TandaleCommented Jun 2, 2021 at 10:13
-
$\begingroup$ In classical mechanics, we have $W=\Delta K$. But in this equation we asssume that internal structure of the body remains same in the initial and final state. But in thermodynamics, internal configuration of system also changes. As when the piston of gas cylinder moves inward, the particles of gas comes closer and thus the internal configuration changes. So $\Delta U$ includes the energy possesed by the internal configuration of system and Kinetic energy of system. But we take kinetic energy of system to be 0 to analyze the energy exchanges to change the internal state of the system. $\endgroup$– ItiCommented Jun 2, 2021 at 10:27
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
However the formula for internal energy includes this work.
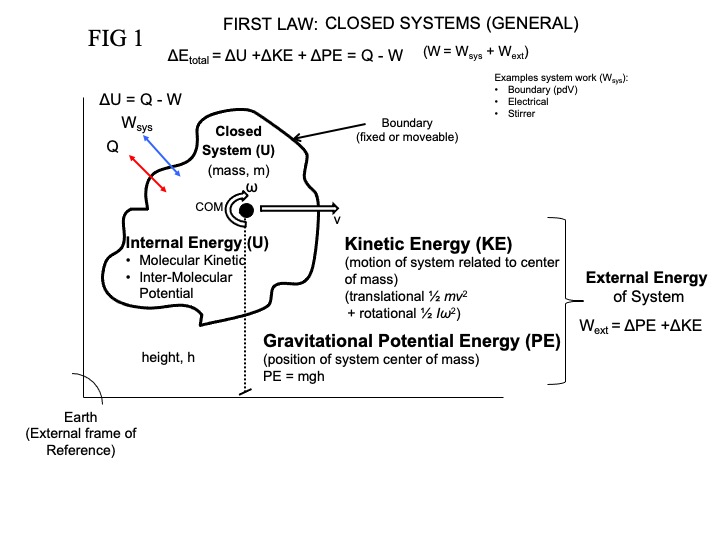
Work $W$ in the form of the first law equation you have given for a closed (no mass transfer) system is boundary work (expansion or contraction of the system boundaries), not the work associated with giving a system as a whole kinetic energy. The work associated with the latter is included in the following general form of the first law equation for a closed system
$$\Delta E_{tot}=\Delta U+\Delta KE +\Delta PE= Q-W$$
(Note that in this version of the first law, $W$ is positive when work is done by the system).
The work $W$ term now includes both the work that crosses the system boundary (that associated with the change in internal energy) as well as the external work on the system as a whole. The terms $\Delta KE$ and $\Delta PE$ refer to changes in the kinetic and potential energy of the system as a whole with respect to an external (to the system) frame of reference.
The figure below illustrates the application of the general form of the first law equation to a closed system.
Hope this helps.
$\begingroup$
$\endgroup$
Generally speaking, the energy transferred to an object goes into increasing its kinetic energy and its internal energy, as requited by the energy conservation. In thermodynamics one is usually interested in the latter, assuming that the object is stationary or moving with a constant speed, and that it is not rotationg. In other words, thermodynamic equations do not include the energy associated with the motion of the center-of-mass of the object.
Thus, in the equations such as $$ \Delta U = \Delta Q + \Delta W, $$ $\Delta W$ is the part of the work that goes into increasing the internal energy of the object, e.g., via changing its shape. This explicitly excludes the work that might go into changing the kinetic energy of the object as a whole.
Unfortunately, thsi point is either not discussed in statistical physics textbooks or discussed in the early chapters, before the equations appear - and therefore not given sufficient attention (e.g., such discussion is present in Landau&Livshits book).
$\begingroup$
$\endgroup$
The more general form of the first law of thermodynamics (if the change can also involve kinetic energy and potential energy changes) reads: $$\Delta U+\Delta KE+ \Delta PE=Q+W$$How does that grab ya?
answered Jun 2, 2021 at 10:51