0
$\begingroup$

I have a dilemma.
If I look at the diagram and say the sum of the forces in x and y direction has to be zero, then I will simply conclude that the force of a wall on a revolvable rod is the blue N as drawn.

But what if the force is actually the green N? To me, it makes more sense because if imagine no tension and no mass M, then the force of a wall N would be drawn as below (green N).

So how can I argue that the orange component of N does exist? If it exists...

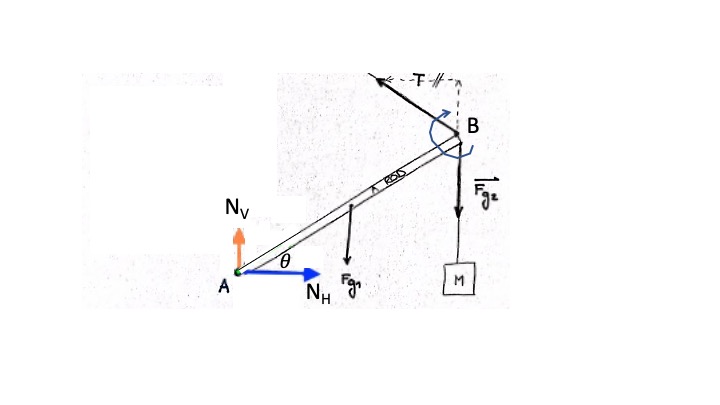
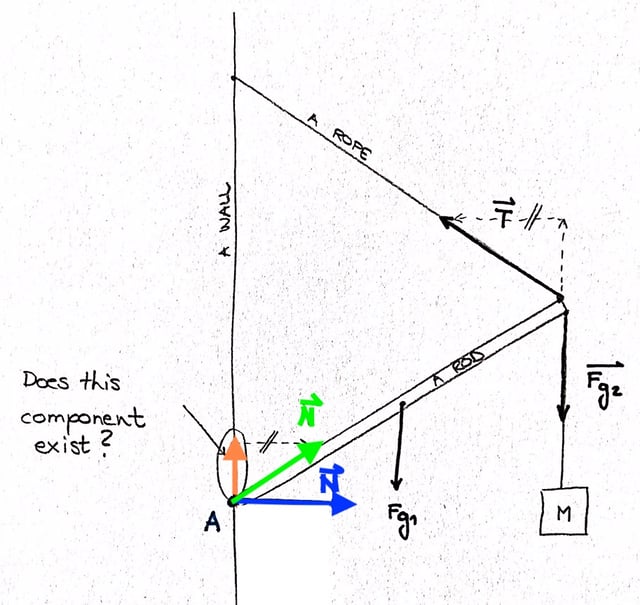
enter image description here

Improve this question
$\endgroup$
2
  • $\begingroup$ Did you try my suggestion? If so, what does it tell you about orange N? $\endgroup$
    – Bob D
    Commented Apr 20, 2021 at 12:00
  • $\begingroup$ @BobD It does make sense. I just had a couple of confusing examples in my study book and the manual solution to one of them was wrong and it confused me. What I actually learned is that I have to look at different points on the body and as you wrote in the answer the sum of the moments have to be 0. It helps for future problems! $\endgroup$
    – thunder
    Commented Apr 21, 2021 at 20:35

1 Answer 1

Reset to default
0
$\begingroup$

If I look at the diagram and say the sum of the forces in x and y direction has to be zero, then I will simply conclude that the force of a wall on a revolvable rod is the blue N as drawn.

In addition to the requirement that the sum of the forces be zero for translational equilibrium, it is also required that the sum of the moments about any point on the rod must be zero for rotational equilibrium. See below.

So how can I argue that the orange component of N does exist? If it exists...

You can argue that the orange component does exist in order that the sum of the moments about the end of the rod supporting the weight M (point B on the FBD below) equals zero.

Note on the FBD the tension force and gravitational force on M do not contribute any moment (torque) about the point B. The two forces $F_{g1}$ and $N_H$ both create a counter clockwise moment about the B. For equilibrium, these moments must be balanced by an equal clockwise moment. The orange component of $N$, $N_H$, is needed to provide that clockwise moment.

Hope this helps.

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.