R134a state at rest

Question

I'm a mechanic and I've had a question for some time regarding AC systems that no one in my garage seems to be able to answer (or care actually).

R134a has a boiling point at -26°C at atmospheric pressure. If pressure increases, boiling point does too, and I've read that at 4.9bar, its boiling point is actually +15°C. When your AC is off, the pressure inside the whole system finds equilibrium at a pressure close to 5bar. At this pressure, if outside temperature is anything less than +15°C, wouldn't R134a become a liquid ?

I've read a lot about pv=nrt but apparently it doesn't work much for refrigerant, and a lot about boiling point and saturation occuring in constant volume, but most of the time, people seem to wonder if liquid can evaporate in a constant volume, and not if gas can condensate.

Any help explaining (if possible in simple terms) what I'm missing here ?

PS : I haven't studied physics for a long time and my question may be particularly dumb for some of you, don't judge :).

Answer 1

If all the R134a in the system condensed to liquid, there would not be enough of it to fill the entire internals of the equipment. So there would also have to be some vapor in there as well, filling part of the equipment. The split between the liquid and vapor would depend on how much R134a there was in the system, the available volume, and the densities of the liquid and vapor at the system temperature.

Answer 2

The ideal gas law $pV=nRT$ is only valid for ideal gases, where interactions between molecules are negligible.

Here is a $pV$-diagram for R134a as a log-log plot:

At low densities, the isotherm at $200\ ^\circ$C behaves like an ideal gas, a straight line with a slope of -1.

At higher densities, there are interactions. For temperatures below the critical point this leads to coexistence of liquid and vapor, with horizontal sections of the isotherms.

The vapor pressure in the two-phase area increases approximately exponentially with temperature, up to the critical point at $101\ ^\circ$C and $40$ bar:

Answer 3

Gases in fixed-volume containers don't do one thing or the other: they form an equilibrium

The mistake I think you are making is that, in a vessel of fixed volume, gases and liquids don't do just one thing (or to use more technical language, have a single state as a liquid or a gas).

Take a more familiar system, water, as an illustration. Water is a liquid at normal atmospheric pressure between 0 and 100 celcius. Does this mean that there is no water vapour in the atmosphere? Clearly not since rain exists and water in standing vessels evaporates. Atmospheric water vapour is, approximately, in equilibrium with the liquid though that equilibrium varies a lot over time explaining rain and fog.

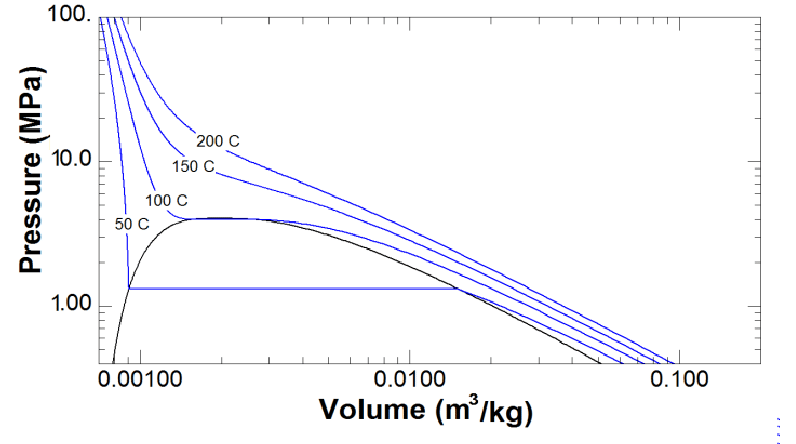
In a vessel of fixed volume you don't just have gas or liquid. You have both. The volume can't vary to compensate for temperature, so what varies is the ratio of liquid to gas inside the vessel. And that varies so that the pressure of the vapour establishes an equilibrium with the remaining liquid. If the temperature is high enough for there to be no liquid, then the pressure will be whatever the equations say the equilibrium pressure is for that much gas and that much volume. Once the temperature is low enough for liquid to exist, then liquid will condense from the gas until the pressure of the remaining vapour reestablishes the "right" equilibrium pressure. Over some range of temperature below a critical point, what will vary is the amount of liquid and the vapour pressure.

This is also how cheap butane cigarette lighters work. Butane is a liquid under normal conditions with only a slight pressure in excess to atmospheric. This is enough to drive the liquid out of the container to burn when ignited. The pressure doesn't vary much but the volume of liquid decreases as the lighter is used. All the time, though, the gas inside is in equilibrium with the liquid (until there is no longer any liquid left and the pressure drops rapidly leaving the lighter defunct.

All this is true unless the liquid completely fills the vessel, of course. When there is space there will always be some vapour in it, in equilibrium with the liquid. If there is no space then you often have a problem as liquids are not very compressible and the pressures can get very, very high very quickly when the temperature rises, which is usually a bad thing.