How is the reference point of potential energy defined in electric fields?

Question

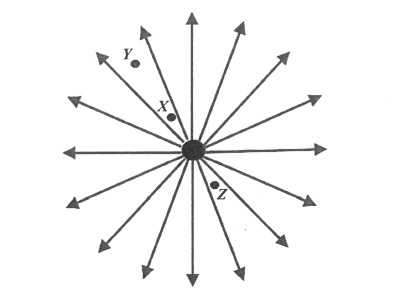
Consider the diagram below of a positive charge and three points marked in the field X, Y and Z.

I understand that for a gravitational field, we define the potential energy as being always negative, and approaching zero as you move further from its source. My questions are, why is this? and how does this differ for the potential in an electric field like the one shown above? Is potential higher or lower at point Y, and does that answer depend on if you are examining a positively charged or negatively charged particle at Y? Furthermore does the zero point become at the source of the field for a different type of charge (+ or -) causing the field?

Answer 1

You see for gravitational or electrostatic fields, Potential is taken to be zero at infinity. Potential is defined with respect to positive charge $q_0$ always. Hence, high potential at a point means that a positive charge$q_0$ at that point will be unstable. (Think in terms of : more energy $\implies$ less stability).So for a positive charge, a point near it has high potential, than a point far off(Potential is zero at infinity and positive otherwise for a positive charge) Since $q_0$ repels positive charge and wants to be as far from it to be stable. For a negative charge, the potential is zero at infinity and negative otherwise. Hence closer the point to negative charge , lesser potential,more stability, since $q_0$ wants to be as close to a negative charge.

For gravitation, the thing is same like two unlike charges, hence same concept of a negative charge.