When is it true that "for a given velocity field, the curl represents a vector equal to twice the angular velocity vector"?

Question

On page 154 of Theoretical Physics, By: Georg Joos, Ira M. Freeman the following is stated:

Thus for a given velocity field, $\text{curl} \mathfrak{v}$ represents a vector equal to twice the angular velocity vector.

This is in the context of discussing strain in the study of deformable solids. The argument begins with the observation that for a rigid body the velocity field due to rotation is given by $\mathfrak{v}=\vec{\omega}\times\mathfrak{r}$. Taking the curl gives $\nabla\times\mathfrak{v}=2\vec{\omega}$.

The objective is to find the part of the strain displacement $d_{\text{rot}}=\left(\vec{\omega}\times\mathfrak{r}\right)dt$ representing the rotation of the element under consideration so it can be subtracted, along with gross translation from the expression for the overall displacement field, leaving only the deformation part. In the context this seems reasonable.

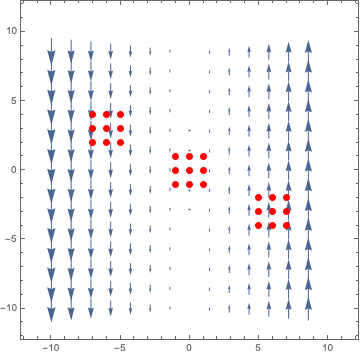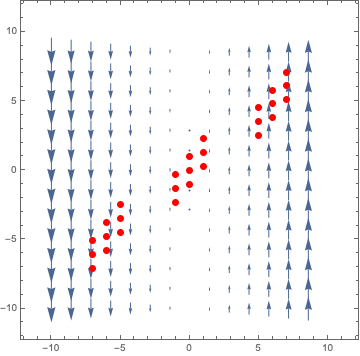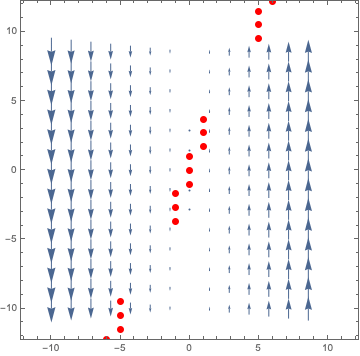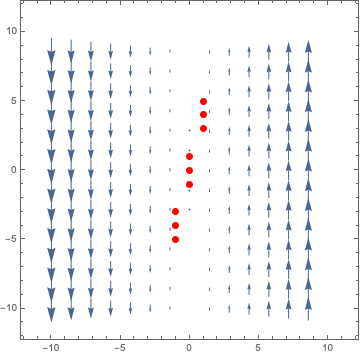
But as a general rule, the quoted statement seems to fail. For example in the case of laminar flow. Consider a velocity field defined by $\dot{x}=\dot{z}=0$ and $\dot{y}=x$. The curl is simply $\hat{\mathbf{k}}.$ But this doesn't represent an angular velocity vector for the velocity field. I can't imagine what an angular velocity vector for that velocity field would be.

Under what conditions (or assumptions) is the relationship $\nabla\times\mathfrak{v}=2\vec{\omega}$ applicable?

Answer 1

Under what assumptions is the relationship $\nabla\times\vec v=2\vec\omega$ applicable?

In polar coordinates, \begin{align*} \vec v&=\dot{\vec r}=\frac{d(r\hat r)}{dt}=\dot r\hat r+r\frac{d\hat r}{dt}=\dot r\hat r+r\dot\theta\hat\theta=\dot r\hat r+r\dot\theta(\hat z\times\hat r)=\dot r\hat r+(\dot\theta\hat z\times r\hat r)=\dot r\hat r+\vec\omega\times\vec r\\ \end{align*} So, $\vec v=\vec\omega\times\vec r$ iff $\dot r\hat r=0$ or no radial movement. Rigid bodies aren't the only ones whose points' motion is described by this equation. Imagine a rotating fluid paraboloid in a container with $\omega\equiv\omega(r)$ in which rings farther from the axis rotate faster than rings nearer. But, since $\vec \omega$ is constant for the whole body, $|\vec v|\propto r$.

Now let's examine $\vec\nabla\times\vec v$ as follows: \begin{align*} \vec\nabla\times\vec v=\vec\nabla\times(\vec\omega\times\vec r)&=(\vec\nabla\cdot\vec r)\vec \omega-(\vec\nabla\cdot\vec \omega)\vec r+(\vec r\cdot\vec\nabla)\vec \omega-(\vec \omega\cdot\vec\nabla)\vec r\\ &=3\vec{\omega}-(\vec\nabla\cdot\vec \omega)\vec r+(\vec r\cdot\vec\nabla)\vec \omega-\vec\omega\\ &=2\vec{\omega}-\left(\frac{\partial w_z}{\partial z}\right)(x\hat i+y\hat j+z\hat k)+\left(x\frac{\partial}{\partial x}+y\frac{\partial}{\partial y}+z\frac{\partial}{\partial z}\right)(\omega_z\hat k)\\ &=2\vec{\omega}-x\frac{\partial w_z}{\partial z}\hat i-y\frac{\partial w_z}{\partial z}\hat j+\left(x\frac{\partial \omega_z}{\partial x}+y\frac{\partial \omega_z}{\partial y}\right)\hat k\\ \end{align*} In order for $\vec\nabla\times\vec v=2\vec{\omega}$ to be true, $\frac{\partial w_z}{\partial z}=0$ and $x\frac{\partial \omega_z}{\partial x}+y\frac{\partial \omega_z}{\partial y}=0$. So, if you can find such an $\omega_z$, you are good to go! One example is rigid body definitely, in that $\vec \omega$ doesn't depend on space. $\omega_z=c\frac xy$ also satisfies the equations, but I don't know if it's realistic.

Also, we generally use vorticity instead of angular velocity for laminar flow.

Answer 2

It is applicable quite generally. The flow you describe possesses angular momentum, in the sense that a fluid parcel moving with the flow is rotating.

(You may need to click or refresh to see this animated gif)

If you have a velocity field $\mathbf v$, then the angular velocity at a point $\mathbf r$ is the angular velocity of a fluid parcel which is located at that point, or of an infinitesimal pinwheel which is fixed there.

Angular velocity is generally less useful in fluid flow than the related vorticity. Since it is so closely related, you might find the linked Wikipedia article interesting.