The magnetic monopoles does not exist which can be shown by $ \int {\vec{B} \cdot d\vec{A}} = 0 $.
But in Faraday's Law of electromagnetic induction, we clearly show the EMF induced is the time rate of change of the magnetic flux, which is $ E = -\frac{d\Phi_B}{dt} = -\frac{d\int{\vec{B}\cdot d\vec{A}}} {dt}$.
Now if $ \int {\vec{B} \cdot d\vec{A}} = 0 $ then shouldn't the induced emf be zero?
This can be resolved by being clear about what surface you're integrating over. In the first equation, $$ \oint {\overrightarrow{B} . \overrightarrow{dA}} = 0, $$ you're integrating over any closed surface, i.e. a surface without a hole in it, such as a sphere. The equation says that the magnetic flux coming in must equal the magnetic flux going out.
But in the second equation, $$ E = \frac{d}{dt} \int_\Sigma {\overrightarrow{B} . \overrightarrow{dA}}, $$ you're integrating over a surface with a hole in it, where the hole is a loop of wire, as shown in this diagram from Wikipedia:
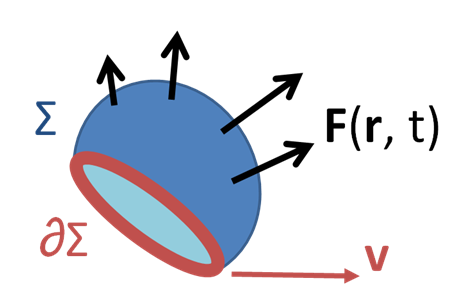
This equation says that the rate of change of flux passing through the surface must equal the EMF in the wire loop. You can imagine it as coming in through the hole, and out through the surface. (Or the other way round or a bit of both.)
You can see a connection between the two equations if you imagine making the wire loop smaller and smaller until the hole closes completely. Then you're back at a closed surface again, where the first equation applies - and this infinitely small loop of wire can never experience an EMF.
