Context:
For instance, the quantity Torque, $\vec{\tau}$ is defined about a point: by the formula $$\vec{\tau}=\vec{r}\times\vec{F}$$ We can use this defintion to define torque about an axis. Let the axis be along the vector $\vec{n}$. If the torque of a force about a point on the axis,=$\vec{\tau_1}$=$\vec{r}\times\vec{F}$, Then, torque of the same force about another point on the axis$=$$\vec{\tau_2}$$=$ $(\vec{r}+\lambda\vec{n})\times\vec{F}$. Clearly, $\vec{\tau_1}.\vec{n}=\vec{\tau_2}.\vec{n}$.
The component of torque about the axis direction, is the same for any point on the axis, and this component is what is defined as the torque about an axis.
I believe, that a similar treatment fails for angular velocity. I present an example:
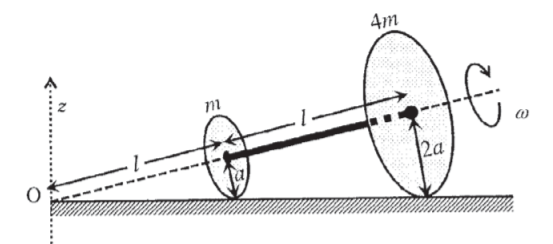
Two thin circular discs of mass $m$ and $4 m$, having radii of $a$ and $2 a$, respectively, are rigidly fixed by a massless, rigid rod of length $l=\sqrt{24} a$ through their centers. This assembly is laid on a firm and flat surface, and set rolling without slipping on the surface so that the angular speed about the axis of the rod is $\omega$. The angular momentum of the entire assembly about the point ' $O$ ' is $\vec{L}$ (see the figure).
Let $\theta$ be the angle made by the massless rod with the horizontal.The statement(which we had to check):
The Center of mass of the system rotates about the Z axis with an angular speed $\omega/5$.
The solution presented:
The center of mass of the system is at a distance $9l/5$ from point $o$.If angular velocity vector of the center of mass relative to point $o$ is $\vec{\Omega}$,Then $\vec{\Omega}$ will be at an angle $\theta$ from the z-axis. Since the velocity of the center of mass is the disc is $\dfrac{m(a\omega)+4m(2a\omega)}{m+4m}$=$(9/5)a\omega$, it follows that $|\vec{\Omega}|$ =$\dfrac{|\vec{r}\times\vec{v}|}{|\vec{r}|^2}$=$\dfrac{|\vec{r}||\vec{v}|}{|\vec{r}|^2}$=$\dfrac{|\vec{v}|}{|\vec{r}|}$=$a\omega/l$, And thus $\vec{\Omega}.\hat{k}$=$|\vec{\Omega}|\cos\theta=a\omega\cos(\theta)/l=\omega/5$.
My issue with the solution:It seems that they have calculated the z-component of angular velocity of the Center of mass, relative to point $o$. The question asked us to find the "angular velocity vector of the center of mass" about the z axis.
I fail to see how these two are equivalent. The equivalence of these two statements implies that the component about the axis,of the angular velocity vector relative to any point on the axis,is the same.(a concept which worked for torque). This is false, it can be easily shown(using the same procedure as torque).The z component of the Angular velocity vector of the Center of mass are different relative to different point for the Z axis, If we go by the following formula:
$$\vec{\omega}=\dfrac{\vec{r}\times\vec{v_{a}}}{|\vec{r}|^2}$$
Where ${\vec{r}}$ is the vector joining $a$ to $b$. The treatment which worked for torque, fails for angular velocity. Hence, the question.
Instantaneous axis of rotation and rolling cone motion presents a very similar question. As per its last paragraph, in our example, Velocities of the centers of the discs ** won't** be $a\omega$ and $2a\omega$!! So it seems to me that the presented solution is completely wrong.
The statement in the bold is still un-answered.