Why does a voltmeter have to have nearly no current passing through it?

Question

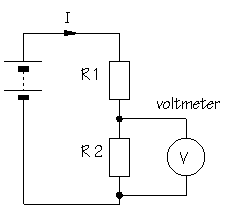
I understand that a high resistance of a voltmeter will allow nearly no current to pass through it. However, why is this condition required? Let's consider a simple circuit that consists of a cell, and a resistor. The potential drop across the resistor will be the same as the potential difference across the cell. Now, if we introduce a voltmeter across the resistor, since the voltmeter and the resistor are connected in parallel, the potential drop across the resistor and the voltmeter will be the same, although the current passing through the resistor and the voltmeter will vary depending on their resistances. So, to me, it seems like the potential drop across the resistor (which the voltmeter measures) is independent of the current flowing through the resistor and the voltmeter, and thus independent of the resistance of the voltmeter.

So, why should there be nearly no current flowing through a voltmeter (which is the reason why the voltmeter has to have a very high resistance)?

Answer 1

Without Voltmeter

See the circuit given below. Before inserting the voltmeter, the current through the circuit is $ E /(R_1 +R_2)$ where $E$ is the EMF of the battery. So the potential difference across $R_2$ is

$$V_0=E\frac{R_2}{R_1+R_2}$$

^{Image source}

After Voltmeter

Let ther resistance of voltmeter be $R_V$. Then the equivalent resistance of the circuit will be

$$R_{\text{eq}} =R_1 + \frac{R_2 R_V}{R_2+R_V}$$

Therefore the current through the circuit will be

$$I_{\text{total}}= \frac{E}{R_{\text{eq}}}$$

And the current through $R_2$ will be,

$$I_2 = I_{\text{total}} \frac{R_V}{R_2 + R_V}$$

Therefore the potential difference across $R_2$ will be

$$V=I_2 R_2 = I_{\text{total}} \frac{R_V R_2}{R_2 + R_V}= \frac{E}{R_{\text{eq}}}\frac{R_V R_2}{R_2 + R_V} =\frac{E}{ R_1 + \frac{R_2 R_V}{R_2+R_V}}\frac{R_V R_2}{R_2 + R_V} $$

which simplifies to

$$V= E \frac{R_2 R_V}{R_1 R_2 +R_2 R_V + R_V R_1}$$

Clearly this is different from the original result without the voltmeter($V_0$). Also when $R_V \rightarrow \infty$, $V\rightarrow V_0$ which is expected. At all finite values of $R_V$, $V<V_0$

Intuition

When you connect a voltmeter in parallel, the equivalent resistance of the circuit decreases and the total current increases. But at the same time the current also gets divided across $R_2$ and $R_V$ which results in lower current through $R_2$. The latter effect dominates and a lower potential difference is obtained across $R_2$. So we try to minimize these two effects by increasing $R_V$. This is clearly evident from the mathematics of this scenario.

Answer 2

The goal is indeed for the voltmeter to have the smallest possible effect on the rest of circuit. The higher the resistance, the less of an effect. If you imagine the opposite extreme is it more clear: no matter the circuit, if you take the resistance of the voltmeter to zero, the voltage measured will go to zero too (and you'll probably break something).

When I took my AC/DC electronics course, we were forced to use these ancient voltmeters (example). The challenge with these is that the resistance was actually quite low, maybe only 100 Ohms (depending on the setting), so they would dramatically effect the circuit. In order to get the correct voltage (before you attached the voltmeter) you had to (by hand) work out how the voltmeter affected the circuit and then calculate the correct voltage.

Answer 3

Generally when we connect Voltmeters or Ammeters, we do not want to disturb the rest of the circuit. This means preserve the current and voltage difference in the circuit as much as possible. And since any resistance connected parallel across a portion of the circuit will provide the same potential difference, the least hamper done to the main circuit is when the current in the new branch (voltmeter branch) is as low as possible. Since $V=IR$, to reduce the current we use as large an $R$ as possible.

And similarly we can reason out why the resistance must be small for an ammeter (connected in series).