A question about photoelectric effect (graph)

Question

I'm convinced with the graph except for when x=0.

When $x=0$, the collector is at 0 potential. So photoelectrons that are emitted from the plate are not influenced by any electric field.

Since there is no electric field, the photoelectrons are equally likely to go in a particular direction (towards collector plate) regardless of their energy.

So, since the same amount of electrons are being emitted from the plate by source of different frequencies and since the electrons are equally likely to go towards the collector plate,

I say that at $x=0$, the photocurrent should be the same for all three curves.

Please tell me whether what I have said is right or wrong. And if it's wrong, why is it wrong?

Experiment diagram: !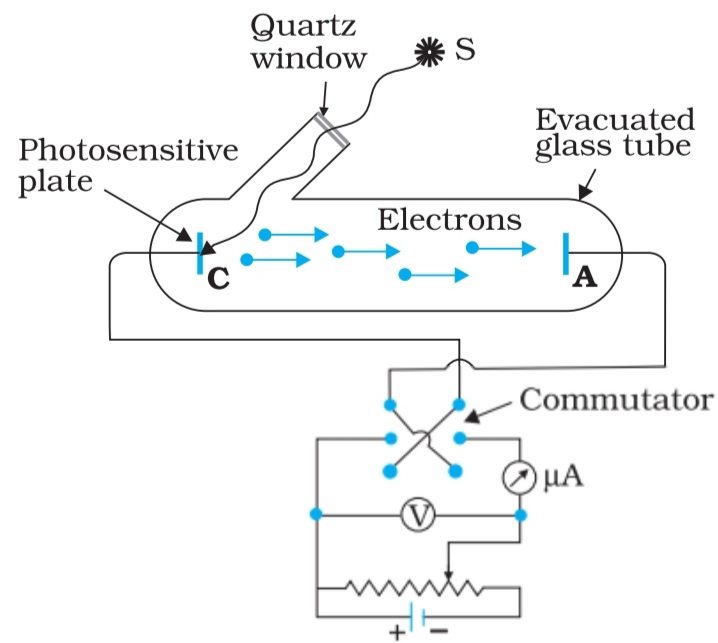

Answer 1

Electric current law in terms of current density $j$: $$ j = \rho\,u$$ where $\rho$ is charge density and $u$ - electron drift velocity. Expressing equation in terms of electron drift kinetic energy : $$ j = \rho\sqrt{2\frac{E_k}{m_e}}$$ where $m_e$ is electron mass. Finally, incident photon does work by freeing electron from a surface and accelerating it, so : $$ j = \rho\sqrt{2\frac{h\nu-\psi}{m_e}} $$ where $\psi$ is work function - energy needed to free electron from a surface. Now you clearly see that increasing incident photon frequency, makes photo-current density bigger, because photon transfers more energy to electron drift velocity. When you apply additionally external electric field, depending on voltage sign - it either stars stopping electrons, by reducing their kinetic energy or in reverse - by accelerating them even to a greater speeds until saturation is reached. Hope that helps.

EDIT:

This equation is for no external electric field applied ($V_{\textrm{external}}=0$).

Answer 2

Most of such graphs are neither data nor theoretical computations; they are just sketches. The main thing that is wrong in this case is that the saturation current seems to be the same for all frequencies. But that would be ok if the vertical axis is given as a percentage of the saturation current.

There is not necessarily a problem at $V=0$. Zero applied voltage does not mean zero field because the photocathode work function can differs from that of the anode (normally it is significantly lower).

Answer 3

Photoelectric effect is only observed when electrons reach the positive plate and to reach the positive plate they must have sufficient energy. A part of this required energy is the electrons kinetic energy and the rest comes from the energy of the incident light. You must agree that all the electrons don't have the same energy so all the electrons do not require the same amount of energy for emission from the metal plate. Thus, light of lower frequencies may not provide the energy required by some of the electrons for emission. You will easily understand this with an example. Suppose that there are 50 electrons in a certain area of the metal. 10 of them require only 6 eV of energy for emission, other 10 of them require 10 eV energy, and the rest of them require 20 eV energy. So, Light having energy,say 25eV(of greater frequency) will release more electrons than light of 15 eV energy ( of lower frequency)

Edit 1: Consider 2 beams of light having same intensity (i.e.,same energy) but beam 1 has higher frequency, so each photon of it have higher frequency and thus higher energy too( from planks law, E=hv). So, a lesser number of photons of beam 1 have the same energy as beam 2( having greater number of photons). Thus, beam 1 and beam 2 having same intensities have different number of photons.(You also must know that all the photons do not successfully emit an electron, this happens only for saturation current) See what happens at saturation current: the photons of beam 1 impart greater kinetic energy to the electrons, so the electrons move faster as compared to the electrons emitted by beam 2, which impart lower kinetic energy. But, there are lesser number of photons in beam 1. So the net effect is that the saturation current due to beam 1 have lesser number of photoelectrons but those photoelectrons move faster! But the photoelectrons due to beam 2 are greater but move slowly. As a result the rate of flow of " charge" remains same in both cases. Since current is defined as " rate " of flow of " charge" so current due to both the beams have the same value!

Answer 4

Firstly, you must keep in mind that current is the rate of flow of charge. So to change current you may change the number of charge carriers crossing the conductor's cross sectional area (CSA) per unit time or you may accelerate or deaccelerate the charge carriers so that a greater or lesser number of those charge carriers cross that CSA per unit time. A lower frequency light have lesser energy so it cannot accelerate the photoelectrons( the charge carriers in this case) as much as a higher frequency light. So photocurrent decreases for light of lower frequencies.

Also, a lower frequency light does not emits as much electrons as compared to light of higher frequency ( it does not carries sufficient energy to free the lesser energetic electrons (

But saturation current is same for different frequency lights. This is a special case, lower frequency light have greater number of photons so they can emit more number of photoelectrons, i.e., increase the number of charge carriers. All the photons of the light beam incident emits photoelectrons only at saturation current because at higher voltages the electrons in the metal plate become more energetic so requires lesser energy.