1
$\begingroup$

I just thinking that, if we could place satellite to orbit earth in opposite direction of earth rotation, inverse of geostationary orbit. If we carefully choose a speed to sync with earth rotation, it would have that satellite stay exactly the same position in the sky as the sun

What is that altitude and are there any name of that orbit? Or is it just the same as geostationary orbit?

Improve this question
$\endgroup$
1
  • $\begingroup$ Look into sun-synchronous orbits. They are slightly retrograde, near-polar orbits, that use a Pacific mass concentration to perturb their orbits to rotate their orbital plane as the Earth travels around the sun. A large fraction of LEO satellites use them. $\endgroup$
    – notovny
    Commented Nov 26, 2019 at 11:38

2 Answers 2

Reset to default
3
$\begingroup$

I think what you're talking about are Lagrangian points:

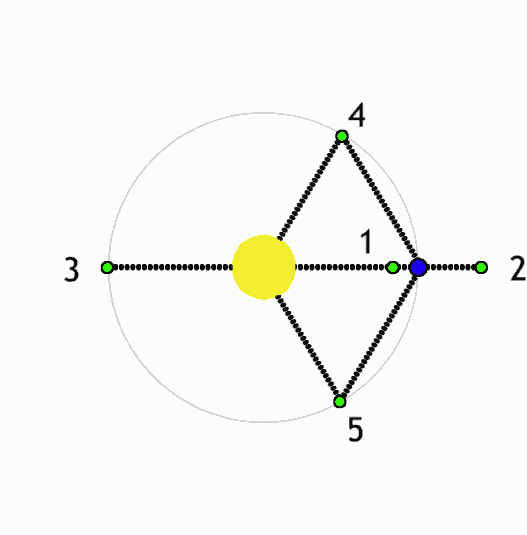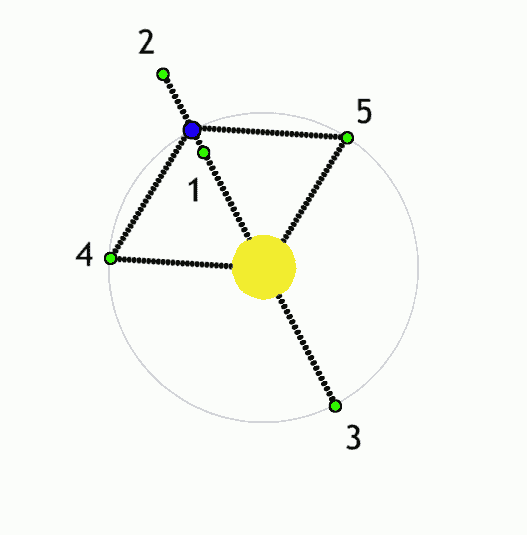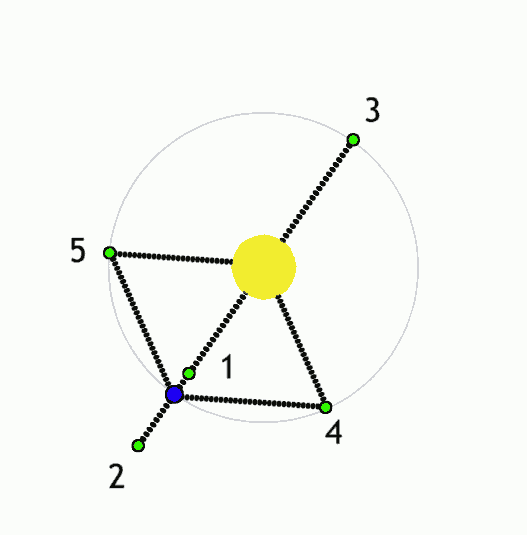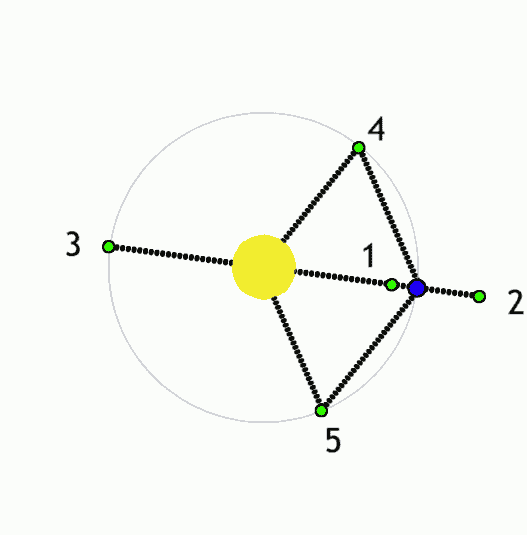
enter image description here

Image Credit: Anynobody / Wikimedia Commons

They are points such that the combined gravitational effects from the Earth and the Sun give rise to areas that would have the same angular velocity as the Earth. However, out of the five lagrange points shown below: 1,2,3 are unstable

You are probably referring to the first Lagrangian point, known as $L_1$ which is roughly $0.01$ AU away from Earth.

Edit: Turns out I misunderstood what you were asking for. If you were curious if such a point can exist such that it is still "orbiting" around the Earth, the answer is no. You may think that as the orbit goes further and further out, you can eventually reduce the period to one year. However, at this point the effect from the Sun cannot be ignored and you can't realy call it "orbiting" around Earth.

We have:

$$m\left(\frac{2\pi}{T}\right)^2 r = \frac{GMm}{r^2} \to r^3 = \frac{GMT^2}{4\pi^2}$$

Plugging in $G=6.67\times 10^{-11} \mathrm{N\cdot m^2 \cdot kg^{-2}}$, $M=5.972 \times 10^{24} \text{ kg}$, and $T=31556926 \text{ s}$ gives:

$$r = 2.16 \times 10^9 \text{ m}$$

while $L_1$ is at a distance of 0.01 AU or $1.50 \times 10^9 \text{ m}$ which is slightly farther than the $L_1$ point which exists there due to the interaction between the Earth AND the Sun, so a satellite cannot orbit around Earth with a period of one year.

Improve this answer
$\endgroup$
8
  • 1
    $\begingroup$ Note, though, that a satellite at the Lagrange point isn't "orbiting the Earth" in the same sense that a satellite in LEO or geostationary orbit is. In those cases, the gravitational force from the Sun can be basically ignored; but the existence of the Lagrange points depends critically on the forces from both the Sun and the Earth. In other words, if the Sun didn't exist, the orbit described by the OP would be impossible. $\endgroup$
    – Michael Seifert
    Commented Nov 24, 2019 at 21:03
  • $\begingroup$ Please properly credit the author of the diagram, which is from WP. This is required by the license and also by common courtesy. $\endgroup$
    – user4552
    Commented Nov 24, 2019 at 21:32
  • $\begingroup$ @BenCrowell my bad, I added the appropriate credits now $\endgroup$
    – QiLin Xue
    Commented Nov 24, 2019 at 21:46
  • $\begingroup$ The OP is asking about orbits around Earth though. $\endgroup$
    – BioPhysicist
    Commented Nov 24, 2019 at 22:17
  • $\begingroup$ @MichaelSeifert If the Sun did not exits, there would still be an orbit around the earth with a period of 1 year. It would be 5 times as far from the Earth than the L1 point is in reality though. $\endgroup$
    – TimRias
    Commented Nov 24, 2019 at 22:23
0
$\begingroup$

It wouldn't be geostationary because we would see it move across the sky. I think you mean geosynchronous, which is what the orbit would almost be. If you wanted it to always stay in front of the sun from some special vantage point, then you would need to somehow have the satellite correct its orbit based on the changing tilt of the Earth.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.