0
$\begingroup$

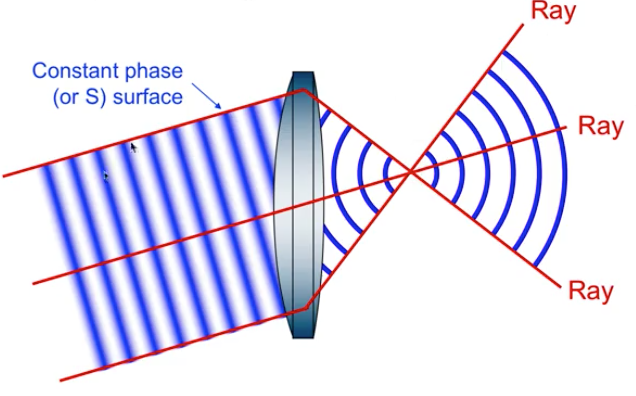
In my current studies of geometrical optics, I have been presented with the following image:

enter image description here

In looking at this diagram, I've been curious about the behaviour of the waves and the accompanying rays at the focus.

If my understanding is correct, when the amplitude of the wave is changing slowly, the ray equation is a valid solution to Maxwell's equations, and can therefore be used to evaluate optical systems, or something of the sort.

In researching this question, I was able to find the following on page 388 of Electromagnetic Fields, by Jean G. Van Bladel:

An important example of failure occurs when the rays converge to a focus, where the theory predicts an infinite value for the power density of $I$ in (8.101).

But this still isn't an explanation, although it at least confirms what I suspected: The ray equation is invalid at foci.

I would greatly appreciate it if people could please take the time to explain why the ray equation is invalid at foci.

Improve this question
$\endgroup$
2
  • $\begingroup$ Please don't cut and paste on the internet without crediting the author. It's rude. $\endgroup$
    – user4552
    Commented Nov 20, 2019 at 21:29
  • $\begingroup$ @BenCrowell What aspect of my post are you referring to? $\endgroup$
    – The Pointer
    Commented Nov 20, 2019 at 21:33

1 Answer 1

Reset to default
2
$\begingroup$

According to the ray equations, a wave inciding on a converging lens could, in principle, generate infinite intensity at the focus, but this assumes the lens is perfect (i.e., free of any aberrations) and that the inciding wave is perfectly plane. Naturally, both assumptions do not hold exactly in a real physical system, so no such infinite intensity occurs. The real value of ray equations is found in approximations and conceptual/pedagogical approaches.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks for the answer. Your answer raises three questions for me: 1. So are you saying that, in general, assuming a perfect lens, it is not true that the ray equation is invalid at foci for all types of lenses? 2. If 1. is correct, then which types of lenses is it valid for (and which is it not)? 3. So, in a real physical system, where it is practically impossible to have a perfect lens, the ray equation is (in practice) valid at foci? $\endgroup$
    – The Pointer
    Commented Nov 20, 2019 at 20:53
  • $\begingroup$ All 3 questions can be answered in the same way: even for ideal lenses, the ray equations only hold perfectly for plane waves - which are indeed solutions of Maxwell's equations, but represent only approximations in real world. For a more realistic inciding wave, I suggest you look for the so-called Gaussian beams. They are frequently used as decent approximations for beams generated by lasers, for example. Their focusing can be described in terms of the lens focus as well, but is more complicated than the ray equation (see Pedrotti L.M., Introduction to Optics, page 475, for example) . $\endgroup$
    – Woe
    Commented Nov 20, 2019 at 21:03
  • $\begingroup$ Interesting. Thanks for the clarification. $\endgroup$
    – The Pointer
    Commented Nov 20, 2019 at 21:06

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.