2
$\begingroup$

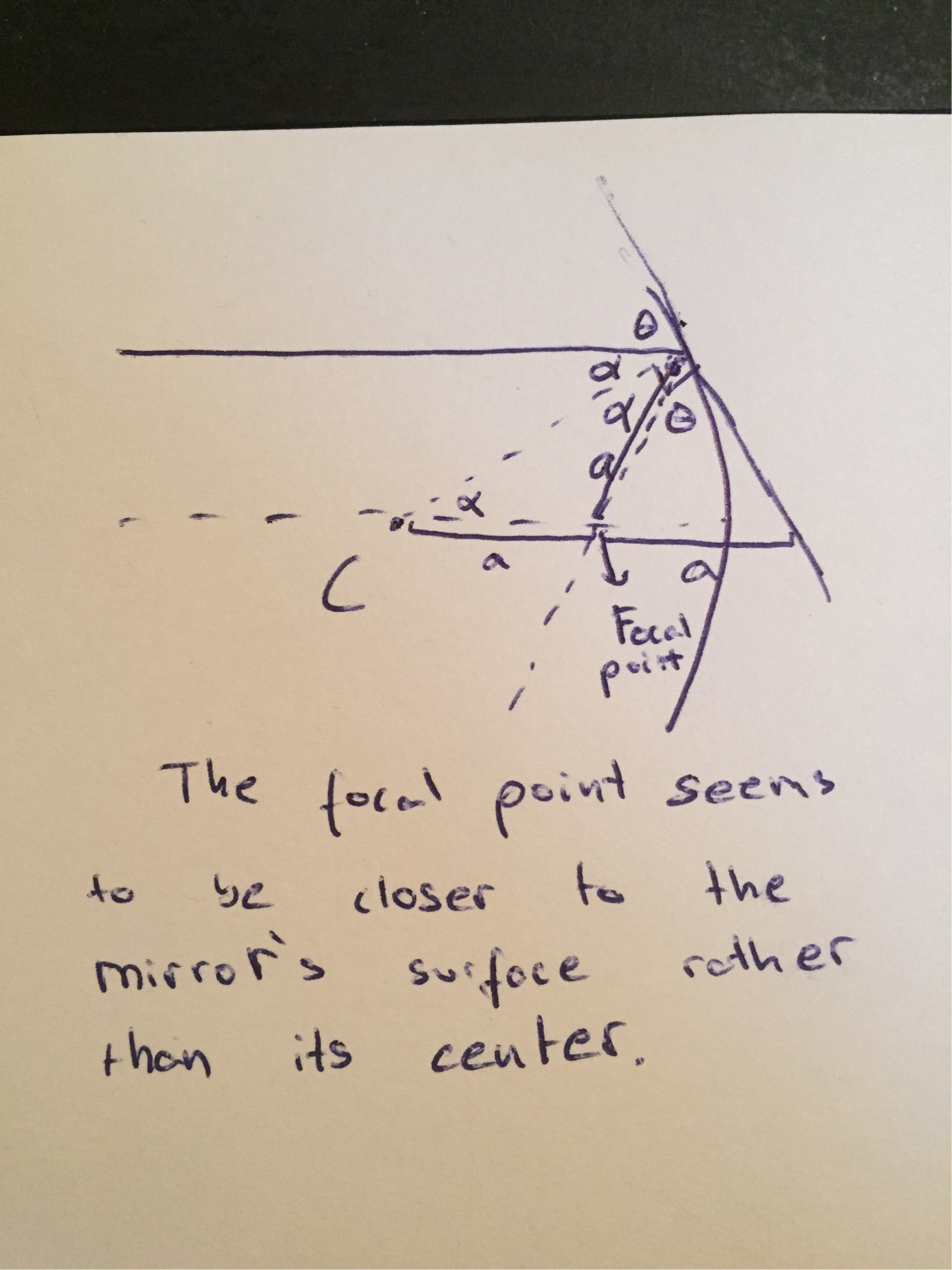
I am a high school student trying to learn spherical mirrors, and I’ve been told that the focal point is the equidistant point between the center and the surface of the sphere. I played around with the system geometrically and it seems like this information is a bit off. Tried to explain it better with a sketch. enter image description here

Text in the Image: The focal point seems to be closer to the mirror's surface rather than it's centre.

Am I missing something or is this some kind of an approximation that works with very big spheres where the discrepancy of the surface and the tangential line would be smaller?

Improve this question
$\endgroup$
1
  • $\begingroup$ Generally, rays parallel to the principal axis and closer to it pass through or appear to come from the focal point. Such rays are called paraxial rays and your statement holds true only for such rays. For marginal rays, i.e., far away from the principal axis the rays get focussed closer to the pole as you have described in your question. $\endgroup$
    – Vishnu
    Commented Jan 6, 2020 at 12:21

3 Answers 3

Reset to default
1
$\begingroup$

You are right. The focal length is define using paraxial rays. These are very close to the axis.A paraboli6 mirror would send the rays you drew through the focus.For the shallow curves of most telescope mirrors, the difference between a sphere and parabola might be around a wavelength of light.

Improve this answer
$\endgroup$
1
$\begingroup$

You are right — it's the known deception of poor basic school / high school student using (perverted) math to “prove” something with is not true.

Open-eyed students as you often reveal that the math here is used inappropriately, and will be for a long time very suspicious about every usage of math for proving statements in physics (my personal experience with the same issue many years ago).

I don't understand why teachers (and textbooks, too) don't simply say:

This “proof” is not a proof — it is only a very good approximation for paraxial rays in a very close distance from the axis. Only for parabolic mirrors it's perfectly true.

Improve this answer
$\endgroup$
0
$\begingroup$

I think the diagram is a bit off. Your reflected ray is suffering much larger reflection (

Rough hand drawings dont help in ray optics.Look up online for more diagrams and you will see.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.