Circular inversion and spherical mirrors

Question

The object distance ($u$), image distance ($v$) and the focal length of a spherical mirror ($f$) are related by the well-known formula (using the appropriate sign convention):

$$ \frac{1}{u} + \frac{1}{v} = \frac{1}{f} $$

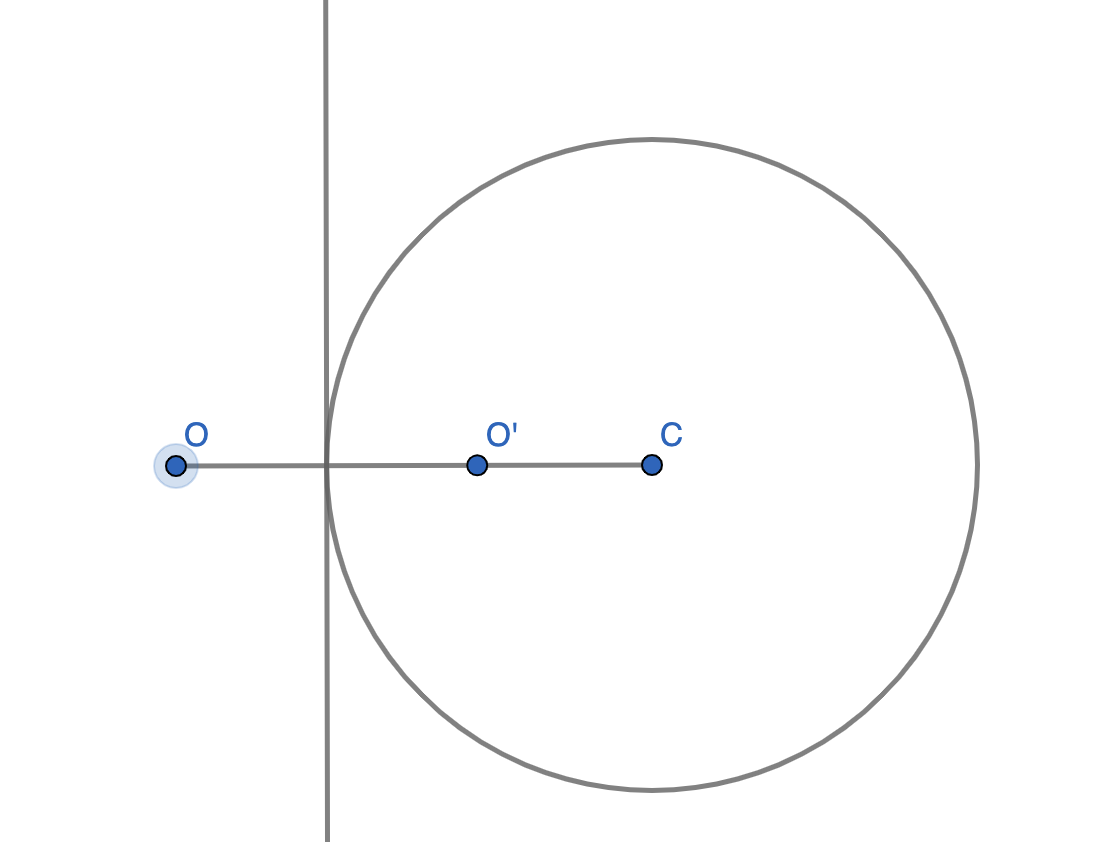
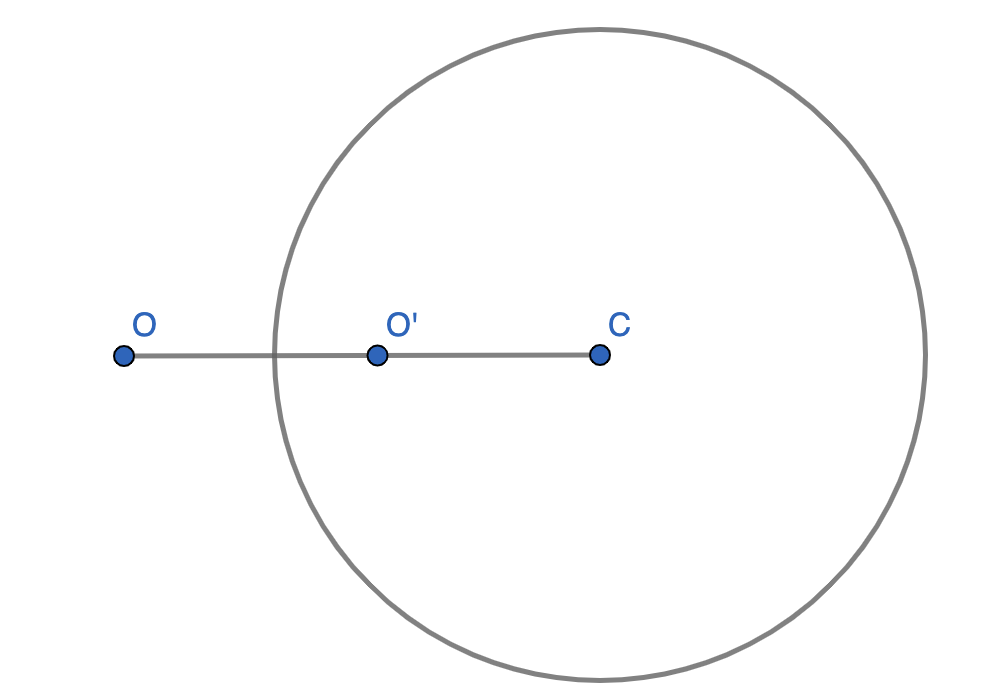
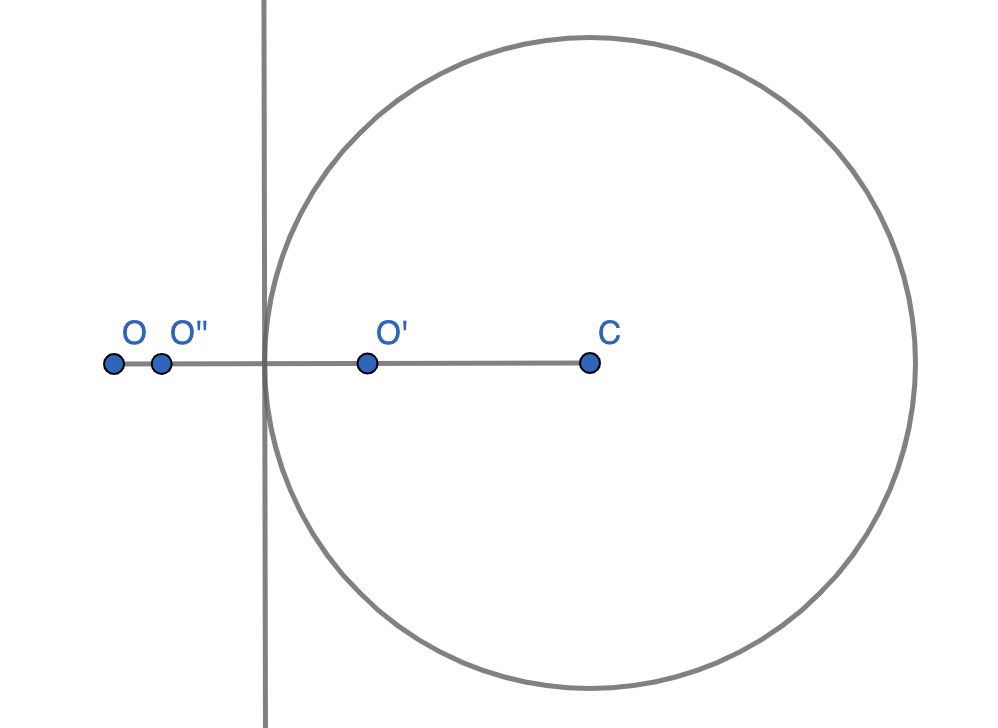
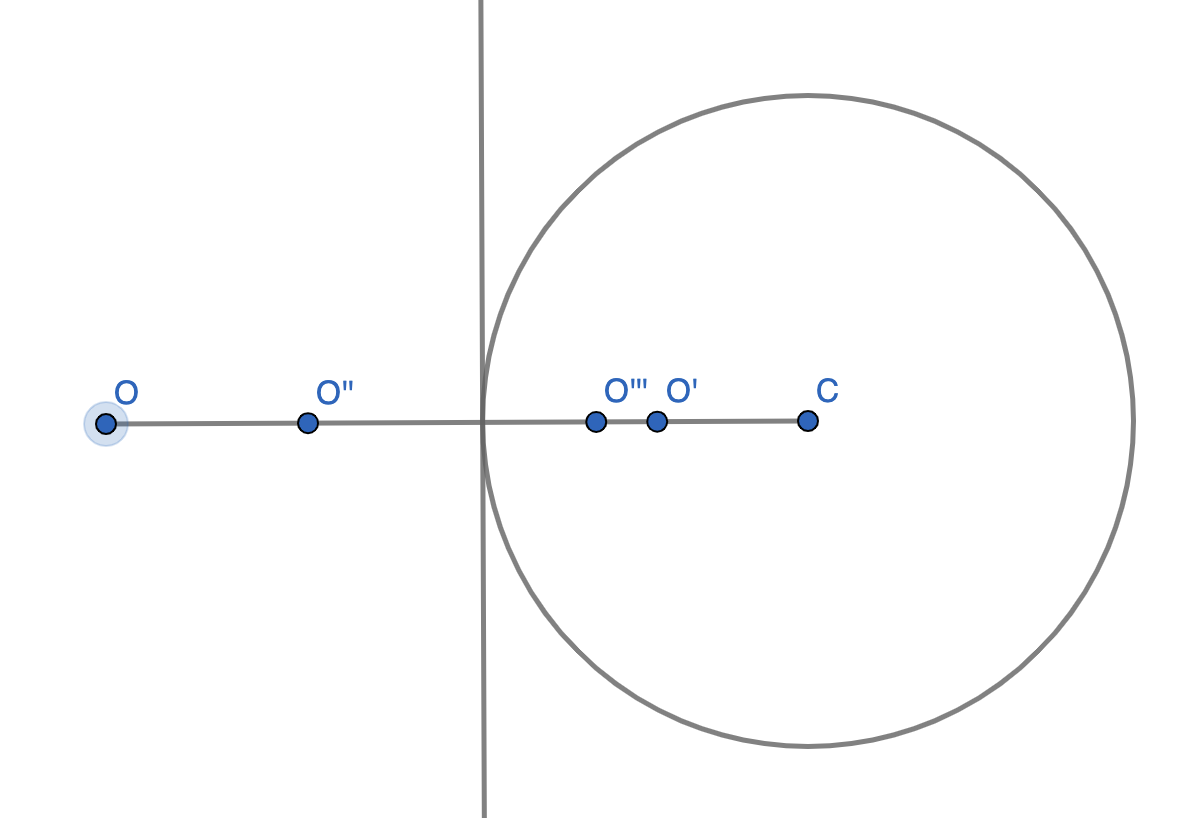
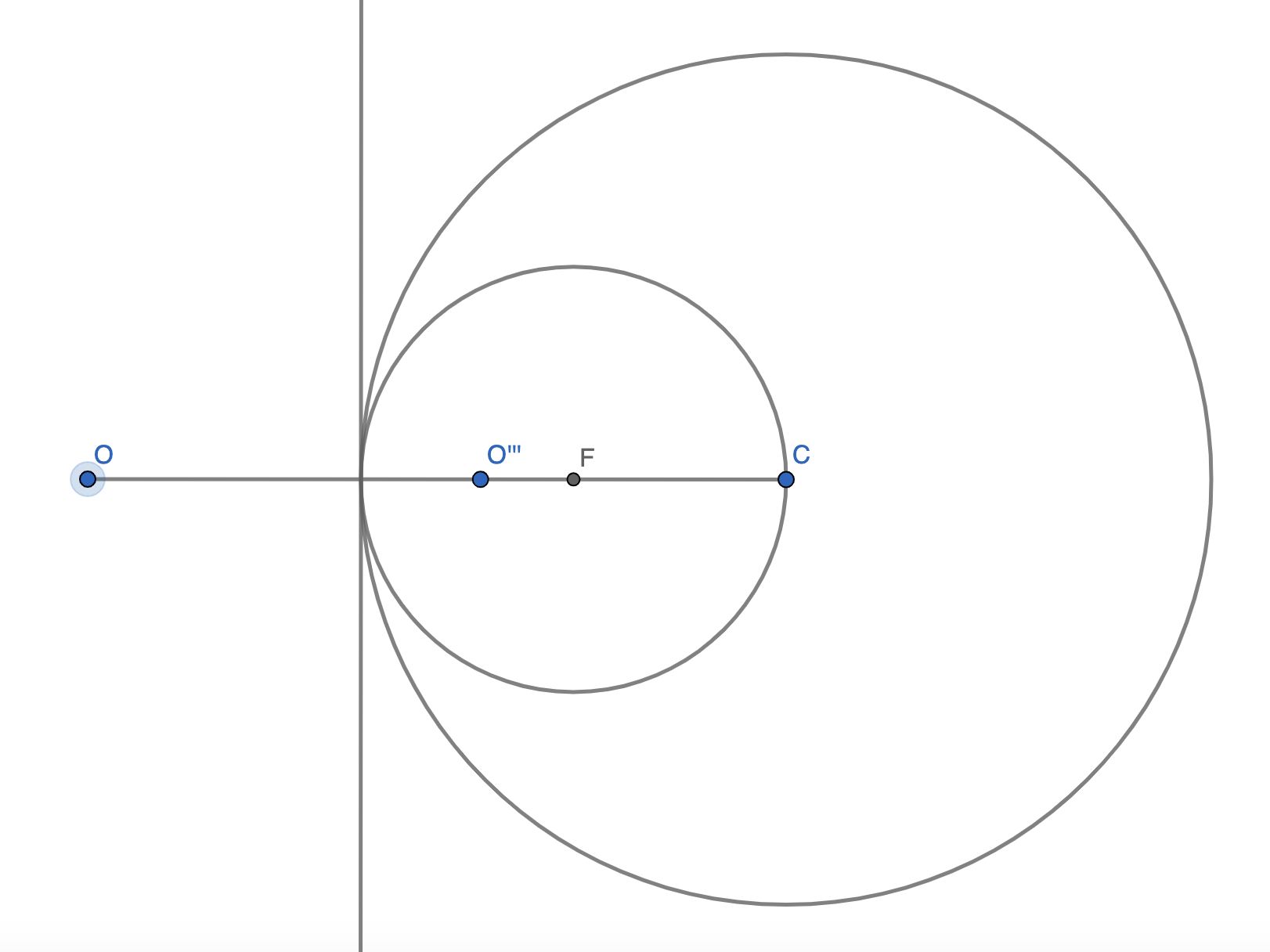
The location of the image happens to coincide exactly with the image of the point object $O$ when reflected about the circle centered at the focus $F$. (Note: Throughout this explanation, I'm assuming that $O$ is a point object on the $x$-axis.)

This relation can actually be derived quite easily from the defining equation of circle inversion: $OP \cdot OP' = r^2$ (where $O$ is the centre of the circle, $P$ is the original point, $P'$ is the reflected point and $r$ is the radius of the circle).

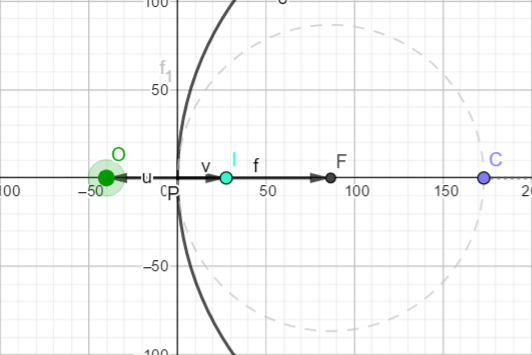$I$ coincides exactly with the reflected image of the object O about the circle centered at F.">

In the case of spherical mirrors, the original point is $O$, the image is $I$, the center of the circle is $F$, and the radius is $f$. Further, $OF = f - u$, $IF = f - v$. Plugging these values into the equation gives

$$(f - u)\cdot (f - v) = f^2$$ $$\implies f\cdot (v + u) = u\cdot v$$ $$\implies \frac{1}{u} + \frac{1}{v} = \frac{1}{f} $$

Is it merely a coincidence that reflection in spherical mirrors can be described by circular inversion about an imaginary circle about the focus, or is there a deeper reason behind this?

Answer 1

The first thing to note is that the formula given above for spherical mirrors is only a paraxial approximation, so one part of the mystery to unravel is where the approximation comes from.

The below discusses the 2D case but equally applies to 3d.

One way to get an approximate reflection O' is to create a flat planar mirror (represented by the line) that is perpendicular to the line OC and tangent to the circular mirror.

This approximation only works well when the point O is close to the mirror (or looked at another way, the circular mirror is very large).

Now lets use the circular mirror as a circle of inversion. The plan here is to invert point O through the circular mirror, then reflect the inverted point, then undo the inversion. Notice that inverting a point twice gets back to where you started, so to undo an inversion just apply the inversion again. So we are going to invert, reflect, invert. Let's do it!

1. Invert. Invert the point O through the circular mirror to point O'. Since we used the mirror as the circe of inversion, the mirror looks the same after inversion.

2. Reflect. Now we can use the same reflection approximation on the inverted point. Create a flat mirror and reflect the inverted point O' to O''. This is why the final point we come up with in the end will only be an approximation. Also notice that this inversion brings far away points close to the mirror, which is what is needed for the flat mirror approximation to be accurate.

3. Invert back. We're still in the inverted world, so we now need to invert back to the real world. Invert the reflected point O'' to O'''.

Now here is the really cool part. We can directly get to O''' from O by a single inversion through the circle that you describe above that has half the radius of the circular mirror!

In hindsight, that special circle with half the radius now makes some sense. If you invert that circle through the circular mirror, it becomes the flat plane mirror we used in the reflection approximation.

So hopefully this gives some intuition on why inversion through the half circle is a good approximation for reflection in the circular mirror when the point is close to the axis of the system.