I began boiling pasta on a power burner on our stove. After about ten minutes, I looked into the pot to see the pasta encircling the outer edge of the pot and standing vertically. Below is a picture. What makes pasta do that? Has anyone ever seen this phenomenon before?
-
$\begingroup$ When you say the pasta was 'circling the outer edge', do you mean the pieces of pasta were 'orbiting' around the rim in a particular direction? $\endgroup$– Time4TeaCommented Sep 26, 2019 at 18:19
-
1$\begingroup$ Hi! No- the noodles weren't actually moving. They were stationary along the outer edge of the pot, but I'm interested in learning how they came to be in that formation. I literally just dumped them in the pot, left for ten minutes, and then came back to this visual. $\endgroup$– Cashida DCommented Sep 26, 2019 at 20:42
-
$\begingroup$ electric or gas stove? Just wondering if convection currents might be involved $\endgroup$– pentaneCommented Sep 26, 2019 at 22:35
-
$\begingroup$ Hi - it's a gas stove. The particular "eye" that I used was the "power broiler." $\endgroup$– Cashida DCommented Sep 27, 2019 at 13:34
-
$\begingroup$ I think this is a nice illustration of Bernoulli's principle, or more exactly - dynamic pressure. When air/hot water flows to the surface- pot walls experiences additional pressure term due to flow movement upwards, due to which some pasta is aligned across walls. $\endgroup$– Agnius VasiliauskasCommented Oct 17 at 8:49
2 Answers
My best guess would be that it's due to convection flow passing through the pasta's cylindrical gap in a stable manner when upright.
Convection when the bottom is heated causes hot water (and possibly an amount of steam formation if very hot) at the bottom to flow upwards.
- Pasta's that are lying down will be pushed around all the time and never really find rest.
- Pasta's that are standing upright will not be pushed around (at least, much less) since the upwards flow can pass through the cylinder's gap.
Eventually, if any lying-down pasta's by chance happen to reach an upright state when tumbled about, then they would prefer to keep this state.
Only those that are upright along the pot's wall will be stable in this upright state. Upright pasta's in the interior will quickly be tumbled over by the lying-down pasta's that are jumping around. Therefor we see these preferred upright pasta's states mainly/only near the wall where they are stable and kept from falling over when hit by a bouncing pasta piece from the middle.
This would be my best guess on an explanation here.
I suspect the idea of Rayleigh-Bénard convection may play a role here.
When you have a layer of fluid - like water in a pot - which is hotter at the lower surface and cooler at the upper surface, the result is natural convection. The less-dense hot fluid rises, and the more dense cooler fluid circulates back down to replace it. Exactly how the fluid circulates depends on a number of parameters, mostly captured by the Rayleigh number:
$$Ra = \frac{\alpha}{\nu\kappa}g\Delta TH^3$$
When you punch in the gravitational acceleration $g$, the temperature difference between the lower and upper surfaces $\Delta T$, height of the fluid $H$, and a few physical properties of the fluid into that equation, you get a number as the answer. If that number turns out to be on the smaller end, you might not get much convection at all. As the Rayleigh number increases, you start to get an upward swell in the center of the container, with downwards motion near the edges. For even larger Rayleigh numbers, the structure of a single plume in the center will start to break down, and you'll get more dynamic and unstable behavior with smaller plumes popping up and moving around.
Of course, the Rayleigh number is designed to predict behavior in the theoretically ideal scenario, where the entirety of the upper and lower surfaces are held at constant, uniform temperatures, and you don't have any boiling or simmering going on. It's hard to say for sure, but from your picture, it looks like you were cooking your pasta in an aluminum pot, on a gas stove-top. Depending on the relative size of the pot and the burner, it's possible the heat was more localized towards the center of the pot, and the edges were a bit cooler. Even if the pot technically had a high enough Rayleigh number to generate several smaller rising plumes, most of the falling fluid would likely remain near the edges in this case.
Furthermore, if there was a hot spot in the center of the pot, most of the boiling action would be localized there as well. The rising pockets of steam would be more likely to stir up the pasta in the center, and allow it to be carried by the convective current out towards the edges, where it would tend to align with the local flow direction before settling in by the wall.
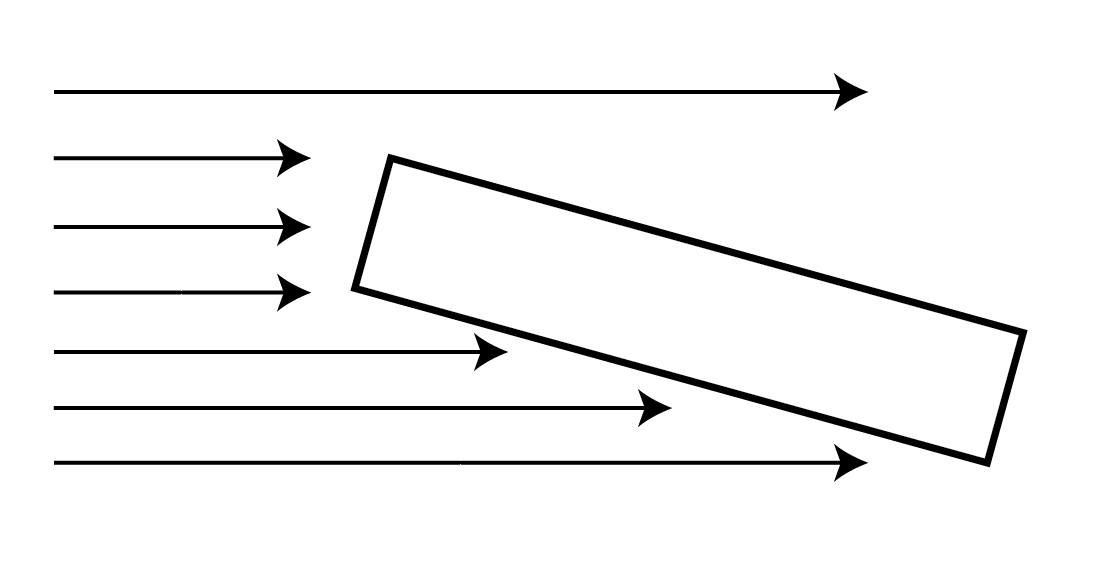
If a given noodle is not perfectly aligned with the flowing water around it, it will naturally tend to align itself due to the difference in hydrodynamic pressure. The image below depicts a pasta noodle, with water flowing past it in a uniform direction. The water will more aggressively impact the lower surface, tending to want to rotate it to be parallel with the fluid flow (note that the lengths of the arrows are not meant to represent the magnitude of the flow velocity as in a vector field, it's purely an artistic rendering meant to show where the water is hitting the pasta).
-
$\begingroup$ The Rayleigh number normally describes the whole system (in these small cases, not a huge thin atmosphere of a planet), here the whole pot. There should not be a different Rayleigh number in different parts. There can, of course, be a different Nusselt number in different parts, or more simply, different heat flux and different wall temperature in different parts. $\endgroup$ Commented Oct 17 at 7:59
-
$\begingroup$ Fair point. The way I had it worded was a bit of a misuse of the concept of the Rayleigh number, though I still believe my overall logic to be correct. I edited the answer to reword the offending text. $\endgroup$ Commented Oct 19 at 5:56