I suspect the idea of Rayleigh-Bénard convection may play a role here.
When you have a layer of fluid - like water in a pot - which is hotter at the lower surface and cooler at the upper surface, the result is natural convection. The less-dense hot fluid rises, and the more dense cooler fluid circulates back down to replace it. Exactly how the fluid circulates depends on a number of parameters, mostly captured by the Rayleigh number:
$$Ra = \frac{\alpha}{\nu\kappa}g\Delta TH^3$$
When you punch in the gravitational acceleration $g$, the temperature difference between the lower and upper surfaces $\Delta T$, height of the fluid $H$, and a few physical properties of the fluid into that equation, you get a number as the answer. If that number turns out to be on the smaller end, you might not get much convection at all. As the Rayleigh number increases, you start to get an upward swell in the center of the container, with downwards motion near the edges. For even larger Rayleigh numbers, the structure of a single plume in the center will start to break down, and you'll get more dynamic and unstable behavior with smaller plumes popping up and moving around.
Of course, the Rayleigh number is designed to predict behavior in the theoretically ideal scenario, where the entirety of the upper and lower surfaces are held at constant, uniform temperatures, and you don't have any boiling or simmering going on. It's hard to say for sure, but from your picture, it looks like you were cooking your pasta in an aluminum pot, on a gas stove-top. Depending on the relative size of the pot and the burner, it's possible the heat was more localized towards the center of the pot, and the edges were a bit cooler. Even if the center of the pot would technically havehad a high enough Rayleigh number to generate several smaller rising plumes, most of the falling fluid would likely remain near the edges in this case.
Furthermore, if there was a hot spot in the center of the pot, most of the boiling action would be localized there as well. The rising pockets of steam would be more likely to stir up the pasta in the center, and allow it to be carried by the convective current out towards the edges, where it would tend to align with the local flow direction before settling in by the wall.
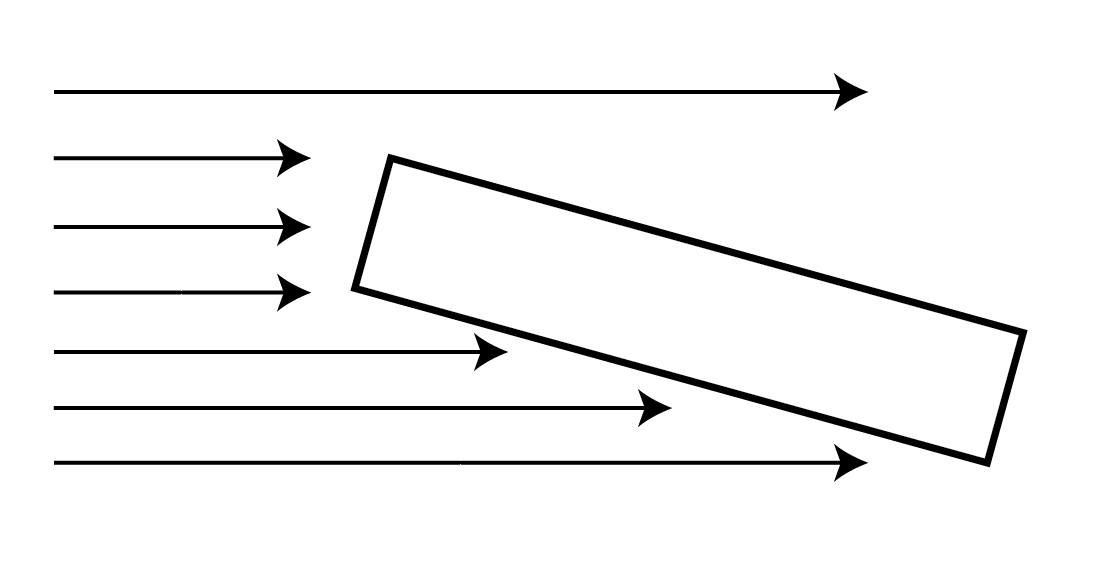
If a given noodle is not perfectly aligned with the flowing water around it, it will naturally tend to align itself due to the difference in hydrodynamic pressure. The image below depicts a pasta noodle, with water flowing past it in a uniform direction. The water will more aggressively impact the lower surface, tending to want to rotate it to be parallel with the fluid flow (note that the lengths of the arrows are not meant to represent the magnitude of the flow velocity as in a vector field, it's purely an artistic rendering meant to show where the water is hitting the pasta).