To answer your first question, we have to remind ourselves on a very important property of the plasma: if you insert a test charge into a plasma, plasma particles of the opposite charge will form a space charge cloud around that test charge cancelling its electric field such that the test charge is not "seen" by the plasma outside of this space charge cloud. The distance over which the test charge is shielded is the Debye length which depends on plasma temperature and density.
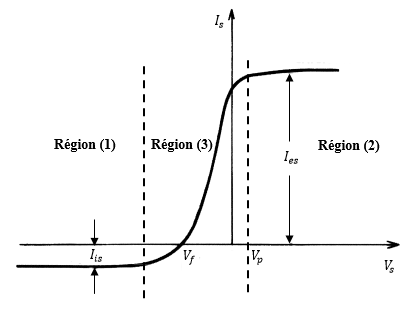
If we replace the test charge with a Langmuir probe, the exact same thing will happen: depending on plasma temperature and density, the potential of the probe will be shielded over a certain distance (Debye length) and it is not possible to attract charged particles outside this sheath. This is why the current saturates.
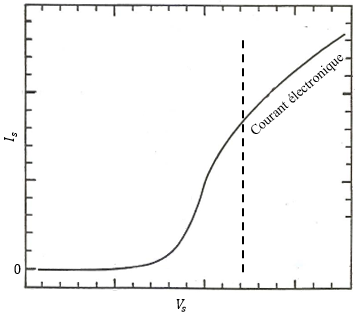
Your second question implies that such a saturation does not exist in reality (i.e. the lab). This is indeed the case, as illustrated by the characteristic you have plotted. A simple explanation for the non-saturation is the Orbital Motion Limit model. Let's consider a spherical Langmuir probe. As explained above, a sheath will be formed around the probe shielding its potential. If, however, charged particles from the plasma fly by the probe, passing it, they will be attracted by the probe's potential (or repelled). This will change their trajectory. If they are very slow, their trajectory can change such that they are collected by the probe (similar to celestial object movement, hence the name of the model). This will increase the effective probe surface. The higher the probe bias (the applied voltage), the more likely it is to "catch" passing charges. This is why the drawn current increases further with increasing bias voltage.
Note that edge effects, leading to higher electric fields, are not part of the above mentioned model. Higher (local, i.e. at the edges) electric fields, however, will simply result in an increased effective probe surface based on the same explanation.