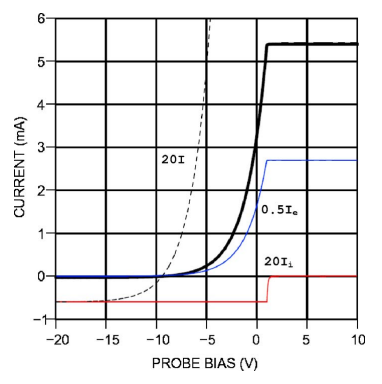
From the I-V curve of a Langmuir probe, the curvature in the ion saturation region (negative voltage) is due to the electron temperature (assuming maxwellian distribution). Similarly in Merlino's paper Understanding Langmuir probe current-voltage characteristics, the curvature in the electron saturation region (positive voltage) would be caused by the finite ion temperature.
This equation shows the ion current as a function of the probe potential. $V_B$ is the probe voltage, $V_p$ is the plasma potential and $T_i$ the ion temperature.
He also shows the contributions of the electron current and ion current on a generated I-V curve from the current equations. In his example, the ion current is a lot smaller than the electron current so the ion contribution is so small that the curvature due to the ion temperature appears as a sharp knee.
In Chen's mini course on Langmuir probe you can read "From the I-V curve, the plasma density $n$, electron temperature $KT_e$, and plasma potential $V_s$ can be determined, but not the ion temperature."
Merlino also says "As a consequence, it is impossible to use the probe to determine the ion temperature [...]".
In practice, I have seen some I-V curve with curvature at high positive voltages. If you could see the curvature instead of a sharp knee, would you be able to measure the ion temperature? I know a Ball-Pen probe can reduce the electron flux which could reveal the curvature due to the ion temperature. Is there any condition where can you measure the ion temperature from the I-V curve of a standard Langmuir probe? Does any sheath condition prevent the measurement of the ion temperature?
Sources: