I recently conducted an experiment to study the $I$-$V$ characteristics of a Debye sheath, but I can't obtain electron saturation. Why does it happen?
$\begingroup$
$\endgroup$
3
-
$\begingroup$ Would engineering SE be a better home for your question? $\endgroup$– GonencCommented Jul 1, 2015 at 7:41
-
$\begingroup$ There are a number of things which could be to blame, but without knowing more about your experimental setup it's impossible to say. What's the working gas, what kind of discharge is it, what's the geometry, is there a magnetic field, how strongly ionised is the plasma...? $\endgroup$– tok3rat0rCommented Jul 1, 2015 at 7:51
-
1$\begingroup$ In general, trying to get any useful information out of LPs based on the electron saturation current is pretty hopeless: see the lecture notes by Francis Chen here. $\endgroup$– tok3rat0rCommented Jul 1, 2015 at 7:54
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
Although this is a very old question, I think it deserves a brief answer as the non-existence of electron saturation current is a common phenomenon in Langmuir probe experiments.
A short answer would be: look at the Oribatal Motion Limit model, see for example [Piel2010]. Let's try to elaborate this very briefly a bit further.
If a charged object is inserted into a plasma, a space charge region will be formed around that object, effectively shielding its electric field. The shielding happens within a distance of the Debye length (this is how it is defined). If our charged object is a small sphere, its electric field will attract passing charged particles thereby modifying the trajectories of those (passing) particles.
If the passing particles do not have much energy, i.e. they are slow, they can be caught by the sphere (which is our Langmuir probe). This increases the effective probe area. The higher the voltage applied to the Langmuir probe (our small sphere), the more passing charges are attracted by the probe and the "saturation" current increases further. Thus, an increase in voltage leads to an increase of the effective probe area.
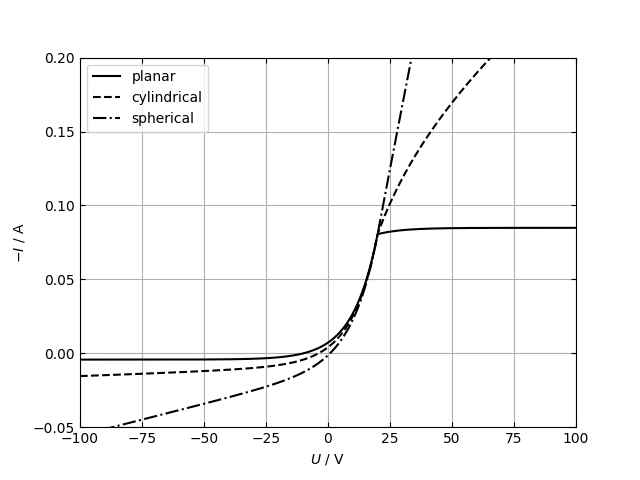
Assuming a Maxwellian electron and ion population, the current drawn by a Langmuir probe can be written as $$ I = I_e + I_i, $$ with, respectively, the electron and ion current $I_e$ and $I_i$: \begin{array} {rclcr} I_e&=&I_{e,sat} \exp\left[ -\frac{e(\phi_p-U)}{T_e} \right] & & U \le \phi_p\\ I_e&=&I_{e,sat} \left(1+\frac{e(U-\phi_p)}{T_e}\right)^\beta & \quad & U > \phi_p\\ I_i&=&I_{i,sat} \exp\left[ +\frac{e(\phi_p-U)}{T_i} \right] & & U > \phi_p\\ I_i&=&I_{i,sat} \left(1-\frac{e(U-\phi_p)}{T_e}\right)^\beta & & U \le \phi_p\\ \end{array} and the corresponding saturation currents \begin{eqnarray} I_{e,sat} &=& -enA\sqrt{ \frac{T_e}{2\pi m_e} } \\ I_{i,sat} &=& 0.61enA\sqrt{ \frac{T_e+T_i}{m_i} }. \end{eqnarray} $\phi_p$ is the plasma potential, $U$ the applied voltage, $T_e$ and $T_i$ are the electron and ion temperature, $n$ the plasma density, $A$ the probe surface, and $\beta$ takes into account the orbital motion model mentioned in the beginning.
The resulting characteristics for a hydrogen plasma with $T_e=T_i=10\,\mathrm{eV}$, $n=10^{18}\,\mathrm{m}^{-3}$, $\phi_p=30\,\mathrm{V}$ is shown in the plot. As indicated in the question, a non-saturation of the electron saturation current is clearly seen for the cylindrical or spherical probe.
[Piel2010] A. Piel: Plasma Physics: An Introduction to Laboratory, Space, and Fusion Plasmas (Springer, 2010)
