2
$\begingroup$

I understand that the solar wind follows the Parker spiral shape of the Sun's magnetic field, thus it is possible for solar wind from a point on the opposite side of the Sun to reach Earth.

I have seen it argued that solar energetic particles (SEPs) from a solar flare not visible to Earth can also reach Earth, following the Parker spiral. However, I'm not sure if this is true. These SEPs are relativistic; surely they are not deflected that much by the magnetic field?

Improve this question
$\endgroup$
9
  • 1
    $\begingroup$ SEPs during strong flares actually fill up the inner heliosphere and do not necessarily follow the nominal interplanetary magnetic field (IMF) along what is usually consistent with the Parker spiral. However, the SEPs that do follow the magnetic tend to arrive earlier than those diffusing across the field. Some of the STEREO spacecraft observations show SEP enhancements on the opposite side of the sun from the flare source location, which is not along the IMF. $\endgroup$
    – honeste_vivere
    Commented Jun 4, 2019 at 7:04
  • 1
    $\begingroup$ @honeste_vivere I'm slightly confused by your last sentence, "not along the IMF". Did you mean "along the IMF"? If SEP enhancement is detected on the opposite side of the Sun, isn't that along the IMF (spiral wrapping around)? $\endgroup$
    – binaryfunt
    Commented Jun 4, 2019 at 11:14
  • 1
    $\begingroup$ No, not in general or even necessary. So far as we can tell, there seems to be a lot of cross-field diffusion and much the inner heliosphere fills up with SEPs during strong solar flares. $\endgroup$
    – honeste_vivere
    Commented Jun 4, 2019 at 15:11
  • 1
    $\begingroup$ The solar wind does not follow the Parker spiral. The solar wind moves radially outward from the Sun. It is the magnetic field that is connected to both this plasma in the solar wind and the location on the Sun's surface it was emitted from. Since the emitted plasma stays at the same angle, but the Sun rotates, the magnetic field forms a spiral. See it as spraying a water hose and twirling around. The stream of water you spray will create a spiral, but that does not mean the water travels in a direction following the spiral, it just moves radially outwards. $\endgroup$
    – Lu Kas
    Commented Mar 22, 2021 at 11:10
  • 1
    $\begingroup$ @OutisNemo ok, cool. I wasn't aware of this. But in general you need to keep in mind that a plasma system is terribly coupled: the plasma motion drives the magnetic field, and the magnetic field drives the plasma motion. To the first order, the Parker spiral is created by the motion of the plasma, with the magnetic field 'frozen-in' it. But when you get more complicated effects where plasma structures are blocked by others, as in your reference, you'll get higher order effects. And then certainly the magnetic field, which is along the Parker spiral, is going to affect the motion of the plasma. $\endgroup$
    – Lu Kas
    Commented May 13 at 11:49

1 Answer 1

Reset to default
2
$\begingroup$

I understand that the solar wind follows the Parker spiral shape of the Sun's magnetic field, thus it is possible for solar wind from a point on the opposite side of the Sun to reach Earth.

No, not really. The solar wind flows almost radially outward from the Sun. The magnetic field is nearly frozen-in to the plasma so they can be thought to move with one another (though be careful how far you take this somewhat careless statement).

Energetic particles generated during the initial launch of coronal mass ejections (CMEs) and/or solar flares are called solar energetic particles (SEPs). In general, these have energies in the range of 10s of keV to ~1 GeV for ions and several keV to a few MeV for electrons (e.g., see this answer for literature references).

I have seen it argued that solar energetic particles (SEPs) from a solar flare not visible to Earth can also reach Earth, following the Parker spiral. However, I'm not sure if this is true.

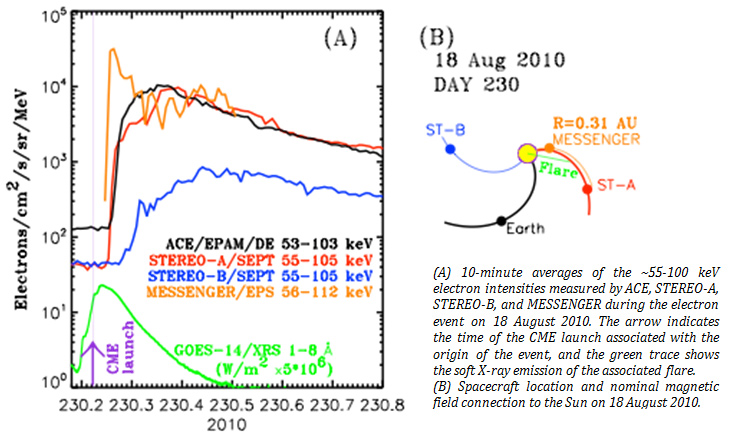
It is true that SEPs generated during a CME or solar flare that launches on a side far from that facing Earth can still send SEPs to Earth (e.g., see image below, which can be found at http://www.srl.caltech.edu/ACE/ACENews/ACENews139.html). They can even send SEPs to spacecraft on the far side of the Sun.

The problem is that the community is not certain whether this results from cross-field diffusion or if the magnetic field topology near the Sun is so complicated that it allows SEPs to stream to all sides of the Sun. This is one of the goals of the current Parker Solar Probe mission and the future SunRISE mission. That is, understanding the source and evolution of these energetic particle events is a top-level science priority for NASA and the Heliophysics community.

These SEPs are relativistic; surely they are not deflected that much by the magnetic field?

Why not? That they are relativistic actually makes it easier for them to diffuse in some ways because they cannot "turn" as quickly as the magnetic field geometry may change spatially. The gyroradius is proportional to $\gamma \ v_{\perp}$, where $\gamma$ is the Lorentz factor and $v_{\perp}$ is the particle speed orthogonal to the local magnetic field vector. So the more relativistic a particle becomes, the larger the gyroradius. The larger the gyroradius, the slower the changes in the magnetic field need to be in order for the particle to continue propagating along it.

http://www.srl.caltech.edu/ACE/ACENews/ACENews139.html

Improve this answer
$\endgroup$
1
  • $\begingroup$ That's a good explanation; but given that Solar wind flows radially, how come fast CMEs are deflected "eastward" (in the reverse Solar physics convention of east and west, i.e. to the left as seen from Earth)? In papers about the deflection of CMEs it says that this occurs due to the Parker spiral. See e.g.: shorturl.at/apPU2 "Under the effect of the Parker spiral magnetic field, a fast CME will be blocked by the background solar wind ahead and deflected to the east, whereas a slow CME will be pushed by the following background solar wind and deflected to the west." $\endgroup$
    – Outis Nemo
    Commented May 10 at 12:40

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.