Why is the cone shaped space capsule free fall through the atmosphere bottom forward the stable orientation?

Question

It seems the stable orientation of the cone shaped space capsule while free falling through the atmosphere is having the bottom point towards the translational velocity relative to the atmosphere. Can someone explain the aerodynamics of this (stabilization) mechanism?

Answer 1

We assume the following.

1) The force exerted by the air on a surface is pure pressure thus normal to the surface without friction. The pressure is an increasing function of the magnitude of the incoming air flow velocity component that is normal to the impinging surface.

2) The surface of the capsule is axially symmetric. Label the intersection of the symmetric axis and the surface (bottom) facing the incoming airflow $B$. The inward normal vector $\vec n$ of any infinitesimal surface patch either intersects the axis at point $N$ some finite distance from $B$ or $\vec n$ parallels the axis. The center of mass of the capsule $C$ locates between $B$ and $N$.

The capsule will achieve aerodynamic stability.

---

Before presenting the proof of this proposition, I give plausible toy model of this air flow pressure function. The realistic function will surely be more complicated.

However, interestingly, two and a half months after I posted this answer, I happened upon the theory of hypersonic aerodynamics that surprisingly endorsed almost fully the following derivation as the correct computation for the pressure of hypersonic (Mach 3-5) airflow on an largely axial symmetric body with blunt surface geometry. c.f. equations (11-2) and (11-3) of chapter 11 on the hypersonic aerodynamics of W. H. Mason's lecture on configuration aerodynamics. Search for "Newtonian Impact Theory" in this accompanying PPT to that chapter.

Suppose an air column of an infinitesimal cross section area $dA$ collide with a facet with its normal vector forming an angle $\theta\in\big[0,\frac\pi2\big]$ with the air flow direction vector. The air bounces off the facet completely elastically. The momentum change (all in the normal direction of the facet) per unit time is then $2\rho v^2\cos\theta dA$, where $\rho$ is the density of the air flow and $v$ the speed of it. The area upon which this momentum change occurs is $\frac{dA}{\cos\theta}$. Divide the first quantity by the second, we get the pressure $p(\theta):=2\rho v^2\cos^2\theta$. Now the early arriving particles bounce off of the surface normally and collide completely elastically with the late arriving particles and bounce back towards the surface again. By symmetry, the average particle velocity near the surface vanishes in the surface normal direction but its component tangent to the surface remains. Macroscopically, the fluid on average as a whole moves along the tangent of the surface.

Moreover, the part of the object surface that is in the "shadow" of the incoming airflow will remain untouched by the airflow and thus experience no pressure.

---

Proof:

1) 2-dimension.

Let us formulate the problem formally. Let $s\in[-s_0,s_0],\,s_0>0$ measure the distance, with sign, from the intersection of the symmetry axis with the surface. Denote the unit inward normal vector at $s$ by $\hat n(s)$. Let $\theta(s)$ be the angle from $\hat n(0)$ to $\hat n(s)$ with counterclockwise direction as the positive direction for the angle. $\theta(-s)=-\theta(s)$ by the axial symmetry. Let the angle from $\hat n(s=0)$ to the incoming airflow direction be $\theta_a$ also with counterclockwise direction as the positive direction. Place the curve $(x(s),y(s))$ in the Cartesian coordinate such that $(x(s=0)=0,y(s=0)=0)$ and the center of mass be located at $(x=0,y=y_c)$. We have $(x(-s),y(-s))=(-x(s),y(s))$. Let $p(\beta)$ be the pressure as a function of the angle $\beta$ with respect to the incoming air flow. The torque at each curve with respect to $(0,y_c)$ is $l(s)p(\theta_a-\theta(s))$ where $l(s)\hat z = \big((x(s),y(s))-(0,y_c)\big)\times \hat n(s)$.

Without loss of generality we assume $\theta_a>0$. Otherwise we can just reflect the coordinate with respect to the $y$ axis and get back the same problem because of the axial symmetry.

The total torque is, needing only to account for the surface facing the incoming airflow, \begin{align} T&:=\int_{-s_0}^{s_0}l(s)p(\theta_a-\theta(s))ds \\ &=\int_0^{s_0}l(s)\big(p(\theta_a-\theta(s))-p(\theta_a+\theta(s))\big)\,ds \end{align} as $l(-s)=-l(s)$ by the axial symmetry of the curve. Stability is achieved if $T>0$. We have $l(s)>0,\,\forall s>0$ since, by Assumption 2), the center of mass $C$ located at $(0,y_c)$ is between $N$ (at the origin of the coordinate $(0,0)$) and $B$. $p(\theta_a-\theta(s))>p(\theta_a+\theta(s))$, since $|\theta_a-\theta(s)|<\theta_a+\theta(s),\ \forall \theta_a>0,\, \theta(s)>0,\, s>0$, and the fact that $p(u)>p(v),\,\forall |u|<|v|$. Therefore $T>0$.

2) 3-dimension

The 3-dimensional case can be reduced to the 2-dimensional one above by symmetry.

(to be continued)

QED

Answer 2

If I wasn't terrible at drawing, I would make an illustration. But think of an egg falling through air. The bottom as quite flat. If it falls bottom first and starts to rotate a bit to any side, the air pressure will increase on this side and bring it back to the bottom first position, so this position is stabilized.

Now think of the egg falling again, but this time with the tip first. As soon as it starts rotating, the air pressure will increase on the tilted side and increases the rotation. This position is unstable.

Answer 3

This answer is posted in Space Exploration https://space.stackexchange.com/questions/61398/during-spacecraft-reentry-why-is-heatshield-side-down-the-most-stable-orientatio/61404#61404. Don't know the Stack Exchange etiquette for copy/pasting answers, but here it is

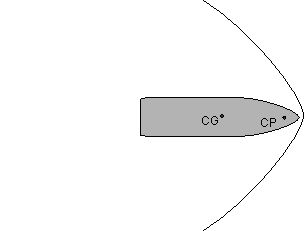
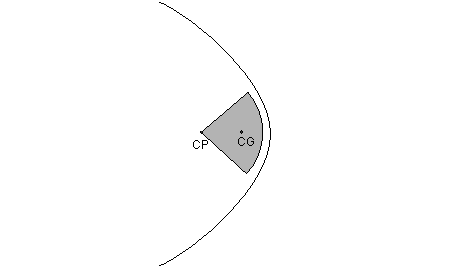
We’re accustomed to seeing things travelling pointy-end forwards (bullets, rockets, arrows, Lamborghinis) so it seems “natural” that Entry Vehicles (EV) should be most stable traveling pointy-end first as well. Not so.

For example, bullets are inherently unstable since their Center of Gravity (CG) is behind their Center of Pressure (CP). They only achieve relative static stability due their extremely high spin rate, hundreds of thousands of RPMs.

An airgun pellet (they sometimes travel supersonic) has static stability since the CG is ahead of the CP

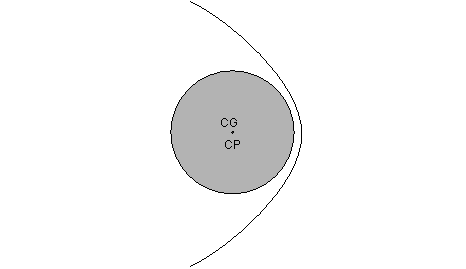
A sphere has static stability due to its symmetry

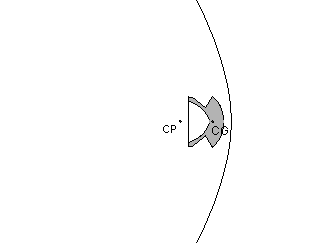
A portion of a sphere has a similar shock wave to a complete sphere. As long as the CG is ahead of the center of spherical curvature, the object is statically stable.

The static stability of a spherical section is assured if the vehicle's center of mass is upstream from the center of curvature

https://en.wikipedia.org/wiki/Atmospheric_entry#Entry_vehicle_shapes

A EV has a similar shape to the pizza pie section above, but rounded at both ends. The relationship between its CG and CP is similar to an airgun pellet.

If this EV is travelling pointy end first, the curvature generating the shock wave has a much shorter radius. This places the CG behind the CP and creates static instability, just like a bullet.

Answer 4

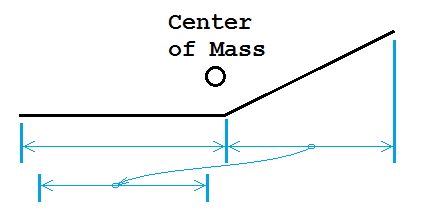
Here's my guess:

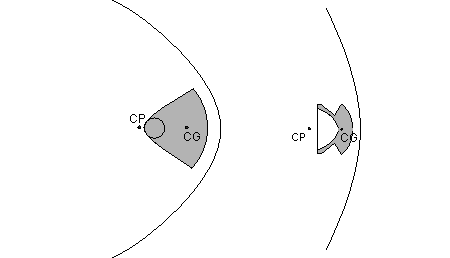
Since the heat shield is not planar, when it pitches off-center one side of the heat shield presents more area to the flow, providing a corrective torque. It depends on the center of mass being close enough to the base of the heat shield.