Can a planet have multiple significant sized moons?

Question

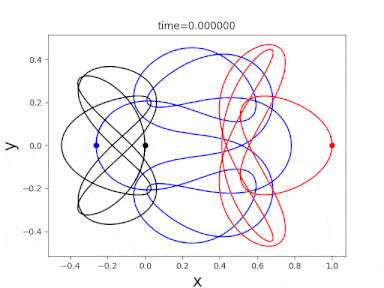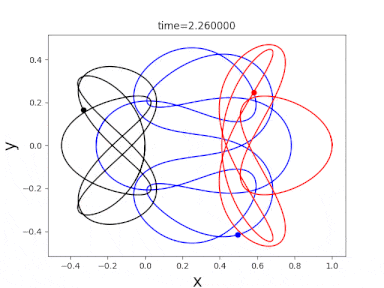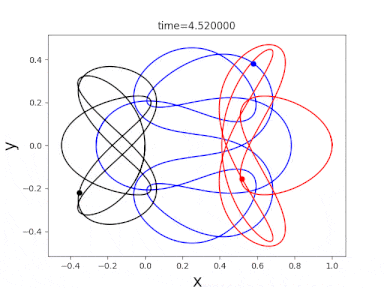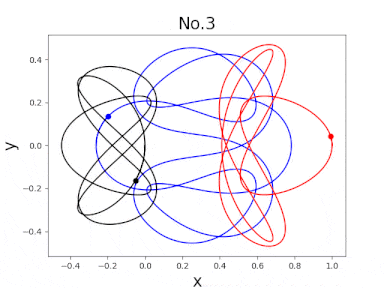
The Earth has one moon at about 1/80 of Earth's mass. Is it possible to have two moons large enough each to subtend a >30 minute disk as viewed from the surface?

I have tried with various simulators and systems fail spectacularly within a few dozen orbits, either with one colliding with the planet, or being thrown out of the system.

This question was prompted by many SF novels with covers showing several large moons in the sky.

Preliminary thoughts:

One possibility is to have the two moons co-orbiting each other and the pair orbiting the planet. As long as they are outside Roche's limit this is fairly reasonable. But it is boring. I'm seeking solutions where moons can be in line with the planet either both on one side or planet in the middle.

If we use Luna as the first moon (our present moon) then if we locate Selene (the new one) at half the distance it has to be only half the diameter and 1/8 the mass. This would make it 1/640th the mass of earth. (Because tides go up as inverse cube of the distance, but direct as mass, little Selene would cause about the same size tides as Luna)

Is it easier to make a long term stable system with large differences in mass?

E.g. Earth/Luna is about 80. If we used a similar ratio between Luna and Selene, then Selene has to be just under 1/4 Luna's diameter or about 800 km. To have the same angular diameter it has to be at 1/4 the distance, giving it an orbital period around 10 days.

However I don't know that this condition will work. So far I have been unable to find papers on how constraints on 3 body problems are derived.

Are there resonances that increase stability (negative feedback loop) rather than scramble the eggs?

I've asked this question on Physics Forums, and gotten no good reply.

---

Comment on 1 answer received so far:

Wikipedia https://en.wikipedia.org/wiki/Trojan_(celestial_body)

As a rule of thumb, the system is likely to be long-lived if m1 > 100m2 > 10,000m3 (in which m1, m2, and m3 are the masses of the star, planet, and trojan).

More formally, in a three-body system with circular orbits, the stability condition is

$27 * (m_1m_2 + m_2m_3 + m_3m_1) <(m_1 + m_2 + m_3)^2$

2. So the trojan being a mote of dust, m3→0, imposes a lower bound on

$m_1 \over m_2$ of ${25+\sqrt{621}} \over 2$

≈ 24.9599. And if the star were hyper-massive, m1→+∞, then under Newtonian gravity, the system is stable whatever the planet and trojan masses. And if

${m_1 \over m_2} = {m_2 \over m_3}$

then both must exceed 13+√168 ≈ 25.9615. However, this all assumes a three-body system; once other bodies are introduced, even if distant and small, stability of the system requires even larger ratios.

Answer 1

There is a paper on this. It does not explicitly take into account the angular diameters of the moons viewed from the planet's surface, but it argues that the earth could support up to three Luna-mass moons. With moons of lower mass it can support more. Given the number of smaller moons that would be possible, I strongly suspect you could have a smallish, nearby moon that looked larger than Luna from the ground.

Just as an example, a moon 200 miles across in geosynchronous orbit would look larger than Luna in the sky.

Answer 2

Even after three centuries of study, three-bodies are still a problem. I don't think there is yet a definite answer to your question.

I assume you don't just want the visual effect of two moon-sized objects visible in the sky, which you could get from the "Earth" in a hierarchical three-body system with an "Earth-Moon" binary orbiting a Jupiter-sized gas giant at a distance that makes the "Moon" and "Jupiter" the same size. I believe such a system passes the mass stability criterion you quote, nor is it obviously impossible based on the discussion in the Astro SE question "Do moons have moons?" and the Kollmeier & Raymond paper "Can Moons have Moons?".

You comment that two moons co-orbiting or in Lagrange points are too boring, so how about a system of three similar-sized planets orbiting each other like this

This is from "One family of 13315 stable periodic orbits of non-hierarchical unequal-mass triple systems" by Li, Li, and Liao. (Original figure here.) Watching the other planets shrink and grow dramatically in the sky would be pretty amazing.

The above authors have an earlier paper where they looked for periodic orbits for two equal (normalized) masses $m_1=m_2=1$ and a third unequal mass $m_3$. They found 565, 401, 237, 85, 35, 17, and 9 orbits for $m_3$ = 0.5, 0.75, 2, 4, 5, 8, 10, respectively. Since the Earth/Moon mass ratio is 81, the extrapolation is not promising. You may also not like that the $m_3=10$ orbits all have the moons always on opposite sides of the planet.

Of course, all these recent stable configurations are for isolated point mass systems, so you are correct to worry that might not be stable for real planetary systems with tidal effects and perturbations from other planets. It is also a bit discouraging that - as far as I can tell - no stable non-hierarchical stellar triples have yet been observed, although Li, Li, and Liao are optimistic they will be. (All observed triple star systems are "hierarchical", where there is a tightly bound binary with a third star orbiting both at a much greater distance.)

I am pessimistic that the kind of system you are looking for exists, but I don't think all hope is lost. The three-body problem is an active area of research.

Answer 3

If you make the moons the same size they can be placed simultaineously in each other's $L_4$ or $L_5$ Lagrange points, which should be stable. https://en.wikipedia.org/wiki/Lagrangian_point