Even after three centuries of study, three-bodies are still a problem. I don't think there is yet a definite answer to your question.
I assume you don't just want the visual effect of two moon-sized objects visible in the sky, which you could get from the "Earth" in a hierarchical three-body system with an "Earth-Moon" binary orbiting a Jupiter-sized gas giant at a distance that makes the "Moon" and "Jupiter" the same size. I believe such a system passes the mass stability criterion you quote, nor is it obviously impossible based on the discussion in the Astro SE question "Do moons have moons?" and the Kollmeier & Raymond paper "Can Moons have Moons?".
You comment that two moons co-orbiting or in Lagrange points are too boring, so how about a system of three similar-sized planets orbiting each other like this
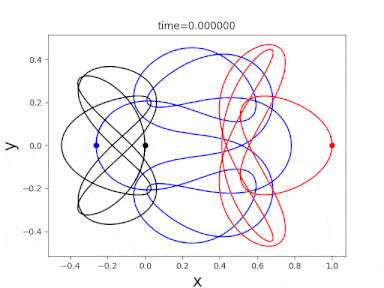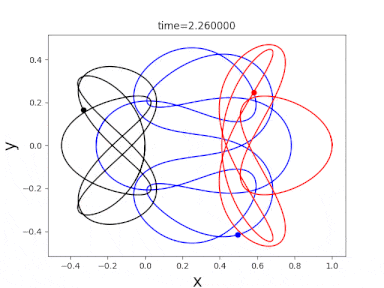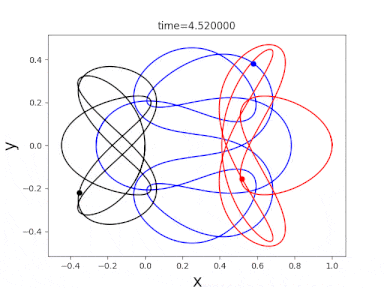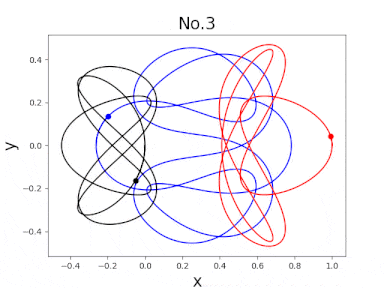
This is from "One family of 13315 stable periodic orbits of non-hierarchical unequal-mass triple systems" by Li, Li, and Liao. (Original figure here.) Watching the other planets shrink and grow dramatically in the sky would be pretty amazing.
The above authors have an earlier paper where they looked for periodic orbits for two equal (normalized) masses $m_1=m_2=1$ and a third unequal mass $m_3$. They found 565, 401, 237, 85, 35, 17, and 9 orbits for $m_3$ = 0.5, 0.75, 2, 4, 5, 8, 10, respectively. Since the Earth/Moon mass ratio is 81, the extrapolation is not promising. You may also not like that the $m_3=10$ orbits all have the moons always on opposite sides of the planet.
Of course, all these recent stable configurations are for isolated point mass systems, so you are correct to worry that might not be stable for real planetary systems with tidal effects and perturbations from other planets. It is also a bit discouraging that - as far as I can tell - no stable non-hierarchical stellar triples have yet been observed, although Li, Li, and Liao are optimistic they will be. (All observed triple star systems are "hierarchical", where there is a tightly bound binary with a third star orbiting both at a much greater distance.)
I am pessimistic that the kind of system you are looking for exists, but I don't think all hope is lost. The three-body problem is an active area of research.
