0
$\begingroup$

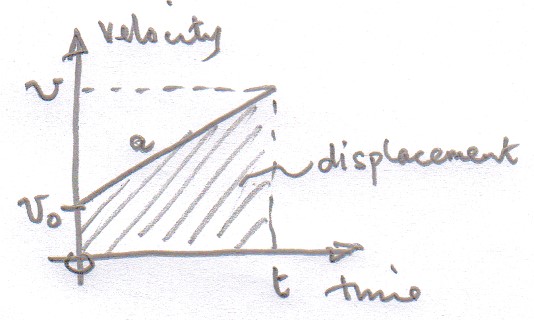
From the linear velocity function in Equation 2-11, the average velocity over any time interval (say, from $t=0$ to a later time $t$) is the average of the velocity at the beginning of the interval ($=v_0$) and the velocity at the end of the interval ($=v$). For the interval from $t=0$ to the later time $t$ then, the average velocity is $$v_{\mathrm{avg}} =\frac{1}{2} (v_0+v)$$

I don't understand why average velocity can be found just be taking the mean of two velocities. Can someone please explain?

Improve this question
$\endgroup$
5
  • $\begingroup$ Check out this link hyperphysics.phy-astr.gsu.edu/hbase/vel2.html. $\endgroup$
    – K_inverse
    Commented Sep 13, 2018 at 3:09
  • 1
    $\begingroup$ @sup: Average velocity is the total displacement divided by the total time. $\endgroup$
    – Vincent Thacker
    Commented Sep 13, 2018 at 3:30
  • $\begingroup$ Hi, welcome to Physics SE! Please do not post images of texts you want to quote, but type it out instead so it is readable for all users and so that it can be indexed by search engines. For formulae, use MathJax instead. I've edited it here. $\endgroup$
    – user191954
    Commented Sep 13, 2018 at 4:46
  • 1
    $\begingroup$ Your book says that the average velocity could be found by taking the mean of the initial and the final velocity in the case where the velocity is a linear function of time and that is correct. Your intuition seems in the right place that, in general, velocity should not be simply the mean of the initial and final velocity but should be the mean over all the velocities, i.e. $\frac{1}{T}\int_{0}^{T}v dt$. But when velocity is a linear function of time, this general formula reduces to the formula provided in your book. Try to work this out explicitly yourself! :-) $\endgroup$
    – user87745
    Commented Sep 13, 2018 at 5:00
  • $\begingroup$ sup, this equation applies ONLY to an object undergoing a constant acceleration (including zero acceleration). $\endgroup$
    – David White
    Commented Sep 13, 2018 at 5:46

1 Answer 1

Reset to default
1
$\begingroup$

I don't understand why average velocity can be found just be taking the mean of 2 velocities.

In general your concern is correct but in the example you have given it is stated

For the linear velocity function

which implies that $v = v_0 +at$ where $a$ is the constant acceleration.

The velocity against time graph looks like this

enter image description here

with the total displacement $s$ in a time $t$ being the area under the graph $s= \frac 12 (v_0+v)t$.

Thus the average velocity $v_{\rm avg}=\frac{\text{total displacement}}{\rm time}=\frac s t =\frac 12 (v_0+v)$

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.