6
$\begingroup$

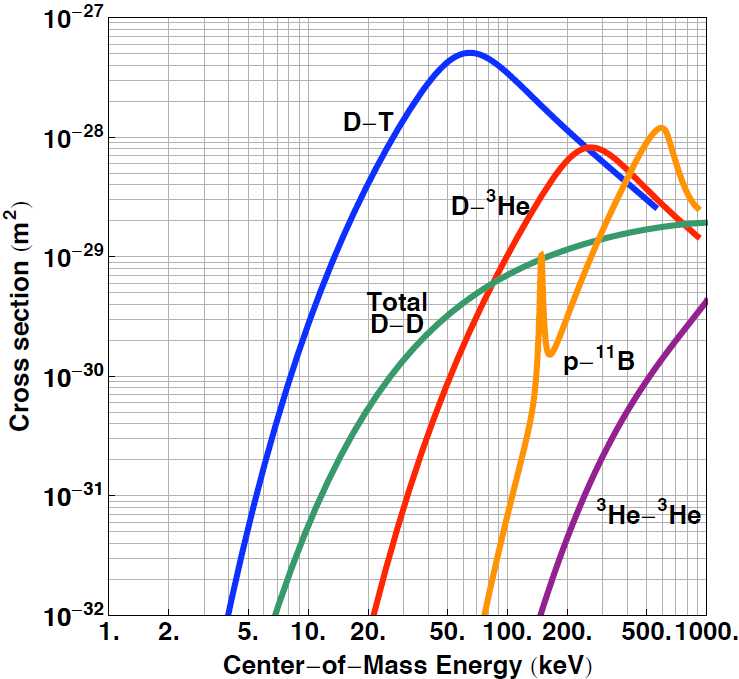
The title sums everything up. The fusion cross section increases up to a certain temperature due to collisions and the increased probability of tunneling through the coulomb barrier. But why does the cross section drop after a certain number? Do the collisions become less frequent or is there any other reason behind this? My friend told me, once the particles start moving extremely fast, they don't have enough time to fuse (for tunneling to make them fuse). Is this right? If it is, what is the minimum time needed for the particles to fuse?

enter image description here

  • Why do the cross sections drop after a certain temperature?
Improve this question
$\endgroup$

2 Answers 2

Reset to default
4
$\begingroup$

If I am not mistaken, this is due to the fact that the fusion cross section is basically a product of the cross section of the two nuclei which have to be close enough to interact with each other. The relevant distance here is the de Broglie wavelength and that decreases with increasing energy, a higher energy nuclei thus corresponds to a smaller target.

The other factor is the tunneling probability through the Coulomb barrier and that increases with increasing energy.

The resulting fusion cross section is therefore the product of two competing function, hence the peak, which is also referred to as Gamow peak. You can also look at that process using the wave functions of the two nuclei which exhibit, in my understanding, a wave-wave resonance at the Gamow peak.

In my understanding, the process @dmckee is referring to, i.e. the splitting of the nuclei into smaller pieces, happens at much higher energies. For a quark-gluon plasma (smashing the protons and neutrons into its constituents, the quarks) you need energies on the order of $100\,\mathrm{MeV}$.

Improve this answer
$\endgroup$
2
  • $\begingroup$ But why is the distance or the radius taken to approximately the de broglie wavelength? why does a particles radius depend on its energy? I don't get it $\endgroup$
    – Chandrahas
    Commented Mar 31, 2017 at 16:53
  • $\begingroup$ @Chandrahas tunneling through the Coulomb barrier roughly occurs when the particles are within one de Broglie wavelength of each other; apart from that, it is slightly confusing here because we are mixing particle and wave picture: you said a particles radius depends on its energy (particle picture), whereas I said its wavelength depends on its energy (wave picture). It is not always possible to easily translate between wave and particle picture , see wikipedia-article $\endgroup$
    – Alf
    Commented Apr 3, 2017 at 6:04
3
$\begingroup$

When the incident nuclei have too much energy left after crossing the Coloumb barrier they are increasingly likely to split one or more of the participants into smaller pieces than to neatly snuggle together. Or even to messily snuggle into a highly excited state that can then drop down toward the ground state by various photon emissions.

For a crude, human-accessible model imagine throwing two lumps of clay together. Too softly and they won't stick. Too hard and the violence of the collision throws bits off in all directions. Just right and you get an intact, combined mass.

Improve this answer
$\endgroup$
1
  • $\begingroup$ So, if the particles have too much energy, the product (Helium nucleus), will just break down again? Into deuterium and tritium? And for the not enough time thing, is it true? $\endgroup$
    – Chandrahas
    Commented Mar 13, 2017 at 6:52

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.