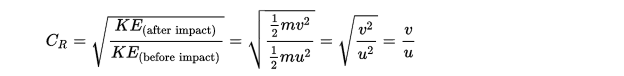3
$\begingroup$

According to Wikipedia,

The coefficient of restitution (COR) is a measure of the "restitution" of a collision between two objects: how much of the kinetic energy remains for the objects to rebound from one another vs. how much is lost as heat, or work done deforming the objects.

and the formula is

So If COR is the part of energy available after collision, why they just can't divide final KE and initial KE. Why the square root came?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

Note first in your simplification in calculating the COR you cancelled the mass before and after the collision, but in general this may not be the case. If there are two masses colliding after the collision one or more may break apart, with additional masses carrying off some of the energy. Or one mass or part of that mass may stick to the other.

But I believe your question rather focuses specifically on why the square root. In either case, with or without the COR you have a dimensionless result.

But the rudimentary definition from the wiki article itself says:

"The coefficient, e is defined as the ratio of relative speeds after and before an impact, taken along the line of the impact"

And in order to to have a ratio of relative speeds with regards to dimension, the square root must be applied.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Addendum: We want the speed ratio because of signs. Negative means a bounce, positive means a collision where one object went through the other, transferring part of its momentum. The signs can be swapped depending on convention. $\endgroup$
    – Kotlopou
    Commented Jul 11, 2020 at 22:01

Not the answer you're looking for? Browse other questions tagged or ask your own question.