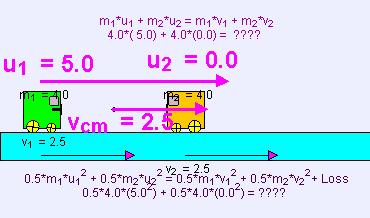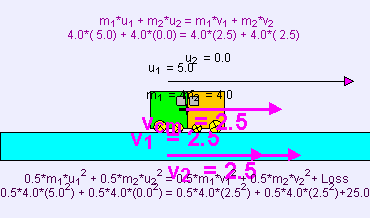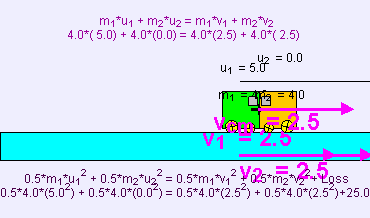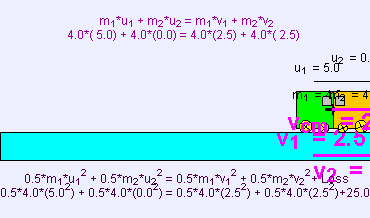I was reading the article on Co-efficient of restitution on Wikipedia. I came across this line:
A perfectly inelastic collision has a coefficient of $0$, but a $0$ value does not have to be perfectly inelastic.
I understand the first part that a perfectly inelastic collision has a value of zero for the COR (Co-efficient of restitution) but I don't get the second part that a value of $0$ doesn't have to perfectly inelastic ? Anyone examples to support that statement ?
Suppose that a body of mass m moving at speed u collides with a stationary body of mass $2m$ and sticks to it, so the coefficient of restitution ($e$) is zero. Applying conservation of momentum to determine the speed of the composite body after the collision, you'll find that 1/3 of the initial kinetic energy has been retained. If we define a perfectly inelastic collision as one in which all the kinetic energy is lost, then the collision is not perfectly inelastic even though $e=0$. This, presumably, is what the Wiki claim means.
I have issues with the supposed definition just given. If we look at the collision in the frame of reference of the centre of mass of the system, then both bodies come to rest when they stick together, and all the kinetic energy is lost. So in this frame, we'd have to say that the collision is perfectly inelastic. So, with the supposed definition given above, whether or not a collision is perfectly inelastic would depend both on the collision itself and on the frame of reference in which we view it. Unsatisfactory!
We therefore have a choice: either don't use the term 'perfectly inelastic', or use it to mean that in the centre of mass frame all kinetic energy is lost – which happens if and only if $e=0$. In the first case the Wiki claim is meaningless, in the second case it is false.
There is no similar issue with perfectly elastic collisions. If kinetic energy is conserved in one frame of reference, it is conserved in all.
To understand this reason, let's define Coefficient Of Restitution (COR) for collision of two particles/bodies as follows $$COR=\frac{\text{Relative velocity after collision}}{\text{Relative velocity before collision}}=\frac{\text{Velocity of Separation}}{\text{Velocity of Approach}}$$ $$\boxed{\color{blue}{\text{Two bodies stick together after collision}}}\iff\boxed{\color{blue}{COR=0}}$$
From above it can concluded that COR becomes zero only when two colliding particles/bodies stick together (or get merged). And when two colliding bodies stick together (i.e. $COR=0$) then the loss of kinetic energy may or may not be maximum. But for the maximum loss of kinetic energy (i.e. condition of perfectly inelastic collision) the colliding bodies necessarily stick together i.e. $COR=0$
Now, a perfectly inelastic collision means there is maximum loss of kinetic energy keeping linear momentum constant (which is always conserved) this is possible only when colliding particles/bodies stick together thus their velocity of separation becomes zero ($COR=0$).
Therefore a perfectly inelastic collision means $COR=0$ but vice versa is not true i.e. $COR=0$ doesn't necessarily mean a perfectly inelastic collision.
If you go with the usual definition of "perfectly inelastic" as "it reduces kinetic energy by the maximum possible amount", $e = 0$ does necessarily imply that the collision is perfectly inelastic. This is because, by Pythagoras' theorem, the KE of a body as a whole is the sum of the KEs of the body as a result of each of the perpendicular components of its velocity:
$$v^2 = v_x^2 + v_y^2 + v_z^2$$
$$\frac{1}{2}mv^2 = \frac{1}{2}mv_x^2 + \frac{1}{2}mv_y^2 + \frac{1}{2}mv_z^2$$
A force as a result of a collision ideally only acts on one axis, leaving the velocity in the other two axes unaffected; thus the maximum KE reduction is the amount by which the KE falls when the component of velocity in the "collision axis" is reduced to 0 (i.e., $e = 0$, and the bodies stick together). Conversely, a perfectly inelastic collision can only occur if the component of velocity in the "collision axis" is reduced to 0, and so $e = 0$ is a necessary consequence of a collision being perfectly inelastic.
("Collision axis" refers to the common normal axis at the contact point between the surface of the two bodies, through which repulsive forces associated with the collision act.)
